I have read in many books about induced current in a coil (Faraday's law), and also the motional emf across a moving conductor in a magnetic field. But somewhere I read about induced electric field due to a time varying magnetic field. And I think that Induction of electric field is the fundamental phenomenon, and induced emf and current are the results of it I am just a novice in physics. Could someone explain me how these phenomenon (Induction of emf and Induction of electric field) are related to each other?
1
-
1$\begingroup$ hyperphysics.phy-astr.gsu.edu/hbase/magnetic/emfchb.html you might need to scroll through the pages, but look for duplicates on this site such as physics.stackexchange.com/questions/98463/… $\endgroup$– user140606Commented Jan 28, 2017 at 1:22
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
2
You are right that a changing magnetic field creates (induces) an electric field, this is an actual law of nature. Now if you put a conductor where the magnetic field is changing, you will get a current due to the produced electric field.
But in the case of the moving conductor moving through a magnetic field the reason is different. The reason for the produced current is Lorentz force, the electrons in the conductor are pushed by Lorentz force and hence you get the current. Notice in this case, even if the conductor is moving through the magnetic field the magnetic field is NOT changing so electric field is not produced, the reason must therefore be the Lorentz force.
Whenever you get confused just check whether the magnetic field is changing or not. If the magnetic field is changing then the reason for the current must be an induced electric field, if it is not changing the reason must be Lorentz force.
-
$\begingroup$ So is high school physics wrong when they teach you that when a conductor moves through a magentic field an emf is induced which is known as Faraday's Law. $\endgroup$ Commented Jan 28, 2017 at 4:21
-
$\begingroup$ But if we were to take into account special relativity where you are in the reference frame of the magnet that is moving, the magnet is stationary and the wire is moving towards it. So why isn't the Lorentz force responsible? Isn't this perspective valid too? $\endgroup$ Commented May 28, 2021 at 18:30
$\begingroup$
$\endgroup$
Talking of "induced" fields is, again, yet more bad terminology and language that conveys a misleading notion - here, an impression of a kind of "causality" of one field upon the other - that is not part of our generally-used physical model.
What it means is this: In any case where that the magnetic field is changing in time, there must also be present at the same time an electric field proportional to the rate of change of magnetic field. It violates Maxwell's equations to have a situation with a changing magnetic field only and no associated electric field.
The reason for this is that the electric and magnetic field are really one single mathematical entity, and the Maxwell's equations describe how that single entity changes. That's why you talk of an "electromagnetic field". Situations where you have, say, a dancing magnet, produce an electromagnetic field that has both an electric and a magnetic component, while if the magnet is stationary, it has only the magnetic component. This is most naturally expressed in the fully-relativistic formulation using the vector four-potential $^{(4)}\mathbf{A}$ (usually called by its components, $A^\mu$), which elegantly and seamlessly integrates the two fields. This is the truly "fundamental" entity.
answered Jul 21, 2019 at 7:34
$\begingroup$
$\endgroup$
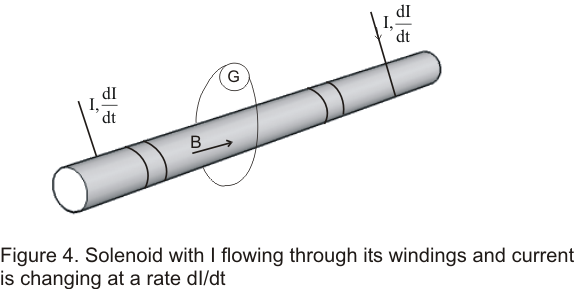
Current I through the solenoid sets up a magnetic field B along its axis and a magnetic flux Φ passes through the surface bounded by the loop.
Since charges are at rest (v=0) so magnetic forces Fm=q(v X B) cannot set the charges to motion.Hence induced current in the loop appears because of the presence of an electric field E in the loop.It is this electric field E which is responsible for the induced emf and hence for the current flowing in a fixed loop placed in a magnetic field varying with time.
using faraday's law
From equation (10) we see that line integral of electric field induced by varying magnetic field differs from zero.This means we can not define a electrostatic potential corresponding to this field.
Hence this electric field produced by changing electric field is non-electrostatic and non-conservative in nature.We call such a field as induced electric field
