If we are rotating a stone tied to a string . Then on it both centripetal and centrifugal force are acting .
Centripetal force is acting towards the centre and centrifugal is acting radially outwards and both are equal in magnitude .
Hence net force acting radially zero.
Then how the stone can perform circular motion .
There is no such thing as centrifugal force on an object. It is just an effect of an object tending to maintain its velocity in a certain direction. In the case of the uniform circular motion The centripetal force changes the direction of the velocity of the object without affecting the magnitude of the velocity. Consider the following image
We can see that if the centripetal force is removed in this case the string breaks then the object continues moving in a straight path tangent to the previous circular trajectory. When in circular motion the centripetal force provided by the string is changing the direction of the velocity of the object. If say the velocity of an object increases the centripetal force must also increase in this case the string stretches more to provide more greater force towards the center. That is Hookes law $F=kx$ to increase the force the length $x$ of the string must increase. Thus the fact that the object has an inertia, it applies a "force" on the string, In other words it's resisting change in motion(Newtons first law), since the string is trying to change the direction of the object for it go in a circular trajectory.
The centrifugal force is applied on the string itself not the object that is undergoing the circular motion, and the string applies the force on an object towards the center, That is Newton's third law, 2 pair from forces which act on "different objects" so you can't just add those forces and cancel them.
This image shows really well what is happening to an object when it starts going in a circular motion when previously going in a straight path
As you can see the blue dot is free to move. And when the car enters a circular motion the blue dot remains a straight path trajectory due to it having inertia. The blue dot is stopped from going into a straight path by the wall of the car, In other words the centripetal force is provided by the wall of the car.
In polar coordinates (in the stationary, outside system), which is the best choice of coordinate system for circular motion investigation, we have the newton's second law like this (obtained by differentiating a general motion twice):
$$\mathbf{F}=m(-r\dot\theta^2 \mathbf{\hat{r}}+\ddot r\mathbf{\hat r}+2\dot r\dot \theta \boldsymbol{\hat \theta}+r\ddot \theta\boldsymbol{\hat \theta})$$
So It is no longer like $\mathbf{F}=m(\ddot x \mathbf{\hat x}+\ddot y \mathbf{\hat y}+\ddot z \mathbf{\hat z})$.
Now. If $r$ is not changing, and $\dot\theta$ (angular velocity) is constant, we conclude $\dot r=\ddot \theta=0$. So we can simplify the general case and it becomes this:
$$\mathbf{F}=-mr\dot\theta^2 \mathbf{\hat{r}}$$
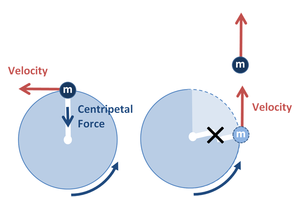
You can see that there is only one force (called centripetal force) acting on the moving particle. Which is radially inward.
But what about centrifugal one??
There is no force like centrifugal force. But! if you go to the system of coordinates of the moving particle, which means you are the moving particle. Then, because your system is not performing a uniform linear motion, there must be a fictitious force on your system. Since the only force on your system was the centripetal one we just calculated, the centrifugal one must be minus that force, which now becomes radially outward.
That our choice of system of coordinates which can totally change the math.
