What will happen if a conductor is introduced instead of a dielectric medium in parallel plate capacitor?

Question

If a conductor like copper is placed between two plates of a parallel plate conductor,neither touching any of them, what will happen to the capacitance of the capacitor?

Answer 1

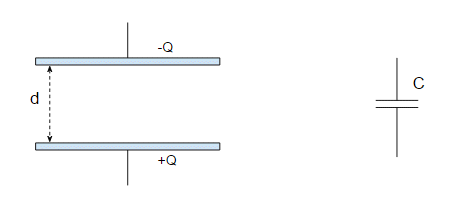
Suppose you have a parallel plate capacitor:

So we have a capacitance:

$$ C = \frac{\varepsilon_0 A}{d} $$

and a charge $Q$ on the plates given by:

$$ Q = CV $$

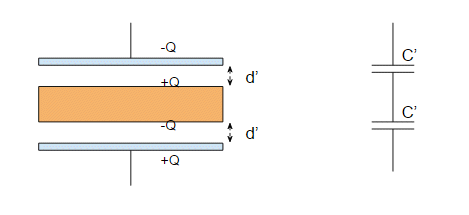
Suppose we now insert a sheet of copper in between the plates as you describe:

The electrons in the copper plate are free to move, so they flow towards the positive plate and you end up with two capacitors with an increased capacitance:

$$ C' = \frac{\varepsilon_0 A}{d'} $$

The combined capacitance is obtained by using the equation for two capacitors in series:

$$ \frac{1}{C_{tot}} = \frac{1}{C_1} +\frac{1}{C_2} $$

So in this case the new capacitance is:

$$ C_{tot} = \frac{\varepsilon_0 A}{2d'} $$

And since the copper sheet has a thickness greater than zero $2d' \lt d$ and therefore when you increase the copper sheet the capacitance increases.