Why are light rays able to cross each other?

Question

See the image first:

Why are light rays able to cross each other? Air isn't able to.

Answer 1

Note: this answer was in response to the original question:

My question is that Why the light rays able to cross each other weather water waves and air could not cross each other

Other waves pass through each other just as with light. This is easy to test. Place four people at the corner of a large room. Have two of them, at adjacent corners talk to the person at a diagonal corner. Use a cone such as a cheerleader might use to somewhat channel the sound. You may be a bit distracted by the other voice but you will clearly hear the voice from the opposite corner.

Here's a standard demo in a high school science class. Have two students hold each end of a moderately stretched slinky resting on a smooth floor. Have each student give the slinky a sharp snap to their right. Since the students are facing each other, the pulses will be opposite one another as they travel toward opposite ends. When the two pulses meet in the middle the slinky will appear relatively straight but only for an instant. The two pulses will continue to travel past one another as if they never had met.

Waves of the same kind traveling through one another maintain their original identity after the encounter. This is a basic property of waves, you can read about it in any introductory Physics text.

Answer 2

I believe it's because you are thinking of the light as particles (little solid balls) that it seems a bit odd that "they can pass through each other." I think thinking in terms of (quantum) fields gets rid of this. As someone mentioned, photons are bosons and so there is no Pauli exclusion principle that applies to them. That is to say, they can be at the same place at the same time....so the fact that they can "pass through" each other seems less odd, knowing that.

Said more simply, the information of the photons spreads out, and there is nothing to prohibit this information from allowing the photons to cross the same point in space at the same time. This is easier to see if you know math and look at the equations explaining photons.

*Note: We are neglecting any interactions between photons in this sort of explanation.

Answer 3

You seem to be looking for a more mathematically oriented answer, so let's try that. You cannot prove that light rays are able to cross each other because that is false: it only works in the approximation of very dim light. If you were to take your source of light and increase the frequency or amplitude of the electric field, you'd observe a non-linear behaviour: rays would very clearly influence each other as the pass through each other.

The key aspect of the superposition principle is linearity, that is, the fact that Maxwell's equations are linear. If you consider electromagnetic radiation in a certain material, it is well-known that for intense enough radiation the polarisation becomes non-linear, and you enter the realm of nonlinear optics. Here, the polarisation $\boldsymbol P$ becomes a (non-linear) function of $\boldsymbol E$, and therefore the wave equation becomes $$ \left(\nabla\times\nabla\times+\frac{n^2}{c^2}\frac{\partial^2}{\partial t^2}\right)\boldsymbol E=\frac{1}{c^2}\frac{\partial^2}{\partial t^2}\boldsymbol P(\boldsymbol E) $$ which is a non-linear equation for $\boldsymbol E$. For example, a very strong source of light (travelling through the air) the Kerr effect or other non-linear effects kick in. This is due to quadratic (and higher) terms in the polarisation tensor $\boldsymbol P\sim\chi_1\boldsymbol E+\chi_2\boldsymbol E\otimes\boldsymbol E+\cdots$.

The polarisation of realistic materials is always non-linear, and therefore light rays always influence each other. Only in the limit $\chi_2E^2\ll \chi_1E$ the wave equation becomes linear; in other words, only in the limit of very weak radiation are light rays oblivious to other light rays.

Even in vacuum you can observe non-linear behaviour. The Maxwell Lagrangian, corrected by quantum-mechanical effects, becomes $$ \mathcal L=\frac12(\boldsymbol E^2-\boldsymbol B^2)+\frac{2\alpha}{45m^4}\left[(\boldsymbol E^2-\boldsymbol B^2)^2+7(\boldsymbol E\cdot\boldsymbol B)^2\right] $$ where $\alpha\sim 1/137$ is the fine-structure constant, and $m$ is the mass of the electron. As $\mathcal L$ is non-linear in $\boldsymbol E,\boldsymbol B$, the propagation of electromangetic waves is no longer linear, and the superposition principle ceases to hold. Only when you can neglect the non-linear terms the superposition principle becomes valid.

In a nutshell, your question is based on a false premise. Light rays seem to be able to cross each other and continue their path unaffected, but this is because you are not using sensitive enough instruments (e.g., your eyes). If you were to measure the effect of light rays on each other with a very good piece of equipment, you'd observe that they do affect each other, both in a material and in vacuum.

Answer 4

Why are the light rays able to cross each other

The underlying level of nature is quantum mechanical. Light is an emergent phenomenon from the quantum mechanical level of photons, where an enormous number of photons of energy $h\nu$ build up the classical electromagnetic wave which is light.

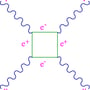
Photon–photon interactions are very very rare at energies below twice the mass of an electron . The quantum mechanical feynman diagram of two photons interacting, from which the probability of interaction can be calculated:

has four electromagnetic vertices, i.e. (1/137)^1/2 for the amplitude, and when squared as it multiplies the integral for the probability, the number becomes miniscule, so photon photon interactions are very rare. As the answer by @AccidentalFourierTransform states, using classical electromagnetic waves, some interaction can happen, but it would need very good instrumentation to see it.

One can see interference between two light beams, but interference is not interaction, it comes from the superposition of two beams collective wavefunctions, which when detected show the interference pattern from the way the photons' wave functions build up the macroscopic light beam. Superposition is not interaction, so the beams can cross and continue on their way, if a detector is not introduced in the overlap.(Note that anyway, to see interference patterns one should have coherent monochromatic beams).

For high energy photons other channels open with higher probability, but that is another story.

Answer 5

There is no force which could act between two photons so they cannot* interact with each other to cause interference.

*At least for the most part, it is possible for them to cause interference via decay to other particles.

Answer 6

Because the light consists of photons. And photons are Bosons (not like for example electrons, which are Fermions. Bosons and Fermions obey different laws (search for Fermi-Dirac statistics and Bose-Einstein statistic on Wikipedia for more info). In short and mundaine words: Fermions can not be in the same place at the same time, but Bosons can. This is why light rays go through each other.

Answer 7

A small addition to other answers. Light is both a wave and a particle, called Wave-particle Duality. Basically, It acts like both a Wave and a Particle. Its very complicated to get into, and I cant properly describe when it acts like what in all situations, but there are a few that are relevant here. First off, Air is a particle, or rather, many many particles. As such, Like you described, they can not cross eachother. What we have found though, is that Light does not act like a particle in that aspect, It acts like a wave. Peter Shor's comment links to this image showing this in water, which shows how waves act when they cross over each other. This is called Interference. When 2 waves cross, they interfere with eachother, changing the total size of the wave. 2 waves can stack on each other, becoming twice as tall, or they meet each others low points, and flatten out. They could do both, and everything in between, but they will not stop moving because of this meeting. The Interference link above to the Wiki page has some good animations of it in action.

Light will act in the same way. and this is most easily shown by the famous Double Slit experiment. There are numerous youtube videos animating this, such as this one, which shows that light, when passing through 2 slits, will hit a wall with the exact same pattern as waves of water would in the same situation, and this pattern is called the Interference pattern. It also shows off the Wave-Particle Duality, because when you actually monitor the light to determine how it passes through the slits, it acts like a particle, but that is a whole other question.