-1
$\begingroup$

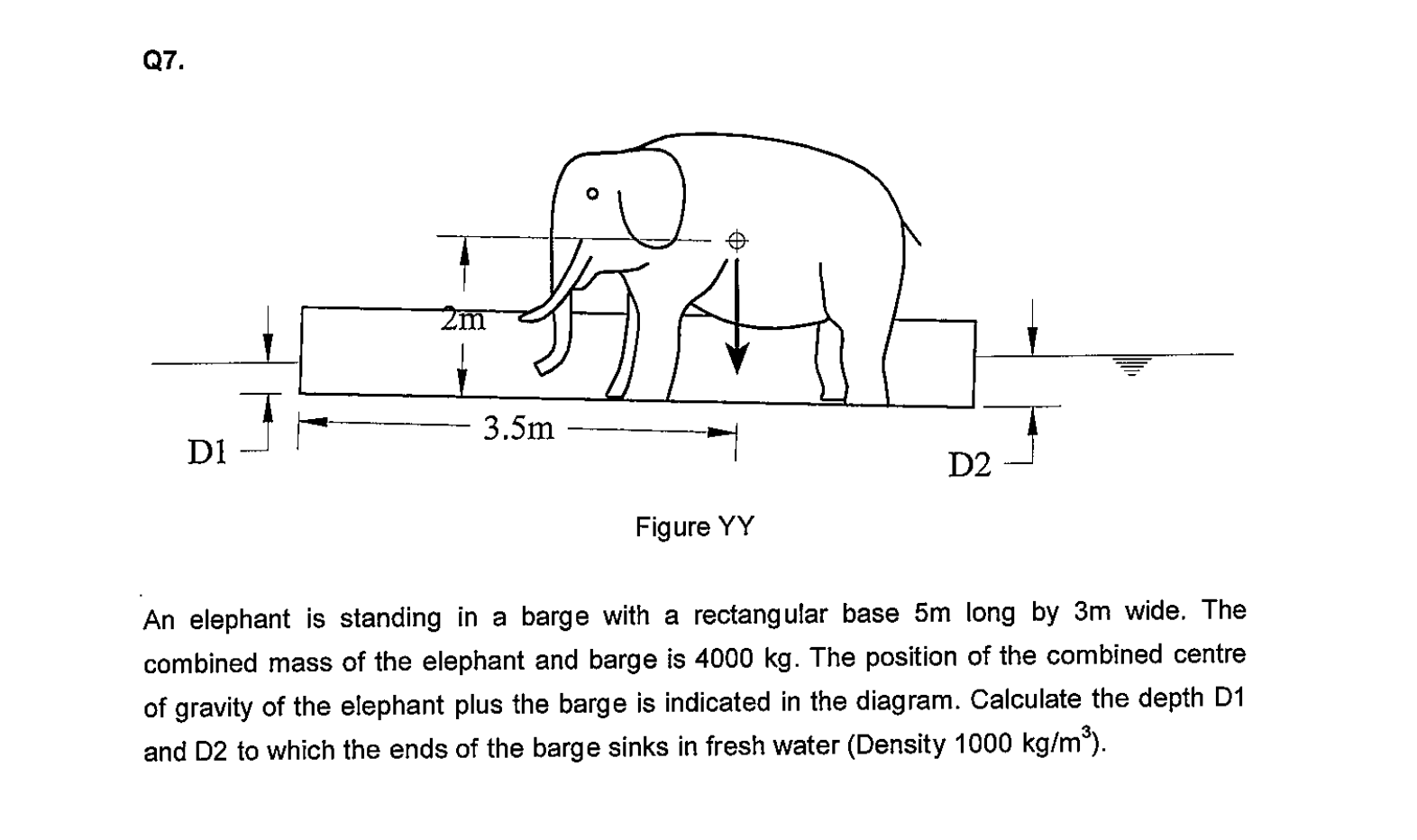
Question

I am having difficulty solving the above question, taken from a past paper. I have tried to take moments about a point O on the surface that is collinear with the bottom of the raft. If the angle of this line with the surface is $\theta$, then the moments due to the force on the bottom of the raft is: $$ M_O = cos\theta \int ^{l+5}_{l} \rho g x^2 (3dx) $$ Where $l$ is the length from O to the bottom left corner.

Now I know that the forces on the sides of the raft must contribute to the moments however I don't know how to find the position of centre of pressure of these to use to use in a moment equilibrium equation. Also I wonder if there exists an easier, more elegant solution?

EDIT:

For finding the position of the centroid of the trapezoid and equating it to the position of the centre of gravity, I got the following answer: Using the centroid of a trapezoid formula, x corresponding to the submerged length of the left side and y that of the right side: $$ \frac{L}{3} \frac{2x+y}{x+y} = L - 3.5 $$ This equation (for $L=5$) has no solutions where x and y are both positive, have I gone wrong somewhere?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

Find the area and the centroid of the submerged trapezoid. The trapezoid has two unknown parameters related to its angle and depth in the water. The two equations to determine them are that the trapezoid's area times its width times the density of water must equal the mass of elephant and barge, and the centroid of the trapezoid must be at the same horizontal position as the center of gravity of elephant plus barge.

Improve this answer
$\endgroup$
4
  • $\begingroup$ I have edited my answer, am having trouble with the solution $\endgroup$
    – user120568
    Commented Jan 10, 2017 at 16:54
  • $\begingroup$ apologies, I forgot to tag you in the previous comment $\endgroup$
    – user120568
    Commented Jan 10, 2017 at 23:31
  • $\begingroup$ Well, the hint here is to make sure you get the geometry right. Making a sketch or two for yourself would probably help. Make sure you decide on one coordinate system to represent things in, perhaps for an exaggerated case with a significant angle of the plank. From what you added to your question, it looks like you neglected the slight angle of the plank either on the left- or the right-hand side. Also, it should be clear that the height of the center of gravity (the $2\,\mbox{m}$ in your problem) matters. $\endgroup$
    – Pirx
    Commented Jan 10, 2017 at 23:37
  • $\begingroup$ Thank you, I did take x and y as being perpendicular to the bottom of the barge, thus not the vertical depth of the side, however I am struggling to see how to include the COG position without using moments in the approach. Should the centre of pressure be in line directly with the COG or the vertical projection of the COG onto the raft floor? $\endgroup$
    – user120568
    Commented Jan 10, 2017 at 23:43

Not the answer you're looking for? Browse other questions tagged or ask your own question.