1
$\begingroup$

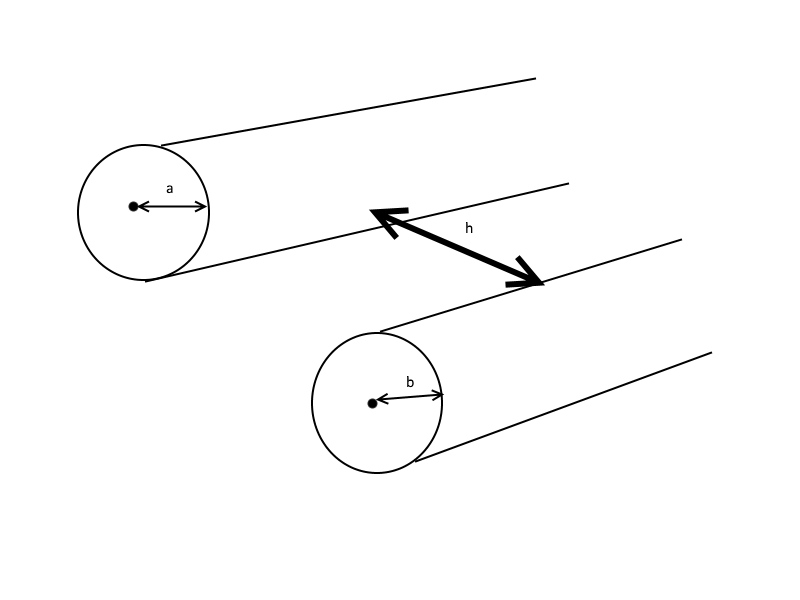
Let's say that I've got two wires, one with radius $a$ and the other with radius $b$, $h$ apart, and I want to calculate the self inductance per unit lenght of this system, which is defined as the "proportionality constant" between the current $I$ and the magnetic flux through the system. The current in the wires is flowing in opposite directions.

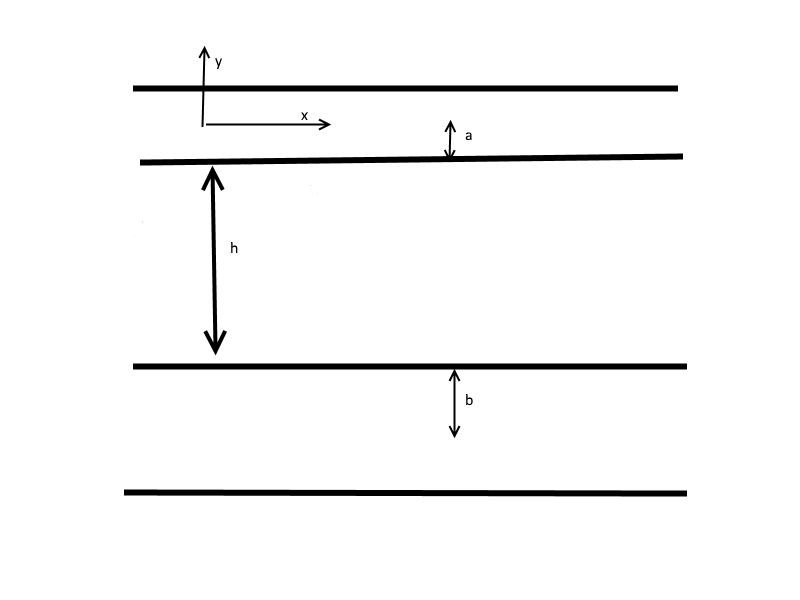
I tried to calculate the magnetic flux to derive the inductance, but got the wrong result... the magnetic flux should be given by: $\phi_m= \int \textbf{B}\space d\textbf{S}$ , $\textbf{B}=\textbf{B}_a+\textbf{B}_b$, those are the magnetic field produced by the two wires... Then I just have to integrate $\textbf{B}_a$ from $a$ to $a+h+2b$, the integral over the first, "a" wire should be zero and the analogous integral for the "b" wire...

So : $\phi_m = L \int_a ^{a+h+2b} \frac{\mu_0 I}{2 \pi y}dy+L\int_b ^{b+h+2a} \frac{\mu_0 I}{2 \pi y}dy$, where L denotes some arbitrary lenght.

enter image description here enter image description here

I know that this is wrong, but dont know why , I have read some similar questions on this board and know that it's got something to do with the "flux linking" concept, I just can't see how... Can someone please demostrate this principle on my example.

Improve this question
$\endgroup$
8
  • $\begingroup$ There are several parts of your question that are not quite clear: Are you talking about self-inductance or mutual inductance? If self-inductance, then the currents should probably be flowing in opposite directions. Also, you're defining 'L' to be a length but traditionally the symbol 'L' is reserved for inductance. $\endgroup$
    – user93237
    Commented Dec 30, 2016 at 22:44
  • $\begingroup$ Sorry... I'm talking about self inductance, and yes the current is flowing in opposite directions. $\endgroup$
    – Luka8281
    Commented Dec 30, 2016 at 23:03
  • $\begingroup$ Just to encapsualte the question here, because it could be different issues, why do you know it is wrong? e.g. I'm trying to understand if we need to get into relativity for this one. $\endgroup$
    – JMLCarter
    Commented Dec 31, 2016 at 0:19
  • $\begingroup$ Still not sure what's going on here. Since the wires have non-zero cross-sections (and the current is flowing uniformly throughout the cross-sectional area of each wire?), I would suggest instead calculating the energy in the magnetic field (proportional to $B^2$) and then using the fact that that's equal to $LI^2$, where $L$ here is the inductance. Calculating the B-field everywhere and then squaring it and integrating it over all space to get total energy may be non-trivial, but I think that that is just the nature of the problem once you start considering non-infinitesimal wire radii. $\endgroup$
    – user93237
    Commented Dec 31, 2016 at 4:44
  • $\begingroup$ They calculate the self inductance using the "recipe" you described, using the magnetic energy, but I would like to calculate this using the direct integration of the magnetic field... My question is very similar to : physics.stackexchange.com/questions/11799/… $\endgroup$
    – Luka8281
    Commented Dec 31, 2016 at 10:13

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.