How fast do you need to travel to go 35 light-years in 2 (apparent) years?

Question

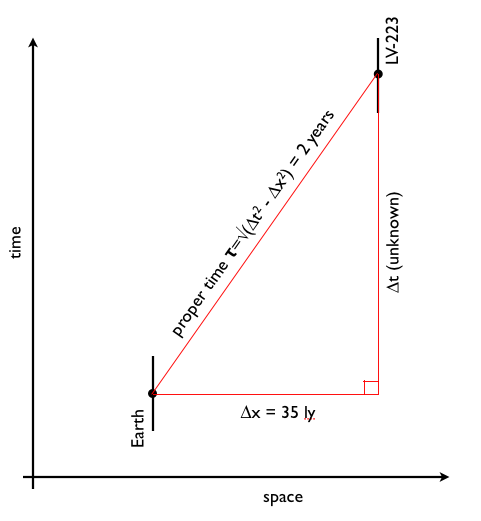
Time slows the faster you go, so perhaps going 35 light-years in "2 years" is actually do-able, but how fast would you actually be going? If

$$t' = \frac{t}{\sqrt{1-\frac{v^2}{c^2}}}\\ $$

If we say $t' = 2 \text{ years}$, and $x = 35\text{ light-years}$ (and $t = x/v$), it's fairly basic to get to

$$\left(\frac{x}{t'}\right)^2 = {v^2\left(1-\frac{v^2}{c^2}\right)} = v^2-\frac{v^4}{c^2}$$

However, time has worn on my math to such a degree that I'm now miffed. I can't figure out how to get an equation in terms of $v$.

Answer 1

A nice easy-to-remember way to do this calculation is to remember that the proper time (i.e. the time you'd experience on the journey) is calculated like Pythagoras' theorem, only with a minus sign instead of a plus sign. You can picture it like this:

A slight caveat is that the units must be such that $c=1$, but since we're using years and light years, this is indeed the case.

So we have that $\tau = \sqrt{\Delta t^2 - 35^2} = 2$, and solving this for $\Delta t$ gives $\Delta t^2 = 35^2 + 2^2$ or $\Delta t \approx 35.057$, which is the time the journey takes in Earth's reference frame. The speed in this reference frame can be calculated in the usual way, by doing $\Delta x / \Delta t$, which in this case gives us $0.99837c$.

Answer 2

With the correct formula noted by Rahul; it's much simpler.

$$t'=t\sqrt{1-\frac{v^2}{c^2}}=\frac{x}{v}\sqrt{1-\frac{v^2}{c^2}}\\ \frac{t'}{x} = \sqrt{\frac{1}{v^2}\left(1-\frac{v^2}{c^2}\right)} = \sqrt{\frac{1}{v^2}-\frac{1}{c^2}}\\ \frac{1}{v^2} = \left(\frac{t'}{x}\right)^2+\frac{1}{c^2}\\ v = \left(\left(\frac{t'}{x}\right)^2+\frac{1}{c^2}\right)^{-\frac{1}{2}}$$

Plugging in numbers, we get 0.998 371c.

Answer 3

For future reference, if you really wanted to solve the original (incorrect) equation $$u^2 = v^2 - v^4/c^2,$$ where I've replaced $x/t'$ with $u$ for convenience, you could let $w = v^2$ and get a quadratic equation in $w$, $$-\frac1{c^2} w^2 + w - u^2 = 0,$$ to which you can apply the quadratic formula $$w = \frac{-1\pm\sqrt{1^2 - 4u^2/c^2}}{-2/c^2}$$ and then take square roots to get back the solution(s) for $v$. It doesn't work with the numbers you have, though; with the incorrect formula, perceived time increases with speed, and you want to find the speed that decreases the time, so there are no real solutions.