7
$\begingroup$

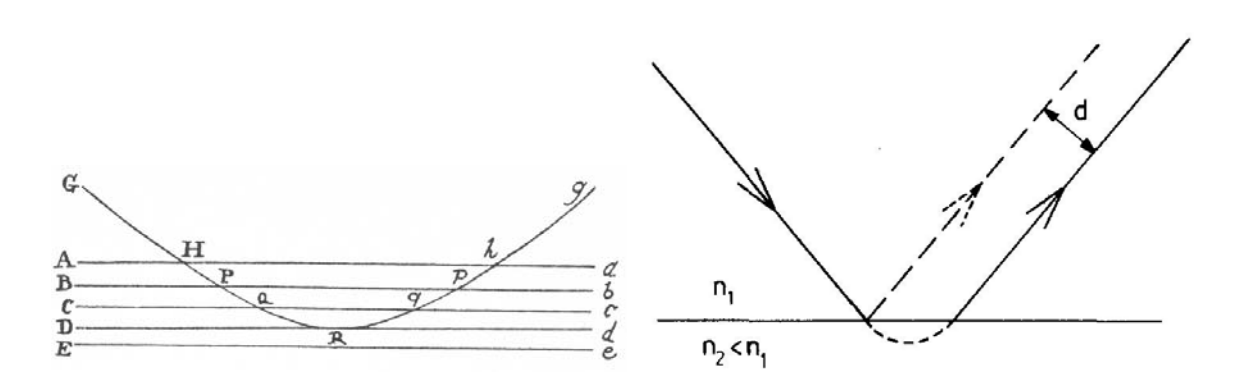
I remember reading a really elegant paper many years ago about the Fresnel reflection of a narrow Gaussian beam from a dielectric interface. The paper showed mathematically that since reflection from a dielectric slab is actually a bulk effect from all of the oscillating polarizations within the volume, the outgoing Gaussian beam follows an axis with does not intersect the incoming axis at the surface. Instead they intersect at a point which is a significant distance inside the volume of the dielectric, probably of order $\lambda / \epsilon$ (just a guess) where $\epsilon$ is the dielectric constant.

I can't find that paper right now, but my question is different. Is there any distortion of the profile of the beam? Since the reflected wave from each layer will produce an increasing lateral displacement, when they are added up would a circular-profile incident beam be reflected with an elliptical profile?

I am going to try to generate a drawing for this, but I think the question is sufficiently clear for an optics answer.

Improve this question
$\endgroup$
5
  • $\begingroup$ How is this different from reflection from a metal mirror? Light makes electrons just inside the metal oscillate. The oscillating electrons radiate a reflected beam. The illuminated spot on the mirror/dielectric interface is an ellipse if the beam is at an angle. But the reflected beam has a circular cross section. $\endgroup$
    – mmesser314
    Commented Oct 24, 2016 at 1:55
  • $\begingroup$ @mmesser314 actually what you say is almost but not exactly correct. The effect is very small in Fresnel reflection and even smaller reflecting from the electron plasma, but the reflection in both cases is a bulk effect, and does not happen exactly at the surface. Think about it - why is 10 Å of metal almost transparent - you need a few hundred angstroms to get a good reflection. Likewise 100 Å thick 'slab' of dielectric doesn't give you much either. Reflection is a bulk phenomenon, and to do the math correctly you need to integrate throughout the volume. 'Surface equations' are approximate. $\endgroup$
    – uhoh
    Commented Oct 24, 2016 at 2:00
  • $\begingroup$ @mmesser314 the key to my question is in this sentence: "Since the reflected wave from each layer will produce an increasing lateral displacement, when they are added up would a circular-profile incident beam be reflected with an elliptical profile?" $\endgroup$
    – uhoh
    Commented Oct 24, 2016 at 2:09
  • $\begingroup$ Transparent optical components are often coated with antireflection coatings. These are dielectrics typically half or a quarter wave thick. They are modelled by assuming reflection at the surface. The idea is to arrange it so all the back reflections from all the interfaces between layers add up to 0. We never considered it as a bulk effect. So I would have to say it happens so close to the surface as makes no difference. Especially when the beam is much much more than a wavelength across. But maybe that is an engineering view. $\endgroup$
    – mmesser314
    Commented Oct 24, 2016 at 2:09
  • $\begingroup$ @mmesser314 OK your opinion is noted. Let's give this question a day or two for some mathematical answers to kick in and see where it goes. I didn't ask if it makes a big difference, I asked if it happens. $\endgroup$
    – uhoh
    Commented Oct 24, 2016 at 2:11

1 Answer 1

Reset to default
6
$\begingroup$

I believe you are talking aboug the Goos-Hänchen shift which is described in this paper.

From there, the following diagram:

enter image description here

That link also gives a detailed mathematical description. The original paper (referenced from the above) is

F. Goos and H. (Lindberg-)Hänchen, Ein neuer und fundamentaler Versuch zur Totalreflexion, Ann. Phys. 1, 333 (1947), http://puhep1.princeton.edu/~mcdonald/examples/optics/goos_ap_1_333_47.pdf
Neumessung des Strahlversetzungseffektes bei Totalreflexion, Ann. Phys. 5, 251 (1949),
http://puhep1.princeton.edu/~mcdonald/examples/optics/goos_ap_5_251_49.pdf

Unfortunately, both those links point to a private (Princeton) server - and judging by the title, they are in German which may or may not be a problem for you. The original link gives a pretty detailed mathematical treatment.

So yes - you are right; there is some evidence of a shift. But since the same shift applies to every aspect of the entire beam, I don't think the profile would be distorted.

Improve this answer
$\endgroup$
6
  • $\begingroup$ OK so I think it is more than some evidence, I think it can be shown mathematically from Maxwell's equations, but I am in a pinch because I can't do serious reading for another half-day or so. I did find this though arxiv.org/abs/1210.8236 Next I need to figure out if what I remember was total internal reflection or just Fresnel reflection (which is what I'm really remembering). Thanks for this!! I'll do the work and let you know soon. $\endgroup$
    – uhoh
    Commented Oct 24, 2016 at 5:08
  • $\begingroup$ OK, off to the library today (finally)... $\endgroup$
    – uhoh
    Commented Oct 27, 2016 at 2:59
  • 1
    $\begingroup$ Let us know what you find out! $\endgroup$
    – Floris
    Commented Oct 27, 2016 at 4:29
  • $\begingroup$ I've re-remembered both this question's existence and what I was talking about. I'm pretty sure it's Fresnel reflection not total internal reflection. I've also linked to my new answer here. I'm now inspired to once again go look for the paper I've remembered and get to the bottom of this. Stay tuned... (looking forward to the future Optics and Photonics SE site!) $\endgroup$
    – uhoh
    Commented Jun 21, 2022 at 22:53
  • 1
    $\begingroup$ @uhoh wow - quite the blast from the past. Ping me again when you have it! $\endgroup$
    – Floris
    Commented Jun 21, 2022 at 23:02

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.