Magnetostatics or dynamics?

Question

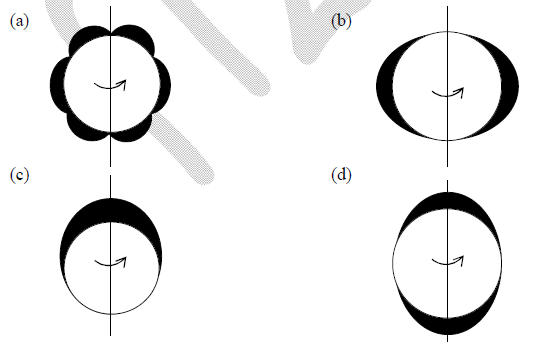
A spherical conductor, carrying a total charge $Q$, spins uniformly and very rapidly about an axis coinciding with one of its diameters. In the diagrams given below, the equilibrium charge density on its surface is represented by the thickness of the shaded region. Which of these diagrams is correct?

I am not sure if quasistatic approximation of magnetic field applies here since it is mentioned that the sphere is spinning very rapidly. Conductor is mentioned i suppose to say in other words that the charges will reside on surface no matter what.

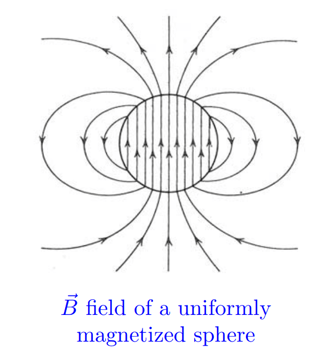
And i know that magnetic field inside a spinning spherical shell is uniform and points in the direction of rotation vector ${\omega}$ and outside, it is akin to that of a dipole situated at the origin.

The electrostatics part is unclear to me since the charges in one hemisphere cannot interact through electric field with the charges in the other hemisphere since electric field has to pass through the conductor but inside, the conductor it has to be zero.

I am not sure how exactly to use all these facts or just simply say that the centrifugal force is huge due to the high rotation speed and hence charges are just pushed away from the axis in which case the answer without any effort is option b??

---

EDIT

I may have made a mistake in assuming electric field inside the conductor to be zero since that is only true for a conductor in electrostatic equilibrium.

Answer 1

This is indeed magnetostatics. The question is about an equilibrium charge distribution. The charges are moving, and this produces a current, but that current and the charge distribution are constant. When each electron moves along the sphere, it is replaced by another one moving into its old position from behind. This is the sense in which charges can be moving without the charge distribution changing.

Also currently there are other answers that seem to think centrifugal forces on the electrons or the electrons' inertia plays a role. It does not - this effect will be negligible compared to magnetostatic forces. When you spin an iron rod, do you make voltages at either end from electrons moving outward? No.

As you point out, a uniformly charged spinning sphere creates a constant magnetic field inside the sphere (upward pointing if charge is positive). Then outside of the sphere it makes a perfect dipole field. I think, despite the different caption, this picture validly describes the magnetic field in this situation (I got this picture from here)

If you're a charge between the equator and the poles (above the equator), you'll see a magnetic field somewhere between vertical and outward. Whether it's upward or outward will depend on whether you're on the inside of the charged shell or the outside, but the conclusion won't matter. Your velocity is into the screen/page (assuming positive charges), and your $v\times\mathbf{B}$ force is somewhere between downward and outward. Either way you feel a force pushing you toward the equator from the $\mathbf{B}$ field. So I think (B) is the correct answer.

Answer 2

Well its certainly not (a) or (c), because there's no reason for electrons to bunch up like that when they still repel each other (assuming no quantum effects, here). So that leaves (d) or (b). When the sphere first spins up, it takes less energy to assume configuration (d) than (b)--so I think there's a brief time it takes on configuration (d). However, after its been spinning at the nominal rate for a while, the electrons are free to absorb more energy and do other things, if they so please. Note that a free electron orbiting in a magnetic field does not change its orbital parameters (except to radiate energy--but that doesn't apply to a classical analysis with uniform charge). So the only things that guide them in the steady state are their repulsion from each other and the centripetal 'force' from their mass. So they will slightly move toward configuration (b).

EDIT for more clarity:

(d) is initially preferred because it takes energy to accelerate an electron, even if it has no mass, because you are creating a magnetic field in the process. There certainly is a magnetic field because of Mach's principle; only if the universe were spinning along with the sphere would there not be a magnetic field. We assume that in the steady state there is no current, because this isn't a superconductor.

Answer 3

I have a different view, this is all due to centrifugal force. As we know the a particle at equator moves faster than at the poles. So to explain the charge distribution in the sphere during rotation, they will tend to move outwards and at equator there is more possibilty to get outwards (as it is moving faster it has a greater momentum and hence more chances of getting out) rather than poles. So it sums up to the answer as option b. And as someone has said earlier, there's no chance of answer as a and c as the charge wont bunch up like that.