How is the parallax angle actually measured?

Question

I understand that parallax is used to measure distances to stars. But how is the parallax angle actually measured?

In the parallax diagram we have two similar triangles, but we don't know any values other than the distance from one side of the orbit to the other, 2 A.U. Do we know the distance between the background stars? Surely we can't know that information without doing more parallax measurements?

The only explanation I have found is that the telescope knows how many pixels relate to a certain angle, but I want to know how it is measured without this.

Answer 1

It is purely a measurement of angle - essentially how many pixels the star moved and how many arcseconds/pixel the camera+telescope is measured to have.

Previously the stars were measured one at a time with a transit telescope so the angle was directly from the encoder on the declination axis (think vertical) and a clock for the right ascension (direction the stars rotate past a fixed point as the Earth turns).

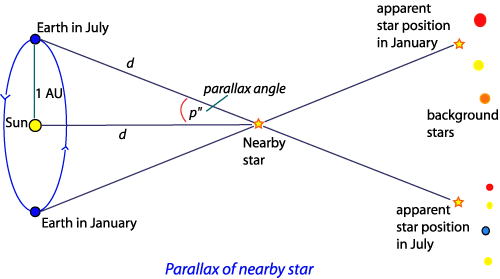
If you have telescope with a very well calibrated angle scale (pixels/arcsec). And you assume the most distance stars are fixed then you can measure how the foreground star appears to move relative to the same background stars in measurements 6months apart. You know the satellite has moved 2au around the sun and you can measure the angle difference to the star in arcsec, from the pixel movement relative to the background in the 2 images. You have then distance to the star in parsecs.

The actual technique used by Hipparcos (and I assume Gaia but I don't know the mission) is interested. The satellite has two telescopes at a fixed angle able to measure pairs of stars approximately that angle apart. As it rotates it sees lots of pairs of stars and records the relative angle. For stars close enough to measure those angles will change through the orbit.

It then performs the "mother of all simultaneous equations" (in the words of the project scientist) to work out which stars moved and which stars are fixed. It also has to solve for the constant angle between the two telescopes because this couldn't be measured well enough on the ground. The measurements are so precise they have to take into account the bending of light by Jupiter as well as the sun.

As an aside, an error during the launch meant that the final booster stage didn't separate - leaving it in the wrong orbit and with several tons of scrap metal stuck to it. The extra mass smoothed the motion of the telescope (reducing the effect of solar wind, micrometeorites etc) and led to more accurate results. A suggestion that they deliberately add several tons of scrap metal to the successor was rejected.

Answer 2

I don't know how it's actually done, but if I had to do it from Earth, I would use a telescope and a mirrors mounted on a gimbel with very accurate angle scales. (Measuring angles to an arc second isn't that difficult.) One star is viewed directly. One is viewed by mirror. Superimpose the two images.

But your baseline is the orbital diameter of the earth.

So on March 3 you measure the angle between suspected nearby star Alpha Centauri and compare to some dimmer stars (probably far away). You also measure angles between the dimmer ones.

Now September 3, when the Earth is on the opposite side of the sun, you redo all those measures. Some of the probably distant stars don't move relative to each other. This increases your confidence that they are distant. Your nearby star will shift exactly as your finger does against the background when you close one eye or the other.

So it's 6 months between blinks. This takes meticulous record keeping.