2
$\begingroup$

Observe this case

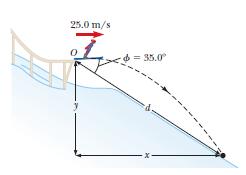
enter image description hereasdas

The goal is to maximize $d$ by increasing the angle of the initial velocity. Since we know that the range is maximum for $\theta=45^\circ$ I would reason that the jumping ramp has to be elevated for $\theta=10^\circ$ in order for $\theta+\phi=10^\circ+35^\circ=45^\circ$. However this is not the case, since the ideal angle of ramp elevation is found to be $\theta=27.5^\circ.$ Why is this?

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ The $45^\circ$ maximum range condition is for level ground. In your problem the point of projection is above the point of contact after the jump. $\endgroup$
    – Farcher
    Commented Oct 3, 2016 at 10:02
  • 1
    $\begingroup$ But couldn't we model this by placing our $x$-axis on the line $d$, then the problem reduces to a projectile motion on level ground? $\endgroup$
    – bonehead
    Commented Oct 3, 2016 at 10:08
  • 4
    $\begingroup$ You then have a component of the gravitational field strength $g$ along the x-direction. $\endgroup$
    – Farcher
    Commented Oct 3, 2016 at 10:15
  • 1
    $\begingroup$ The 45° thing is a pretty special case. For example, try working out the maximum range over a height disparity (e.g. firing over a cliff) - it is surprisingly messier than you'd think, as I recall. $\endgroup$
    – Emilio Pisanty
    Commented Oct 4, 2016 at 17:11

2 Answers 2

Reset to default
3
$\begingroup$

Adding the angles would be like rotating the hill to make it level. However, if you rotated the hill with a simple coordinate transformation you would need to rotate gravity with it. Unfortunately the 45 degree maximum range rule only works if gravity is directly downward, so the equation would no longer be valid.

Improve this answer
$\endgroup$
1
$\begingroup$

In the general case the optimum angle is the bisector of the plane and the vertical. In the case of a horizontal plane this gives you $45^{\circ}$.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.