Free body diagram of block on accelerating wedge

Question

Consider the following system:

I am thoroughly confused about certain aspects of the situation described in this diagram in which a block is placed on a wedge inclined at an angle θ. (Assume no friction everywhere)

Let us consider a few different cases:

Firstly, when the wedge is accelerating toward the left, if I were to observe the system from the ground(assumed to be an inertial reference frame), what will I see? Will I see the block stay put on the wedge and accelerating along with it toward the left or will I see it move down the inclined plane, which itself is moving leftwards?

Secondly, in some problems, they have mentioned that the block is accelerating "down the inclined plane with acceleration $a$ w.r.t the wedge". In such problems, I pick the wedge as my reference frame, introduce a pseudo force and deal with the situation. However, if I were to observe the block from the ground, what would its motion look like to me?

Thirdly, when drawing the free body diagram of a block that is given to be "moving down an inclined plane", in which direction should I assume its acceleration? Directly downward or along the plane?

Fourthly, if given that the block doesn't "slip over the wedge", what is the condition to be used?

As you may see from all this, I am spectacularly confused about all this changing reference frames and accelerations. If anyone could please sum it up concisely, it would be so so helpful for me. I hope that I have conveyed my doubts clearly. If more clarity is required, please let me know and I will edit my question accordingly. MUCH thanks in advance :) Regards.

Answer 1

Rather than answer your individual questions I will give you an overview and then discuss some of the points that you have raised.
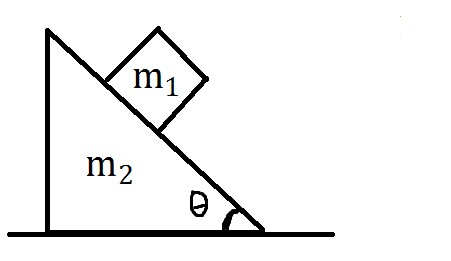
There are many ways of tackling such problems but drawing a few FBDs together with some coordinate axes is always a good to start.

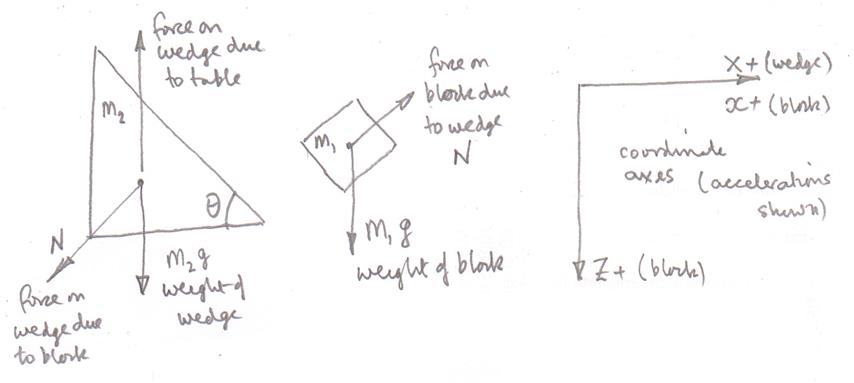
I will use the laboratory frame of reference as it is perhaps then easier to describe what one sees from that reference frame and I will further assume that there is no friction and that everything starts from rest.
The other important assumption for the first part of the analysis is that the block and the wedge stay in contact with one another. Newton's second law can then be applied which will yield equations with the vertical and horizontal accelerations of the block, $z$ and $x$, the horizontal acceleration of the wedge $X$ and the normal reaction between the block and the wedge $N$ as the four unknowns.
The problem is that application of Newton's second law only yields three equations.

As with a lot of mechanics problems the fourth equation comes from the geometry of the system.
The block keeps in contact with the wedge and relative to the wedge it slides down the wedge at an angle $\theta$.
That is, if you sit on the wedge you will see the block accelerating down the wedge but staying in contact. The downward acceleration of the wedge relative to the block is $z$ (the wedge has no downward movement as the table is assumed immoveable) and the horizontal acceleration of the block relative to the wedge is $x-X$.

The acceleration vector diagrams looks like this:

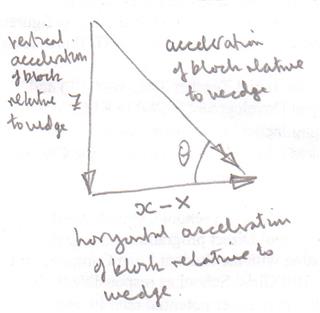
It yield the fourth equation $\tan \theta = \dfrac{z}{x-X}$

I hope that this is sufficient to answer all your questions?

The wedge has to go left and the block towards the right. This must be so in that the net horizontal force on the block-wedge system is zero and so the centre of mass of the system does not move.
Using this idea one can get a equation linking the horizontal acceleration of the block $x$ and that of the wedge $X$ directly; $(m_1\;x + m_2 \; X = 0 +0 \Rightarrow X = - \dfrac {m_1\; x}{m_2} $.

If for some reason the acceleration of the wedge to the left is greater than $X$ in the example above, eg due to an external horizontal force on the wedge acting to the left, then the situation becomes more complicated.
Assume that the force is such that the horizontal acceleration of the wedge to the left stays constant with a magnitude $Y$.

The normal force between the wedge and the block will decrease so downward acceleration of the block $z$ will increase whereas its horizontal acceleration of the4 block $x$ will decrease but it will still stay in contact with the wedge.
In the acceleration diagram remembering that because the acceleration of the wedge is to the left the magnitude of $x-Y$ will increase as will the magnitude of $z$ to ensure that the block stays in contact with the wedge.
So if you sit on the wedge you will see the block staying in contact with the wedge but with a greater acceleration downwards than before.
Sitting in the laboratory frame you again will see the wedge accelerating faster down the wedge but with a trajectory whose angle with the horizontal is greater than the angle of the wedge $\theta$.

The limiting case is reached when the downward acceleration of the block is $g (= z) $ and its horizontal acceleration $x$ is zero.

So in this limiting case $\tan \theta = \dfrac{g}{(-)Y}$

Any further increase in the horizontal acceleration of the wedge to the left will result in the block losing contact with the wedge and undergoing free fall.

I am not entirely sure about the last part of the analysis but the limiting acceleration $Y$ formula seems to predict what one might expect.
As the angle $\theta$ gets smaller and smaller to keep just in contact with the block the horizontal acceleration of the wedge $Y$ has to get bigger and bigger whereas as the angle $\theta$ tends towards $90 ^\circ$ the acceleration of the wedge $Y$ has to get smaller and smaller.

Answer 2

I notice that you are asking about A block on a wedge. This problem is more difficult than it looks, even without friction.

The motion is easy to predict. The wedge moves left, the block moves down and right. Horizontally there is conservation of momentum, so the horizontal position of the Centre of Mass does not move. If you wanted to solve this problem, it is probably easiest using conservation of energy and momentum, rather than force and acceleration. See Block slides on smooth triangular wedge kept on smooth floor. Find velocity of wedge when block reaches bottom.

From the ground frame of reference, the path of the block is an incline with an angle $\theta' > \theta$ which depends on the ratio of $m_2/m_1$. For example, if $m_1>>m_2$ then the wedge accelerates very fast to the left and the block $m_1$ falls vertically down at $\theta' \approx 90^{\circ}$; if $m_1<<m_2$ then the wedge does not move and the block slides down the incline at $\theta' \approx \theta$.

It may help to resolve the acceleration of the block into x and y components. Then $m_2a_2=m_1a_x$. Note that the equations set up by xylong97 are incorrect.

The problem is tackled on many webpages and a few videos. For example : A Problem of Relative, Constrained Motion.

Answer 3

Will I see the block stay put on the wedge and accelerating along with it [...]

Absolutely not, as there is nothing (no force) to pull the block along with the incline.

Draw the free-body diagram of the block, and you only see normal force $\vec n$ and weight $\vec w$. Their sum can never point left.

[...] or will I see it move down the inclined plane, which itself is moving leftwards?

Yes. Unless the incline moves away faster than the block can fall (in which case it naturally doesn't follow the incline's surface).

[...] the wedge is accelerating "down the inclined plane with acceleration $a$ w.r.t the wedge". [...] if I were to observe the block from the ground, what would its motion look like to me?

• The extreme case: If the incline stands still, the block will feel the maximum normal force $\vec n$. It slides down at angle $\theta$.
• The other extreme case: If the incline moves leftwards extremely fast - faster than the block can fall - then $\vec n$ becomes zero. The block falls straight down at $90^\circ$ as if the incline wasn't there.
• The in-between case: If the incline moves leftwards not too fast, $\vec n$ is smaller (but not zero). The block slides down the incline but at a more narrow (larger) angle than $\theta$ (but not vertically at $90^\circ$), since the incline moves away under it simultaneously.

[...] when drawing the free body diagram of a block that is given to be "moving down an inclined plane", in which direction should I assume its acceleration? Directly downward or along the plane?

According to Newton's 2nd law $\sum \vec F=m\vec a$, the acceleration $\vec a$ is in the same direction as the resulting force $\sum \vec F$. So when drawing the free-body diagram (still only containing $\vec w$ and $\vec n$), their sum will point along the acceleration.

In the three cases mentioned above, this resulting force will change direction, according to how fast the incline moves leftwards, because $\vec n$ is affected. So the resulting force and the acceleration will point more and more vertically downwards, the faster the incline moves.

Fourthly, if given that the block doesn't "slip over the wedge", what is the condition to be used?

To avoid that the box slips over the wedge, we must avoid that it slides over the top - which means that we must avoid that it starts sliding in the first place. So, the condition would be that the two objects move equally! In other words, that they have the same acceleration so that none is "dropped" behind.