16
$\begingroup$

Weight is a force. Force is mass × acceleration.

When I'm standing motionless on the scale, how do I have a weight greater than $0~\mathrm{N}$ when my mass is $90~\mathrm{kg}$ and my acceleration is $0$? $90~\mathrm{kg}$ × $0~\mathrm{m/s^2}$ = $0~\mathrm{N}$

EDIT: Thanks, @Inquisitive for showing me that the acceleration component of force need not be explicit and allowing me to understand this concept of weight logically and mathematically.

I weigh 200lbs. 9.8m/s^2 is 32.152ft/s^2. 200lbs ÷ 32.152 = 6.22slugs (about 90kg). 90kg × 9.8m/s^2 = 882N (about 200lbs)

My explicit acceleration of 0 is irrelevant to this equation. The magnitude of gravitational acceleration is the correct acceleration to use in this figure. The word "acceleration" caused the misconception that something would be visually observable, namely movement.

Improve this question
$\endgroup$
8

9 Answers 9

Reset to default
42
$\begingroup$

It's not $ \vec{F} = m \vec{a} $, but $\sum \vec{F} = m \vec{a}$. When you are standing motionless there are two forces acting upon you, the gravitational one, and the normal.

The normal force is acted on you by the floor, and the sum of both is zero, but your weight is still the same.

Improve this answer
$\endgroup$
9
  • 9
    $\begingroup$ Exactly. It is such a common misconception that force causes acceleration. Only the sum of forces does. $\endgroup$
    – Steeven
    Commented Aug 7, 2016 at 19:40
  • 3
    $\begingroup$ The obvious question here is how do you define each $\vec{F}$ individually? $\endgroup$
    – user541686
    Commented Aug 7, 2016 at 21:28
  • 4
    $\begingroup$ @Mehrdad Your question is confusing. You do it by considering your actual problem and looking at the individual forces involved. If there's gravity there's gravity. If there's a floor there's a floor. If there's buoyancy there's buoyancy. And whatever else you find relevant to consider. $\endgroup$
    – Jason C
    Commented Aug 8, 2016 at 0:36
  • 1
    $\begingroup$ @JasonC: It's not really "my question", but I'm trying to say that there's a subtle logical gap that I think might be confusing for a new student. Try to interpret what you said literally: if you're telling them $F$ is not $ma$, then how are they supposed to compute each individual $F$ in your equation and sum them to zero to get the net $a$? Wouldn't they have to use $F = ma$ to get each one (e.g. $w = -mg$)? For someone who's confused and asking a question like this, your answer looks a bit self-contradictory. $\endgroup$
    – user541686
    Commented Aug 8, 2016 at 1:24
  • 1
    $\begingroup$ @Mehrdad I see what you mean. I think the sentences beyond the first in this answer seem to clear that up. There are many ways to explain the concept that net force is the sum of individual forces and that weight just refers to one of those. Of all the answers posted so far, hopefully every reader can find at least one that resonates with them. I think I can at least reword my own answer to address the gap, although there's already a lot of good answers here. $\endgroup$
    – Jason C
    Commented Aug 8, 2016 at 1:30
12
$\begingroup$

Gravity wants to accelerate you at $9.8~\mathrm{m/s^2}$ downward when you are standing on a scale. The scale, which is on the floor, which is on the Earth, wants to resist your downward acceleration. So, the scale must resist that acceleration with $F = m*9.8~\mathrm{m/s^2}$. It shows up as your weight on the readout.

Even though you aren't moving, you are still being subjected to a gravitational acceleration.

Improve this answer
$\endgroup$
15
  • 10
    $\begingroup$ You are not being subjected to a gravitational acceleration if you are not accelerating. You are being subjected to a gravitational force. $\endgroup$
    – jburns20
    Commented Aug 7, 2016 at 22:09
  • 5
    $\begingroup$ @jburns20 I said a "gravitational" acceleration, not a net/resultant acceleration. $g$ is still in play regardless of the person's motion or lack thereof. $\endgroup$
    – Inquisitive
    Commented Aug 7, 2016 at 22:16
  • 3
    $\begingroup$ @Inquisitive, I agree with jburns20. If you aren't moving, you aren't being subjected to an acceleration. By definition, zero velocity is constant velocity, which is zero acceleration. $\endgroup$
    – David White
    Commented Aug 7, 2016 at 23:47
  • 7
    $\begingroup$ Acceleration is a property of an object. You can't have "two accelerations" -- it's a single (vector) value that, in this case, is zero. $\endgroup$
    – jburns20
    Commented Aug 8, 2016 at 4:04
  • 4
    $\begingroup$ @DavidWhite "By definition, zero velocity is constant velocity" No! Zero velocity is zero velocity; constant velocity is constant velocity. These are two completely different things. If you want to experience non-constant zero velocity, just jump in the air and think about what happened between a time at which you were travelling upwards and a time at which you were travelling downwards. $\endgroup$
    – David Richerby
    Commented Aug 8, 2016 at 8:51
11
$\begingroup$

You are missing one or both of the following in your understanding of weight:

  • Many forces can act on an object at once. The actual acceleration of an object ultimately depends on the sum of all those forces.
  • "Weight" refers to just one of those forces, not the net total.

The first point means that just because an object isn't accelerating doesn't mean there aren't forces acting on it. It's just that those forces happen to all sum up to zero in this case. That is, just because $a+b=0$ doesn't mean that $a$ and $b$ themselves are $0$. Weight is just one of those, not the sum.

Here's the two most relevant forces for your question (source):

enter image description here

There are two forces there:

  • Gravity pulling down ($mg$, red arrow)
  • The ground pushing back ($N$, blue arrow)

For an object that isn't accelerating, $N + mg = 0$, or $N = mg$ (for simplification let's just say those are the only two forces acting on the object, that's all that really matters for this illustration). "Weight" is just one of those.

That's the key part.

As an aside: Now as for which one of those we consider "weight" to be it really depends on context and definition. There are a few definitions of weight, the two main ones being:

  • Gravitational Definition: Here we define weight as the force that gravity exerts - in other words, weight is the red arrow. The weight of an object is $mg$. In this definition, an object has a weight that only depends on gravity and its mass. So an object in free fall has the same weight as if it were "at rest", and we don't care about things like buoyancy (so an object's "weight" doesn't change if, say, it's floating in water).
  • Operational Definition: Here we usually define weight as what a scale would read if the object were sitting on it - in other words, it's the blue arrow. In this definition you can say that an object in free-fall is "weightless" (like the guys in the vomit comet, or in orbit). If you choose to include things like buoyancy, etc. (the list is long) you can say things like "an object floating in water weighs less", or whatever is appropriate for context.

The main difference between the two is whether you use "weight" to refer to the force of gravity pulling down on you vs. the force of the ground pushing back on you, since there are other forces besides just gravity that the ground may be counteracting to keep the sum at 0.

I think in common conversation most people mean the gravitational definition (like if you were sky diving and the person next to you asked you how much you weigh you'd probably give them your scale weight... unless you were being pedantic and responded "Gravitationally, or operationally?"). But anyways that's beside the point.

The key idea, in any case, is that just because you aren't accelerating doesn't mean there aren't forces acting on you. The net force acting on you is 0 if your acceleration is 0, but the individual components need not be 0, and "weight" just refers to one of those.

Improve this answer
$\endgroup$
2
  • $\begingroup$ can you elaborate on the long list of forces that counteract weight in your operational definition above? $\endgroup$
    – user36093
    Commented Aug 8, 2016 at 19:54
  • 1
    $\begingroup$ @user36093 Not completely without an actual example. But those include things like buoyancy, centrifugal force from the earths spin, perhaps it is a windy day and a component of the winds force is in the direction of the normal. Maybe the object is a magnet repelling something under the surface. Maybe the object is propelling itself up or down, or has second object sitting on top of it. Maybe there is an alien spacecraft above the object trying their best to grab it with a wimpy tractor beam. Things like that. Anything that causes $N$ to not equal $mg$. $\endgroup$
    – Jason C
    Commented Aug 8, 2016 at 20:09
4
$\begingroup$

A gravitational force equal to $F{g}=\frac{GM_{earth}M_{you}}{R^2}$ is always acting on you. This force is the primary thing and it acts on you irrespective of your state of motion. Acceleration is a consequence of a net force acting on you. If there is no ground beneath your feet, then the ever present gravitational force on you will $\textit{cause}$ you to accelerate. If you are not accelerating it means the ground or weighing balance beneath your feet is counterbalancing the gravitational force acting on you. In other words gravitational force is the (ever present) cause and acceleration is only an effect that manifests itself under certain conditions.

If people use the expression $mg$ to calculate force it is only because they define $g\equiv \frac{GM_{earth}}{R^2}$. It is also equal to the acceleration you would have if you were to be in free fall.

Improve this answer
$\endgroup$
2
$\begingroup$

Weight is the force of gravity acting on your mass. When you stand motionless on a scale, there is a vertical force pushing up, known as the normal force, which exactly equals your weight. The normal force is being provided by the spring that is in the scale that you are standing on. Due to your weight on the scale, this spring is compressed to some degree. The manufacturer of the scale knows how much the spring will be compressed when it supports a given weight, and this is used to indicate your weight as you are standing on the scale.

To directly answer the question of how there can be weight without movement, your weight is exactly equal (and in opposite direction) to the normal force from the scale. Due to this, there are two forces involved, and a free-body diagram indicates that there is no net force on you. If there was a net force on you, you would accelerate in the direction of that force, according to Newton's 2nd Law.

Improve this answer
$\endgroup$
2
$\begingroup$

The force of gravity tends to cause an acceleration of $g=9.8 $m/s$^2$, acting downward. So there IS acceleration involved. However, the ground pushes on your feet and contributes an acceleration of 9.8 m/s$^2$ upward, so that your total acceleration is zero. But your weight is not the force associated with your total acceleration, but with the acceleration which would result from the force of gravity if no other force opposed it.

Improve this answer
$\endgroup$
1
$\begingroup$

Your question is based on the wrong assumption that your weight is equal to the total sum of the forces acting on you. The common notion of weight is instead the force acting on you due to gravitational field.

Improve this answer
$\endgroup$
0
1
$\begingroup$

First, let's consider a few scenarios:

  • A brick lands on your foot. Even though neither the brick nor the foot is moving, you feel pressure (and, well, pain, but let's ignore that for now) - the weight of the brick pressing against your foot.
  • You accelerate in your car, and feel a force pushing you into your seat - the compression of the seat preventing you from passing through the seat.
  • You jump off from a cliff to water. During the fall, you don't feel any force (though the whole experience can be disorienting and confusing, not to mention short, so you might not even notice). When you hit the water, you feel some force again as you slow down.

You can't feel the force of gravity, because it's uniform as far as your sensory equipment is concerned. Imagine your body as a spring - your sensors can tell you when the spring changes load (and gets shorter or longer). But under gravity, the same force "tugs" on your head as on your feet, so the "spring" keeps the same length, and has no net force.

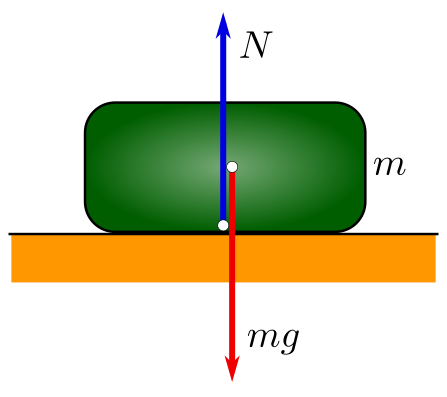
However, things get more interesting where more forces get involved. You stand on the ground, which exerts a force on you that's the same magnitude as the gravitational force, but in opposite direction. This means that even though there are two forces acting on you, you experience no net force - the two forces cancel out.

Now, if the electromagnetic force that prevents you from falling through the ground was uniform, you wouldn't feel anything, and you'd just keep floating as if you were in orbit around the Earth. However, that's not the case here - the force gets stronger as you get closer. It's extremely strong in the area of contact between your feet and the ground (don't forget that it supports your entire weight against the pull of the entire planet), but it doesn't quite reach even into the skin on your feet. However, the skin of your feet is still tugged by gravity, so it wants to go down - only to press against the lower layers. It's this difference in forces that we can perceive - that's how you feel the ground beneath the feet.

A scale works in a similar way. In a typical mechanical scale, you have a static part (in contact with the ground), a spring of some kind, and a moving part you step on. The spring acts similar to the electromagnetic force (in fact, that's what ultimately drives it, but that's not important here) - the more compressed it is, the higher the force it exerts. So once you step on the scale, the spring compresses until it reaches an equillibrium - the force of gravity on your body exactly balances the force the spring (and the scale) exerts on your feet. At this point, there is no longer any (macroscopically important) acceleration - and yet, we can tell that the scale is slightly smaller than before you stepped on it. By understanding the way the spring is compressed under load, we can deduce how much load corresponds to a given compression of the spring.

But the main point in the explanation is the equillibrium. You only get a readout when the two forces balance each other out - that is, the sum of the forces acting on the scale adds up to zero, and there is no net acceleration.

And this is where F = ma comes together - you need to add up all the forces acting on a body to get the acceleration. When you start falling, there is little force opposing your fall, and the acceleration of your body is close to g. As you pick up speed, the air gets less capable of moving away from your path, and starts slowing you down - a force that acts against gravity, so your acceleration gets smaller, even though gravity is as strong as ever. Finally, if you fall far enough, the air resistance becomes so great, that it fully supports your weight against gravity - there is no more acceleration, the forces are balanced. But it's still an equillibrium - if you turned the force of gravity acting on you off for a few seconds, you would quickly slow down and eventually (in a few hours, probably) stop moving entirely.

But through all this, your weight is always the same - it's always exactly the force of gravity acting on your body, whether your body is supported (which makes you feel the weight) or not (the "weightless" feeling).

Improve this answer
$\endgroup$
1
$\begingroup$

First, let us go back to the time when this formula was devised. We knew that an object can be pushed and it can have different magnitudes. Now, the basic problem arose, how to measure this push or the force?

Newton found out that as the force was varied so did the acceleration of an object of mass say $m$. Further, as the force was doubled the acceleration also got doubled for that stationary object (don't confuse yourself with the reference frame, take any inertial frame. An earth can act as an inertial frame to a good approximation). So, acceleration is directly proportional to the applied force. Newton tried to relate this force to the acceleration of the object or you can say the applied force to rate of change in momentum of the object but, if one relate it vice versa, one may go wrong because acceleration is directly dependent on the applied force and not the applied force is directly dependent on the acceleration of the given object of mass $m$

In simple words acceleration is dependent on the applied force but it dosen't makes much sense to say that the applied force is directly dependent on the acceleration of the given object. In your case acceleration was hindered by an equal and opposite force but it dosen't mean that the force wasn't applied.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.