15
$\begingroup$

It seems that the Bloch equations, or a suitable generalization thereof, are enough to phenomenologically model the measurement process in NMR. Has anyone attempted a fully quantum mechanical model of the NMR spin system and measurement coil together?

It seems to me that these coils are intentionally of low quality to ensure no back-action (more phenomenology, so far as I can tell) on the spin system. But perhaps one can engineer more efficient control or parameter estimation strategies with high quality coils and an accurate quantum mechanical model of the measurement interaction, no?

Improve this question
$\endgroup$
12
  • $\begingroup$ I don't quite follow. How would a low quality coil prevent back action? $\endgroup$
    – Joe Fitzsimons
    Commented Nov 1, 2011 at 1:08
  • 2
    $\begingroup$ @JoeFitzsimons: I think of a low-quality coil as coupling to the sample more weakly. Maybe not the right intuition, but I find it at least somewhat helpful... $\endgroup$
    – Chris Granade
    Commented Nov 1, 2011 at 16:26
  • 1
    $\begingroup$ @JohnSidles: As it happens I have a paper with some others on exactly the question of the extraction of information from a spectrometer beyond the standard quantum limit (arXiv:1007.2491). $\endgroup$
    – Joe Fitzsimons
    Commented Nov 2, 2011 at 17:46
  • 1
    $\begingroup$ @JohnSidles: Standard quantum limit often refers to separable states only, whereas the Heisenberg limit is in metrology the Heisenberg limit is the "performance that can't be bettered supposing that standard QM is true". $\endgroup$
    – Joe Fitzsimons
    Commented Nov 3, 2011 at 8:57
  • 1
    $\begingroup$ @ChrisFerrie, stimulated by your general question, yesterday I asked a related concrete question "What Shannon channel capacity bound is associated to two coupled spins?". An early answer by Aram Harrow suggests that even this restricted problem is open. $\endgroup$
    – John Sidles
    Commented Nov 7, 2011 at 8:26

2 Answers 2

Reset to default
8
$\begingroup$

A generic model of a spin coupled to an oscillator is the Jaynes-Cummings model, for which a full-text arxiv search finds 1820 "Jaynes–Cummings" preprints (ouch!). So there is a lot of literature out there.

As it happens, inductive circuit oscillators work worse as one makes them smaller-and-smaller, but mechanical oscillators work better-and-better, thus micromechanical systems are well-suited to studying the dynamics of measurement and noise at the classical-quantum boundary, which I take to be the focus of the query.

For an experimental description of single-spin detection via a mechanical detector, see Rugar, Budakian, Mamin, and Chui "Single spin detection by magnetic resonance force microscopy" (Nature 2004), and for a POVM-style quantum analysis of the (noisy) detection process of this experiment, see our UW QSE Group's "Practical recipes for the model order reduction, dynamical simulation, and compressive sampling of large-scale open quantum systems" (NJP 2009).

For a microscopy-centric look into the future see the PNAS survey Spin microscopy's heritage, achievements, and prospects.

Because Jaynes-Cummings dynamics appears in so many guises, it would be a very considerable undertaking to provide a comprehensive literature review.

If we focus on magnetic resonance imaging and spectroscopy, and adopt Shannon channel capacity as a natural figure of merit, then it is straightforward to compute the quantum limits to this channel capacity (slide from a recent talk):

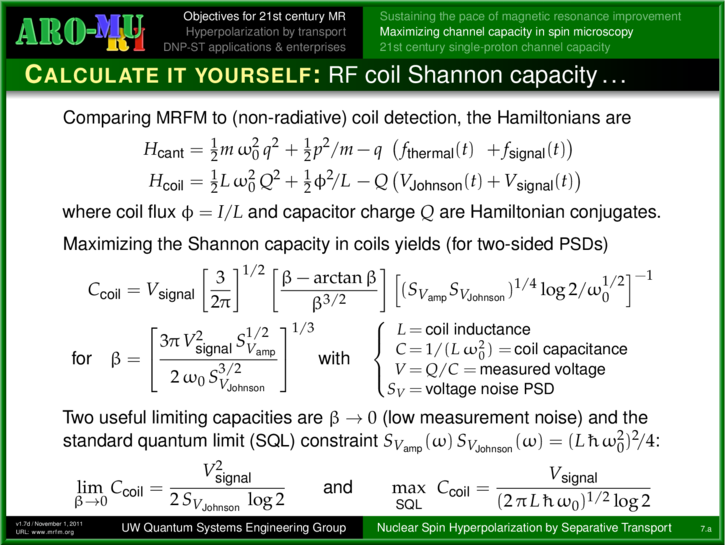
Shannon capacity associated to RF coil detection

(PDF file)

It is a remarkable fact that over the past 65 years, single-spin magnetic resonance channel capacity has doubled approximately once per year, and there is quantum head-room for approximately 27 more such capacity doublings.

Thus, if one is interested to press against quantum limits to speed and resolution in magnetic resonance imaging and spectroscopy, there is ample of work to be done, and plenty of scope for further improvements.

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ So the NMR measurement is a one-way quantum channel (or is it a classical channel?) and you want to ask what the classical channel capacity is? In this information theory context (I like it!), can I rephrase my question as: can one model the NMR measurement as a two-way quantum channel? If so, what is the quantum and classical capacity of this channel? $\endgroup$
    – Chris Ferrie
    Commented Nov 2, 2011 at 17:36
  • $\begingroup$ Chris, in the Hamiltonian shown above, the (transmitting) spin is treated as the source of a classical voltage; there is nowhere in the literature (that I know) a treatment of magnetic resonance channel capacity that is two-way, fully quantum, and experimentally realistic. $\endgroup$
    – John Sidles
    Commented Nov 2, 2011 at 20:37
  • $\begingroup$ @ChrisFerrie, to say a little more, I have often wondered whether a quantum spin microscope, viewed as a "small" sample spin (a proton or election) magnetically coupled by via a scalar or dipolar interaction to a "large" spin (an LC circuit, force microscope, or ferromagnetic resonantor), satisfies some sort of informatic reciprocity relation. That is, which channel has the greater Shannon capacity, "small sample spin" Alice sending bits to "large receiver spin" Bob, or vice versa? This might be a fun problem to work on (my intuition is that Alice can send more bits to Bob than vice versa). $\endgroup$
    – John Sidles
    Commented Nov 2, 2011 at 22:36
  • $\begingroup$ Whoops -- upon back-of-envelop computation I find the opposite -- "loud-shoutin' Bob" can send more bits to Alice than vice-versa. :) $\endgroup$
    – John Sidles
    Commented Nov 2, 2011 at 23:14
6
$\begingroup$

Liquid state or solid state NMR? In the liquid state NMR you can probably model the measurement process and apparatus as a weak measurement to incorporate the effect of the measuring device (say a quantum system with bosonic degrees of freedom). Here is a related paper by Lloyd and Slotine: Quantum feedback with weak measurements which is rather general but is definitely geared towards NMR physics. Despite that, I don't think there is a lot of room for maneuvering with this concept in the liquid state NMR. In solid state NMR (or even quantum dots), one deals with a single copy of the system and the back-action has a more significant effect. As for the high quality coil, superconducting loops are now used as measurement devices for the B field —macroscopic but very quantum systems (similar to a harmonic oscillator but also different). So I'd say the answer is a cautious "yes". The problem is from a practical point of view: there are other sources of noise in control and evolution of the NMR-like spin systems that would make this a far to reach goal.

Sorry, I couldn't leave a comment so I left an answer.

Improve this answer
$\endgroup$
7
  • $\begingroup$ I took his concern to be about the current creating a field which effects the spectrum, rather than about the fact that you are making weak measurements. $\endgroup$
    – Joe Fitzsimons
    Commented Nov 2, 2011 at 7:02
  • $\begingroup$ Could you fix the first link please? $\endgroup$
    – Chris Ferrie
    Commented Nov 2, 2011 at 11:58
  • $\begingroup$ @JoeFitzsimons. I actually don't know enough about this subject but weak measurements may be substituted for weak interactions with the measurement device. That's only one way of looking at this problem. $\endgroup$
    – Kaveh_kh
    Commented Nov 2, 2011 at 13:48
  • $\begingroup$ @ChrisFerrie Thanks, I fixed the link. By the way, I looked at your profile page and you seem to work at IQC in Waterloo. I think you are probably working a few meters from some of the leading experts in this subject who are both great NMR experimentalist and also great theorists. They could definitely provide better answers. $\endgroup$
    – Kaveh_kh
    Commented Nov 2, 2011 at 13:51
  • 1
    $\begingroup$ @Kaveh_kh: Indeed I do get work with one of world leaders in spin physics (In fact, I've wrote a couple papers recently with one). The problem is that quality of answers is not independent of the knowledge of the asker, if you know what I mean. I'd like an answer that abstracts as much of the "physics" away as possible. That is the way that I--and hopefully I'm not alone-- will understand it. $\endgroup$
    – Chris Ferrie
    Commented Nov 2, 2011 at 14:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.