Interactions have a crucial role in the process of making a BEC.
Bose-Einstein Condensation without interactions/collisions
In theory, it is possible to produce a BEC starting from an ideal gas (i.e. a gas of non-interacting particles) of bosons and by cooling it down. Note that most of time, the way the gas is cooled down is never specified, the temperature $T$ of the gas is just taken as a parameter.
More specifically, statistical mechanics tells you that the temperature of an ideal gas gives the mean kinetic energy per particle, i.e. $\langle E\rangle/N\propto k_BT$ where $N$ is the number of particles in the gas.
This means that is possible to define a mean, thermal, de Broglie wavelength $\bar\lambda_T$ for particles inside the gas, such that :
$$
\frac{\langle E\rangle}{N}=\frac{\hbar^2(2\pi/\bar\lambda_T)^2}{2m}\propto k_BT
$$
This implies that $\bar\lambda_T\propto1/\sqrt{T}$. The gas also have a given density $\rho=N/V=1/\bar r^3$ where $V$ is the volume of the gas, and $\bar r$ is the mean distance separating the particles from each other.
For $T$ low enough such that $\rho\bar\lambda_T^3\gtrsim 1$, i.e. $\bar\lambda_T\gtrsim\bar r$, individual wave-functions of the particles start to overlap, meaning it is no longer possible describe independently one by one the state of each particle. Indeed, here particles are identical therefor indistinguishable, so it is needed to build a $N$-body quantum state (which has to be symmetric for bosons) to describe the gas.
It can be shown that from this indistinguishability stems the Bose-Einstein statistics, giving the mean occupancy $N_\textbf{p}$ of a momentum state $|\textbf{p}\rangle$ (remember, the gas is ideal here so there is no interaction/collision between the particles, meaning that $|\textbf{p}\rangle$'s are eigenstates of the system) :
$$
N_\textbf{p}=\frac{1}{e^{(E_\textbf{p}-\mu)/k_BT}-1}
$$
For $N_\textbf{p}$ to be a definite positive quantity, it requires that $\mu$, the chemical potential, to be smaller than the smallest of the $E_\textbf{p}=\textbf{p}^2/2m$ energies, which is simply $E_0=0$, corresponding to the $|\textbf{p}=0\rangle$ ground state.
The total particle number $N$ is then given as :
$$
N=N_0+N_e\quad\text{where}\quad N_0\equiv N_{\textbf{p}=0}\quad \text{and}\quad N_e=\sum_{\textbf{p}\neq 0}N_\textbf{p}
$$
the sum of the condensed fraction $N_0$ (the number of particle in the $|\textbf{p}=0\rangle$ ground state) and the excited fraction $N_e$ (the number of particle in the excited states). The constraint $\mu<0$ implies that :
$$
N_e<N_e^\text{max}=\sum_{\textbf{p}\neq 0}\frac{1}{e^{E_\textbf{p}/k_BT}-1}
$$
meaning that there is a saturation of the population of the excited states. This saturation is the essence of Bose-Einstein condensation. For any $T$, one can be sure that, at least, $N-N_e^\text{max}(T)$ atoms will occupy the $|\textbf{p}=0\rangle$ ground state.
What I wanted to show you with this is that Bose-Einstein condensation requires constraints on both temperature $T$ and density $\rho$. But there is no constraint on the spatial position (the reason being that particles are in the $|\textbf{p}=0\rangle$, meaning that particles are completely delocalized in the volume $V$ because of the uncertainty principle). The condensation occurs in momentum space rather than in real space.
Bose-Einstein condensation with interactions
So far so good, the next question is now, what happens with the interactions? First, by "interaction" (most of the time repulsive), we mean here some momentum exchange, some collision (e.g. dipole-dipole interactions, spin exchange, spin-spin interactions, etc...).
Interactions feels to be critical for the BEC stability since it allows couplings between the condensed fraction and the excited one through the two-body process:
$$
|\text{particle 1},\textbf{p}=0\rangle+|\text{particle 2},\textbf{p}=0\rangle\rightarrow|\text{particle 1},\textbf{p}\neq 0\rangle+|\text{particle 2},\textbf{p}\neq 0\rangle
$$
meaning that interactions can expel particles from the condensed fraction.
Such process is known as quantum depletion.
Without getting into too much details, it can be also shown that, appart this quantum depletion, the BEC phase still stable for (repulsively) interacting particles (people familiar with the field will remember the Bogoliubov approach).
Bose-Einstein condensation in the lab
Actually, people are very clever and have shown that is possible to use the interactions/collisons in order to produce a BEC irl.
If we restrict this discussion to BEC of some atomic species (cf Ariana Grande's comment to your question), laser cooling technics allow you to cool down an atomic sample to a temperature about the order $1$ or $10\;\mu K$ (this is more or less true depending on the atomic species you are using, the most common being Rb87).
In this condition, if you compute $\rho\bar\lambda_T^3$, you will find something which is typically on the order of $0.1$, which is not enough to condense. That is why, most of time, it requires evaporative cooling to reach condensation.
The principle is quite simple. When you want to cool down your hot coffee cup, you usually blow on it. By doing so, you blow away from the surface of the water the hottest particles and cool down the system. In the lab, people are using this idea to cool at very low temperature atomic gas toward condensation.
Atoms are first trapped by lasers in some "atomic cups". Then, by decreasing the power of the trapping lasers, the depth of the trap $\epsilon_\text{ev}$ is gradually decreased and the hottest atoms are expelled from the trap.
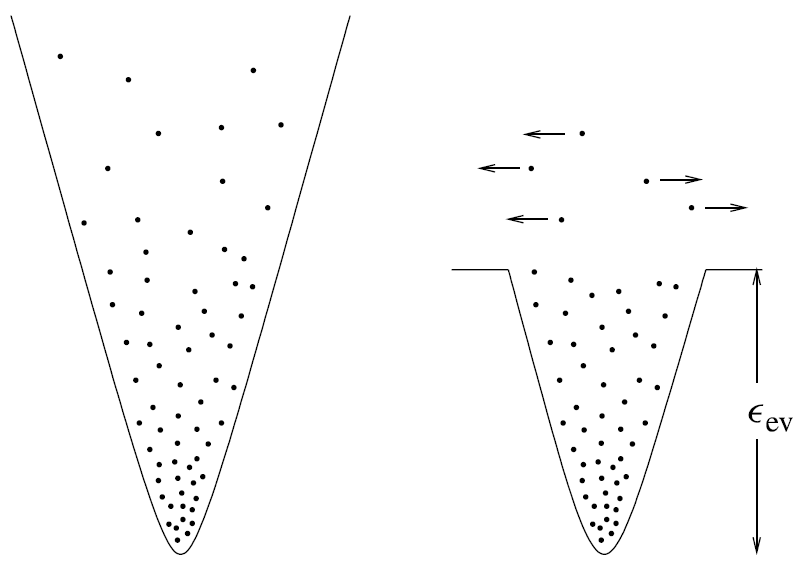 (Source of the image : Bose-Einstein Condensation in diluted gases, C.J.Pethick, H.Smith, Cambridge Press, p91)
(Source of the image : Bose-Einstein Condensation in diluted gases, C.J.Pethick, H.Smith, Cambridge Press, p91)
The interactions are key here : since the atoms can produce collisions with each other, the gas can thermalize, meaning that at each gradual step where the depth of the trap is decreased, the energy loss generated by the loss of the hottest atoms can be redistributed to the all gas, leading to a global decreasing of the temperature.
In general, 99% of the initial atom number is lost by evaporation. But the 1% left can be very cold, with a temperature about the order of $1\,\text{nK}$ , even colder!

