4
$\begingroup$

I've had a bit of a crisis in my understanding of circular motion and I'm hoping to clear it up here.

Would it be correct to say that the condition for a particle to be in uniform circular motion is that there is a net force $\mathbf{F}$ acting on the particle such that $$\mathbf{F}=-\frac{mv^{2}}{r}\hat{\mathbf{r}}$$

Furthermore, if an object is is in uniform circular motion along a vertical path such that, in the critical case, the only force acting on it is gravity, then what stops gravity from making the object fall towards the ground instead of continuing to follow its circular path? (I get that it is the fact that the object has a large enough tangential speed)

By requiring that $ \frac{GMm}{R^{2}}=\frac{mv^{2}}{r}$ is this because we wish to know the conditions that must be satisfied for this object to continue in a circular path (as opposed to taking to the ground due to gravity) under the influence of gravity. Circular motion is characterised by the net force satisfying $F= \frac{mv^{2}}{r}$, and so this relationship must hold in the case in which gravity is the only force acting in order for the object to maintain circular motion?!

Improve this question
$\endgroup$
4
  • $\begingroup$ It's not exactly clear to me what you're asking. Also, I'm not sure how you can keep a ball on a string going at a uniform speed in a vertical circle - it's going to speed up on the way down. I don't think this really affects the question you're asking though. $\endgroup$
    – M. Enns
    Commented Jul 16, 2016 at 14:41
  • $\begingroup$ @M.Enns Apologies for the long windedness. I have updated the post to try and explain my questions better. Uniform circular motion is characterised by an object having constant speed - it's velocity changes (as it's constantly changing direction). If one keeps the radius fixed and the angular velocity constant (difficult to do in practice I know, but this is an idealised thought experiment ;) ), then the speed is necessarily constant, since $v=\omega r$. $\endgroup$
    – user35305
    Commented Jul 16, 2016 at 15:08
  • $\begingroup$ So... you fixed your long-windedness by making the post longer? ;-) Don't be afraid to aggressively remove parts of your post when you edit. Everything is preserved in the revision history. (There's also no need to mark the edited parts with "Edit" or the like. Edit the post to make it look as if you'd written it that way the first time.) $\endgroup$
    – David Z
    Commented Jul 16, 2016 at 15:11
  • $\begingroup$ @DavidZ Point taken. I've edited the post further to try and make it more concise. $\endgroup$
    – user35305
    Commented Jul 16, 2016 at 15:17

5 Answers 5

Reset to default
4
$\begingroup$

Something that is left out of (or insufficiently emphasized in) a lot of textbook treatments of centripetal acceleration/force is how physicist use this fact.

In introductory treatments, uniform circular motion plays a very similar role to equilibrium.

You are expected to read a problem, notice that some object (say a ladder with a fireman on it) is not accelerating and then proceeded to use take advantage of the equations of static equilibrium $\sum_i F_i = 0$ and $\sum_i \tau_i = 0$ to work the problem.

Equivalently you are expected to read a problem notice that some object is following a circular path and say "Oh! I know something about the sum of the radial forces $\sum_i F_i \cdot \hat{\mathbf{r}} = -m \frac{v^2}{r}$. Then you look at the forces in action in the problem and figure out the implication of that constraint.

If the professor is swinging a bucket of water over his head and the water does not fall out and soak the instructor then you know that near the top the water was in circular motion and the net force generated by gravity and the reaction between the water and bucket (which is ultimately supported by the tension in the professors arm) works out to centripetal force. At the slowest speed for which the water doesn't fall out that is the smallest possible value of centripetal force consistent with a circular path. And since the effect of gravity on the water is non-negotialble the only way to reduce the centripetal force is to reduce the reaction force. Therefore, when the reaction force is zero, the bucket has the slowest possible speed that keeps the professor dry. QED.

You've been trying to work the problem forward: I know the force and from that I deduce the action. That works, and is done in a complete treatment of planetary motion, for instance, but the math is complicated.

What the book is expecting you to do is work the problem backwards: the [object] is following a circular path so I know the sum of the radial forces on it, so I can constrain one of the forces...

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ Thanks for such a detailed and well thought out answer. It reflects exactly my thoughts - I never felt that I got a fully satisfactory conceptual understanding from the introductory texts on circular motion. Would it be correct then to say that uniform circular motion is described by the equation of motion $\ddot{r}=\frac{v^{2}}{r}$, such that, whenever the net force acting on an object satisfies $F=\frac{mv^{2}}{r}$, then the object will be in uniform circular motion?! ... $\endgroup$
    – user35305
    Commented Jul 16, 2016 at 22:01
  • $\begingroup$ ... Given this, in the "working forward" sense, does one simply demand that, in the case where gravity is the only force acting on an object, if we want that object's motion to be circular, then gravity must be acting as a centripetal force, such that $mg=\frac{mv^{2}}{r}$? $\endgroup$
    – user35305
    Commented Jul 16, 2016 at 22:01
  • $\begingroup$ The fact that a body following a curved path is accelerating and the magnitude of the transverse acceleration is given by the square of the tangential velocity divided by the radius of curvature is purely kinematic in nature: a curved path means a changing velocity and how fast it changes depends on both how fast you are going and how tightly the path curves. The connection to forces is simply Newton's 2nd law. And yeah, you get the relationship of circular orbits by equating the centripetal force and the gravitational force; but you need a more complex treatment for elliptical orbits. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Jul 16, 2016 at 22:08
  • $\begingroup$ Yes, I appreciate that it will be more complicated for different types of orbits, but I just wanted to check that I'm on the right track considering circular orbits (for the time being). Would you say that I've understood it correctly? (i.e. is what I wrote in my earlier comment to your answer , in reference to circular orbits, correct?) $\endgroup$
    – user35305
    Commented Jul 16, 2016 at 22:25
3
$\begingroup$

In the 1st case, of the object performing circular motion above the ground, presumably a string will keep it moving in a circle when gravity no longer pulls it in the right direction. The centripetal force is provided by a combination of tension in the string and gravity :
$$\frac{mv^2}{r} = T+mg\cos\theta$$ where $\theta$ is the angle between the string and the vertical. Minimum tangential velocity to avoid the string becoming slack at the top of the loop $\theta=0$ (and the particle leaving a circular path) is given by $T \ge 0$, that is
$$\frac{mv^2}{r} \ge mg$$

In the 2nd case, yes - the idea behind $\frac{GmM}{r^2} = \frac{mv^2}{r}$ is that this is the condition for gravitational attraction to provide the centripetal force.

Improve this answer
$\endgroup$
9
  • $\begingroup$ Would it be correct to say then that in order for the motion of an object to be circular the net force acting on the object must satisfy the constraint $F_{net}=\frac{mv^{2}}{r}$, such that, in the case where gravity is the only force acting on an object, it must satisfy $mg=\frac{mv^{2}}{r}$? $\endgroup$
    – user35305
    Commented Jul 17, 2016 at 20:01
  • $\begingroup$ Yes, that is correct. $\endgroup$
    – sammy gerbil
    Commented Jul 17, 2016 at 20:10
  • $\begingroup$ Ok cool, I think that's cleared up my confusion, thanks! One last thing, would it then be correct to say that if this condition is violated, such that the tangential velocity of an object is $v<\sqrt{gr}$, then the object will follow a parabolic path, falling towards the ground? $\endgroup$
    – user35305
    Commented Jul 17, 2016 at 20:17
  • $\begingroup$ Yes : If only gravity is acting (T=0, eg the string breaks or becomes slack), the object follows a parabolic path from whatever point this becomes true, until it is no longer true. $\endgroup$
    – sammy gerbil
    Commented Jul 17, 2016 at 20:34
  • $\begingroup$ But the path will only be parabolic if the gravitational force doesn't satisfy the constraint $mg=\frac{mv^{2}}{r}$ (or equivalenty, the tangential speed of the object doesn't satisfy $v=\sqrt{gr}$), right?! $\endgroup$
    – user35305
    Commented Jul 17, 2016 at 21:01
2
$\begingroup$

The condition for circular motion you stated is right to some extent.This condition not only holds for uniform circular motion,but also for non uniform circular motion.In non uniform circular motion,there will be a tangential force changing the velocity of the particle.The force perpendicular to the velocity (centripetal force) will also have to keep changing as the velocity of the particle changes.This case is non uniform circular motion as the velocity keeps changing.

Coming to your question, the condition that gravity is the centripetal force at the topmost point is correct provided that the tension is 0.For the critical velocity gravity will provide the centripetal acceleration, whereas for higher speeds (at the top) tension will also act as gravity alone cant provide the acceleration.

The particle does fall down under the force of gravity at the topmost point. Imagine another particle of the same mass at the same speed moving in the same direction,but no string is attached to it. Lets say both particles are at the same height and velocity at time t=0. During infinitesimal time interval dt (dt is exceedingly small tending to 0) both of them will have the same trajectory as all the kinematical quantites are the same - gravitational force,velocity and height.

But after dt the particle attached to the string will have another force - the tension.This tension is perpendicular to the particle's velocity and provides the centripetal acceleration along with gravity.

You can resolve the gravitational force along the string and tangential to it .The tangential one will increase the velocity and the perpendicular one along with tension will cause it to move along a circle.(At the topmost point gravity doesnt have any tangential component.But at lower points it will have a tangential component.)

The other particle has no other force on it except gravity and will fall to the ground.

*I didnt write any equations here because this is my first answer and I am still learning how to show them here.

Improve this answer
$\endgroup$
9
  • $\begingroup$ Thanks for the answer. What confuses me is that the condition $\frac{GMm}{R^{2}}=\frac{mv^{2}}{r}$ implies that the object must be travelling at a minimum tangential speed on order to remain on a circular path. An object that is subject to a gravitational force won't necessarily travel on a circular path - if it doesn't have enough tangential velocity to travel along a circular path, it'll follow a parabolic path and not a circular one. Importantly, it won't satisfy the equation $\frac{GMm}{R^{2}}=\frac{mv^{2}}{r}$... $\endgroup$
    – user35305
    Commented Jul 16, 2016 at 17:50
  • $\begingroup$ ...This is why I was asking, does one demand that this equation is satisfied in order for the particle to remain in circular motion at the top of the path and not follow a parabolic trajectory?! $\endgroup$
    – user35305
    Commented Jul 16, 2016 at 17:50
  • $\begingroup$ Yes the particle should have that speed at the top so as to move in a circle.For it to have that speed at the top,it must be given a certain speed at the bottom.You can calculate the speed to be given by conserving its mechanical energy. $\endgroup$
    – cobra121
    Commented Jul 16, 2016 at 17:56
  • $\begingroup$ So does one (in a sense) set the conditional equality $\frac{GMm}{R^{2}}=\frac{mv^{2}}{r}$ "by hand". By this I mean that, one wishes to determine the conditions for the object to remain in circular motion at the top of its path, and by setting this equality this guarantees that the motion is circular, as long as $v=\sqrt{gr}$?! If this is the case, would this be the same for the case in which a satellite is orbiting around the Earth? $\endgroup$
    – user35305
    Commented Jul 16, 2016 at 18:12
  • $\begingroup$ The particle should have a speed greater than or equal to the critical velocity which can be computed by this equality to remain in circular motion.The satellite case is somewhat different as the satellite only experiences the gravitational pull.The particle can have higher velocities and tension will increase so as to keep it in a circle.But if you want the satellite to have a higher velocity you would have to increase its orbit radius around the earth as there is no other force. $\endgroup$
    – cobra121
    Commented Jul 16, 2016 at 18:32
2
$\begingroup$

Gobind Singh's answer is good. I just wanted to add a few quick illustrations to speak to your question "what stops gravity from making the object fall towards the ground instead of continuing to follow its circular path?". Like Gobind said, imagine it there was no string attached to the mass. It would fall straight to the ground but only if it had no initial horizontal velocity, otherwise it would follow a parabolic path. If the speed is too slow that parabolic path lies inside the circular path described by the taut string and the string goes slack. If the speed is too fast the parabolic path lies outside the circle and additional force in the form of tension is required.Sketch of vertical circular motion

Improve this answer
$\endgroup$
5
  • $\begingroup$ Thanks for your answer, and the illustrations. My confusion really relates to the equation $\frac{GMm}{R^{2}}=\frac{mv^{2}}{r}$ and how to interpret it?! Does one simply require that this equation holds in order for the object to remain in circular motion at the top of its path, hence leading to a condition on the tangential speed of the object. It seems to me that tgis equality won't always hold, for example, if the speed is too low, then the object will not satisfy this equation of motion and will follow a parabolic path. $\endgroup$
    – user35305
    Commented Jul 16, 2016 at 18:06
  • $\begingroup$ $\frac{mv^{2}}{r}$ is the net force required to go around a curve with a radius r and at a speed v. $\frac{GMm}{R^{2}}=\frac{mv^{2}}{r}$ is true when gravity is the only force acting on the mass, i.e. the string is slack. More generally you could write $\frac{GMm}{R^{2}}+T=\frac{mv^{2}}{r}$ where T is the tension. $\endgroup$
    – M. Enns
    Commented Jul 16, 2016 at 18:14
  • $\begingroup$ So would it be correct that, if gravity is the only net force acting on the object, then in order for its motion to be circular (i.e. to remain at a fixed radius $r$ from a given point, as it moves around that point) it must satisfy the equality $\frac{GMm}{R^{2}}=\frac{mv^{2}}{r}$ (since, as you say, for a force to cause an object to follow a circular trajectory, that force must be equal to $\frac{mv^{2}}{r}$)?! $\endgroup$
    – user35305
    Commented Jul 16, 2016 at 19:57
  • $\begingroup$ yes - now that is just going to be momentarily true, as soon as its past the top things change... $\endgroup$
    – M. Enns
    Commented Jul 16, 2016 at 19:59
  • $\begingroup$ Right, yes. After it passes over the top there is then tension (or normal force) additional acting on the object to provide a centripetal force. Would what I put be correct then, in particular the very last comment that I wrote (about orbital motion around a planet) to Gobind Singh's answer (I think I understand the concept properly now, but I just want to make sure)?! $\endgroup$
    – user35305
    Commented Jul 16, 2016 at 20:17
1
$\begingroup$

Would it be correct to say that the condition for a particle to be in uniform circular motion is that there is a net force FF acting on the particle such that $$ \mathbf{F}=−\frac{mv^2}{r} \mathbf{\hat{r}} \,.$$ where m and v are the mass and tangential speed of the particle, respectively, and r is the radial distance from the particle to the point about which it is performing uniform circular motion, with r^ being the radial unit vector pointing radially from this point to the particle.

I think your above statement is correct for an Uniform circular motion.

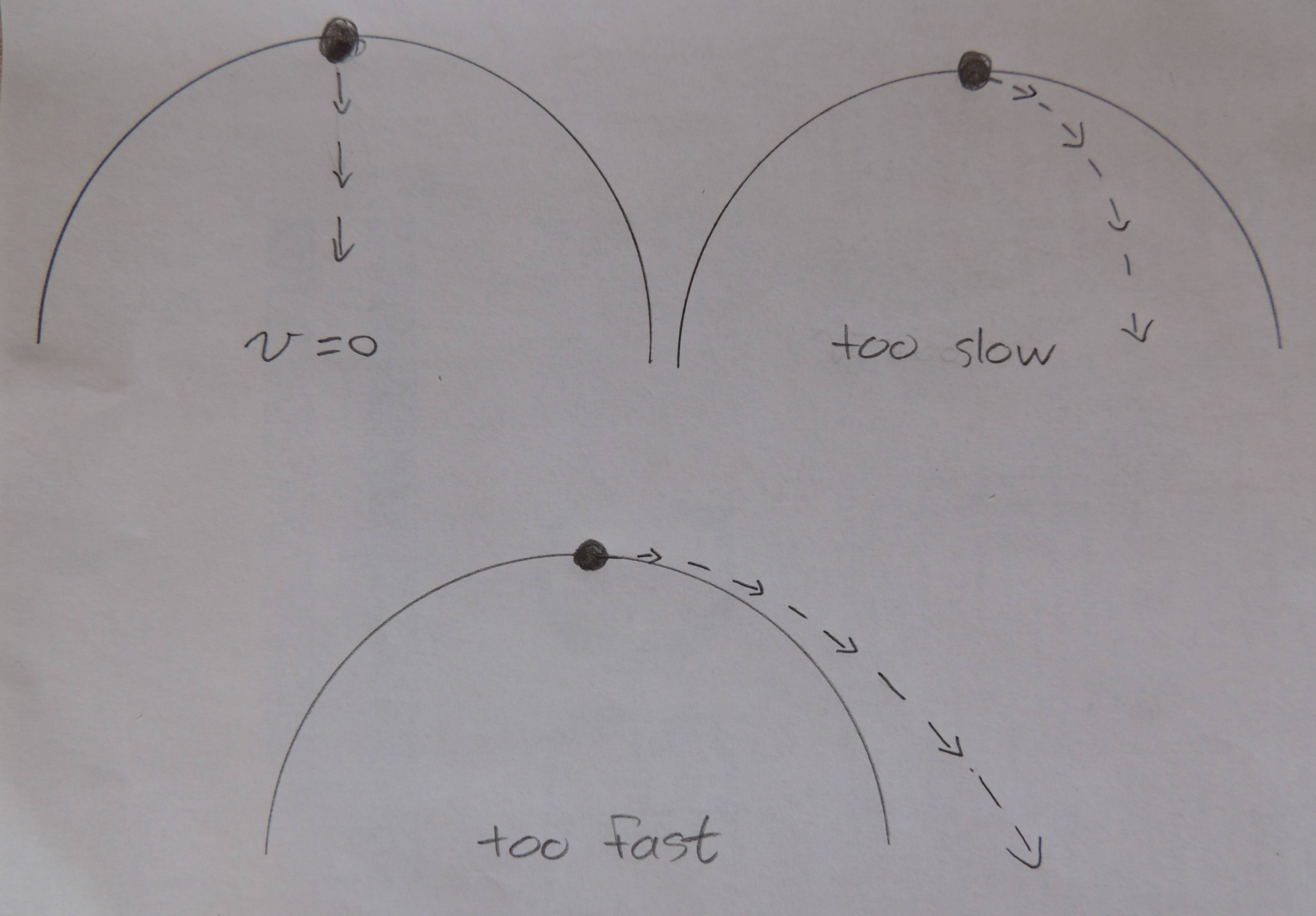
But afterwards you apply it to a body rotating in a vertical circle ...which of course is a motion in a circular path but the action of gravitational pull on the particle changes the energetics of the path.

As the body moves up its potential energy increases and this must be at the expense of its kinetic energy:

Therefore if it has a velocity say $v(l)$ at the lowest point it must get reduced as it proceeds further up on the circle and if it reaches the top point the velocity must have reduced such that the change in kinetic energy must equal to the the increase in potential energy i.e. $2rmg$ if mass of the body is m and radius of the circle is r.

If $v(t)$ is velocity at the top then $$\frac{1}{2} m \left( v(l)^2- v(t)^2 \right) = 2rmg \,,$$ and this situation leads to change in speed of the body at each point of time in its travel.

So, its a case of Non-uniform circular motion and your above statement can not be applied in total.

However ,if the body is moving on a circular path the force needed to maintain its path i.e. the centripetal force will always be acting including the lowest point and the highest point -

the tension in the string will be different -as the tension will represent at each point a force proportional to square of velocity (including a component of weight)...the tension will vary throughout the motion.

If you wish to have tension to be zero at the top -it will mean that the gravitational pull will provide the centripetal force and the observer sitting on the body will not feel the force - a state of weightlessness. The crossing speed at the top will be such that $$m \frac{v(t)^2}{r} = mg$$ Really this happens in the case of earth's satellite moving on a circular path and the centripetal force is provided by the gravitational pull and the man in the satellite does not experience any gravitational pull- a state of weightlessness.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.