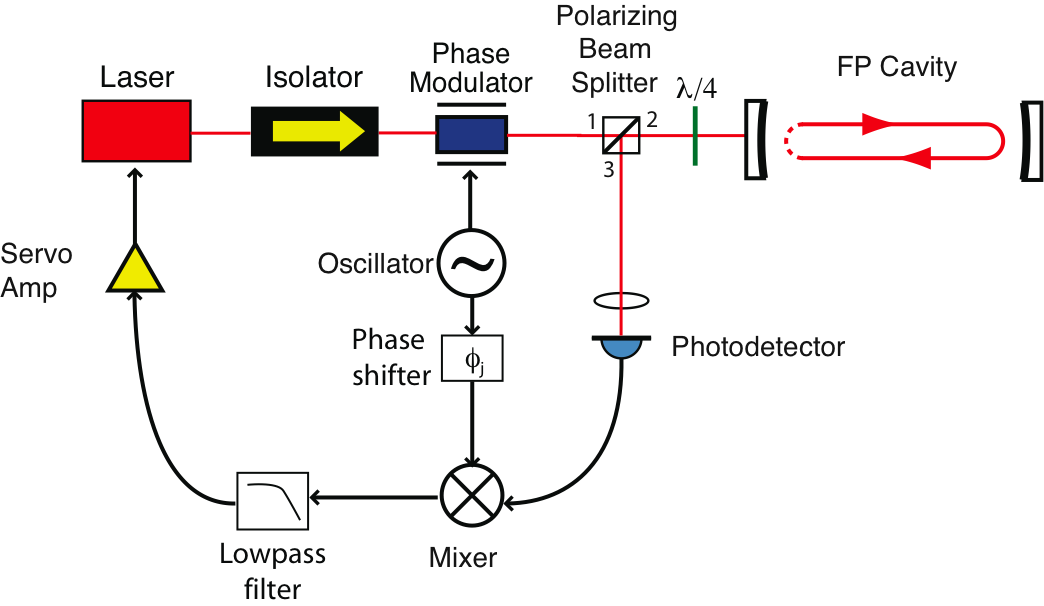
I am having some difficulty understanding the Pound-Drever-Hall frequency stabilisation technique, when locking a laser to a stable cavity.
As far as I understand:
- We emit the laser frequency, f0.
- This signal is modulated with sideband frequencies, f0 ± fm.
- This is fired towards the Fabry-Perot cavity, which has a resonant frequency fc. As f0 ≠ fc, some of the light is reflected at the entrance to the Fabry-Perot cavity, known as the "promptly reflected beam", picking up a phase shift of π/2 like any standard mirror reflection.
- The rest of the f0 signal is transmitted into the cavity (sidebands are never transmitted).
- As f0 is close to fc, the light is able to somewhat resonate in the cavity, with a phase shift of almost 2π picked up on each complete transit of the cavity (it would be exactly 2π at perfect resonance).
- Small amounts of the light periodically escape the cavity, called the "leakage beam".
- There is therefore a phase difference between the promptly reflected beam and the leakage beam, which is just under π (if it were π, such as at perfect resonance, these two beams would cancel exactly).
- The two beams interfere - very close to antiphase - to produce a wave with the two sidebands unchanged and the f0 peak reduced in amplitude and phase-shifted sideways (does the shift directly depend on being above or below resonance?).
- This signal is now incident on a photodetector.
- This signal is now mixed with the modulation frequency.
This is where my understanding breaks down. Why mix this signal with the modulation frequency? I would have expected you to mix the output signal with the initial laser signal, such that the shift in phase could be determined and hence accounted for.
From what I've read, the two different frequency signals produce the beats phenomenon in the mixer, with an internal frequency (f0 + fm) and the envelope/amplitude frequency (f0 - fm). This is low-pass filtered to keep the (f0 - fm) component, and this is the error signal needed to correct the frequency emitted to correctly match the resonant frequency of the Fabry-Perot cavity.
But we want the error signal to be the difference between the resonant frequency and input frequency. I don't understand why we have used the modulation frequency/sidebands at all! Please answer using similar level of language as I have used here. I have attached a schematic of the Pound-Drever-Hall system from Wikipedia below.
Many thanks in advance!