2
$\begingroup$

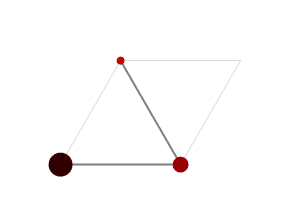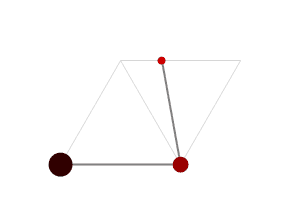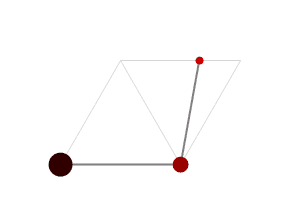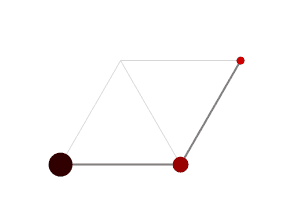
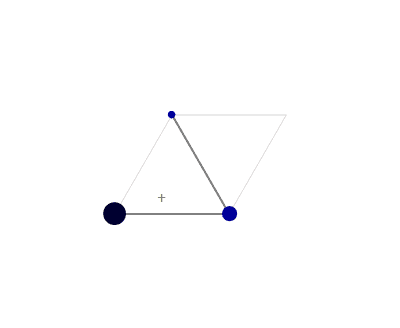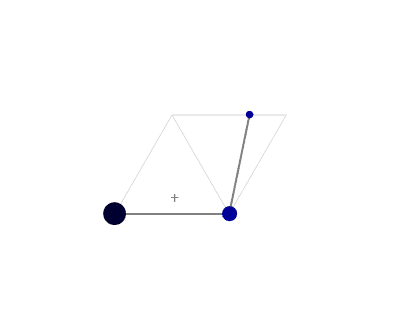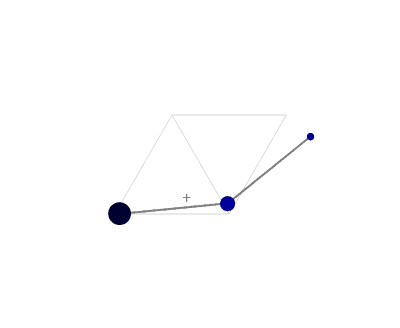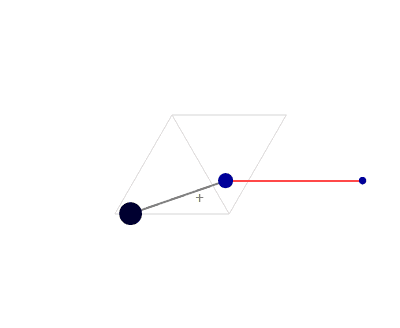
I'm stuck solving this problem with three small balls of masses $m$, $2 m$ and $3 m$ on a smooth table, connected by two equal, light inextensible strings as shown, and initially at the vertices of an equilateral triangle. The strings are initially taut, the two larger masses are at rest and the smallest mass moves to right with some initial speed.

enter image description here

Eventually $m$ comes to a position where the string to $2 m$ gets taut again, and $m$ exerts an impulse on $2 m$, which in turn exerts some impulse on $3 m$. Mass $m$ and initial speed is known, which leaves me with six unknowns, or three 2D velocities after the pull. But I can only muster five equations: two from the conservation of momentum, one from conservation of energy, one from knowing that the impulse on $3 m$ is in the horizontal direction, and one from knowing that the velocity difference of $m$ is in the direction to $2 m$ at the moment of the pull. What am I missing?

Update

Following the answer by @Farcher below, the two-stage calculation yields velocities:

$\vec{v_1} = (2 v_0/3, -\sqrt{3} v_0/3)$

$\vec{v_2} = (-v_0/30, \sqrt{3} v_0/6)$

$\vec{v_3} = (2 v_0/15, 0)$

Where $v_0$ is the initial speed of $m$. Total kinetic energy is conserved, and the center of mass is undisturbed by the event as the second animation shows. Eventually $m$ distances too much from $2 m$ and should interact with it again.

enter image description here

Improve this question
$\endgroup$
7
  • 1
    $\begingroup$ Conservation of total angular momentum? $\endgroup$
    – Martin Ueding
    Commented Jun 15, 2016 at 8:13
  • $\begingroup$ @MartinUeding That's likely the case, but I can't write it down. Let's say the pivot is at $m$ at the moment of the pull. I don't know the direction of where the $2 m$ will go and thus its torque distance. $\endgroup$
    – BoLe
    Commented Jun 15, 2016 at 8:22
  • $\begingroup$ Taking the center of mass of the whole system as the pivot point will give a conserved quantity. $\endgroup$
    – Martin Ueding
    Commented Jun 15, 2016 at 8:34
  • $\begingroup$ @BoLe Energy can't be assumed to be conserved within the Kinetic Energies of balls. $\endgroup$
    – user87745
    Commented Jun 15, 2016 at 10:18
  • 2
    $\begingroup$ @Bole You can show your equations. Of course there's no potential energy since the strings are 'inextensible'. $\endgroup$
    – philip_0008
    Commented Jun 15, 2016 at 13:22

1 Answer 1

Reset to default
1
$\begingroup$

To be able to solve this problem you should assume that all interactions are elastic so kinetic energy is conserved.

Do the problem in two stages and consider each stage as a one dimensional interaction:

  • The interaction of mass $m$ and mass $2m$ along the line of the string joining them and this should give you the velocity of mass $2m$.

  • The interaction of mass $2m$ (with the velocity found in the first stage) and mass $3m$ along the line of the string.

Improve this answer
$\endgroup$
3
  • $\begingroup$ I did that intuitively first. But shouldn't one after the two stages you describe plug the calculated velocity of $2 m$ into the next interaction with $m$ and iterate the stages in this way? That doesn't converge though. $\endgroup$
    – BoLe
    Commented Jun 15, 2016 at 16:09
  • $\begingroup$ In this two step analysis mass $m$ gives mass $2m$ some momentum and then that momentum which mass $2m$ has is shared with mass $3m$ and it is the final momentum that mass $3m$ which will give you the answer to the question. To save time on the algebra you might find this Wikipedia article of use? en.wikipedia.org/wiki/… $\endgroup$
    – Farcher
    Commented Jun 15, 2016 at 16:21
  • $\begingroup$ I see, now. I actually arrived at the solution but then obviously miscalculated the total energy, and panicked ... What I would like to calculate next, are the values after the second pair of interactions (see update to the post), and the third and so on. I think these should converge. $\endgroup$
    – BoLe
    Commented Jun 15, 2016 at 18:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.