NASA published a computer generated black hole image.
In the image you can clearly see the event horizon and the light of background stars graduating from "smeared" to normal.
However, between the event horizon and the smeared stars there is an area which looks rather normal. Why is this? If the smear is due to gravitational force, it should start right from the event horizon across the image, and not after some seemingly random distance.
Fleshing out my answer in the comment above:
The light from all the stars in the picture is lensed (i.e. it's path bent) by the black hole (or BH). The closer it passes to the BH, the larger the angle it's path is bent by.
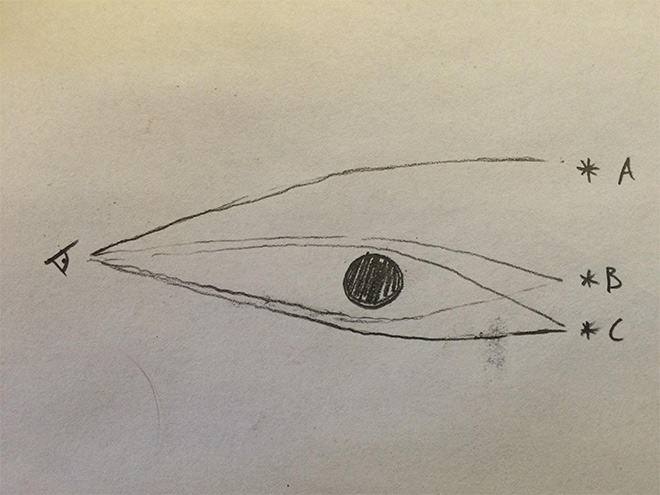
Stars far (in projection) from the BH, like A, are hardly lensed at all (in fact I significantly over-egged it in my diagram, should be a much straighter line from A to the observer!)
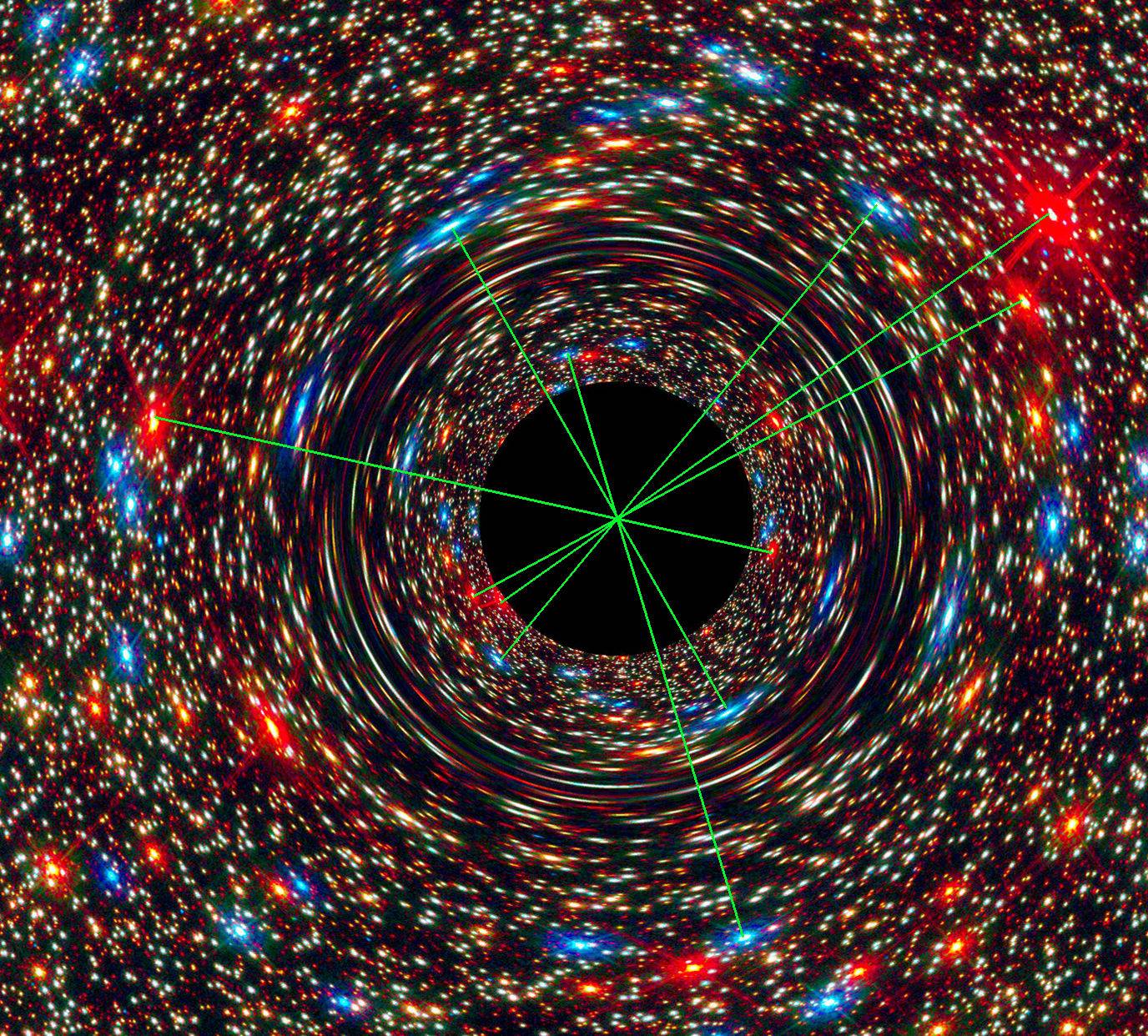
A star directly behind the BH (B) will be lensed in a ring (https://en.wikipedia.org/wiki/Einstein_ring). This is because multiple light rays, all bent by the same amount, make their way to the observer. In 2D only two of these rays are visible, but in 3D it forms a ring. This is the smear you're seeing, a very small area of space projected across a large area, giving a nasty smudge effect. Think of zooming too far on any picture and it becoming grainy.
But stars slightly off-center, like C, will also have light emitted in two different directions that reaches the observer. The first, the bottom ray in the picture, does not pass very close to the black hole, is not bent by a huge amount, and appears outside of the "smear" ring. The second must pass very close the BH, be diverted by a large angle, and will appear within the ring.
Unlike the area directly behind the black hole, this area of sky is projected over a similar (or even smaller) area than it came from, and so does not appear to be a grainy smudge.
So the stars within the ring can all be seen twice, once on the outside of the ring, then again, on the opposite side of the BH, within the ring.
See this excellent image that Previous has made and given me permission to reproduce (as well as his great, more optics based explanation below) to trace which stars are being seen twice.
A magnifying glass will produce an upright, enlarged (virtual) image when the distance to the object is smaller than the focal length, and an inversed image when the distance is larger than the focal length (in which case the size of the image decreases rapidly with increasing distance).
(image by cmglee, wikimedia commons)
For a black hole, the gravitational lensing is stronger closer to the black hole: the focal length is variable, and shortens the closer you come to the black hole. Objects will have two images, an enlarged upright image further from the center, and an inversed (for the most part reduced) image closer to the center. In between you get a region where (angular) magnification becomes maximal, resulting in a "smeared" image.
The "smeared" part is the region where the image switches from upright to inverse (the equivalent of the region where the distance is close to the focal length, like the letters h, i, j in the picture).
