What are some phenomena that can not be described without the help of Newton's third law of motion? [closed]

Question

What are some phenomena that can not be described without the help of Newton's third law of motion? All the phenomena I can think of can be explained with the help of Newton's first law or second law. Are there phenomena that require the help of Newton's third law?

Answer 1

TL;DR: every time you use momentum conservation.

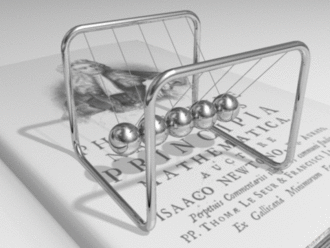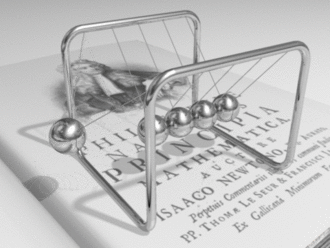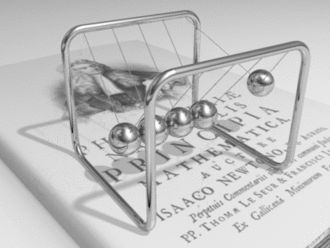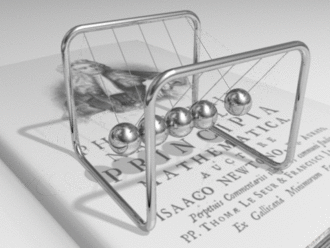
One way to see this is to take a close look at Newton's cradle:

Image is published under GNU Free Documentation License

You can start with Newton's second law:

$$\mathbf{F}=m\mathbf{a}=m\frac{d\mathbf{V}}{dt}$$

By calculating the scalar product with the velocity vector on both sides of the equation we get the expression for the kinetic energy T:

$$ \mathbf{F}\cdot \mathbf{V} = m \mathbf{V}\cdot\frac{d\mathbf{V}}{dt}= \frac{m}{2} \frac{d(\mathbf{V}\cdot\mathbf{V})}{dt} = \frac{m}{2} \frac{dV^2}{dt}=\frac{dT}{dt}$$

Integration over a path in a conservative scalar field then gives energy conservation. With the potential energy $V$ this looks like $$T_1+V_1=T_2+V_2$$

Just taking energy conservation into account we could get several solutions for different balls moving at different speeds. So something is missing to explain the movement we see. What we still need is conservation of the momentum. This is the point where Newton's third law comes into play. With its help we know that if the ball A impacts on the set of balls B, then they feel a force of $$\vec {F}_{A \to B} = -\vec {F}_{B \to A}$$

With that we can derive momentum conservation:

$$m_A\cdot v_A = -m_B \cdot v_B$$

With momentum conversation the multiple solutions break down to a single one. And what we get is the movement of the cradle.

See also: Does Newton's third law apply to momentum or to forces?

Answer 2

The reason why one often thinks that all the familiar phenomena can be explained just on the basis of the second and the first law of Newton is that it is not clearly emphasized (mainly in school textbooks) that Newton's second law for a system of particles can take the form of $F_\textrm{external}=\dfrac{\mathrm d}{\mathrm dt}(p_\textrm{system})$ only when it takes in Newton's third law. Otherwise it would have been just $F_\textrm{net} = \dfrac{\mathrm d}{\mathrm dt}(p_\textrm{system})$.

The elementary form of Newton's second law is simply $F_\text{net}=\dfrac{\mathrm d}{\mathrm dt}(p_\textrm{particle})$.

When one tries to derive the dynamic law for the total momentum of a system the second law helps to reach $$\frac{\mathrm d}{\mathrm dt}(p_1+p_2+\ldots +p_n) = \frac{\mathrm dp_1}{\mathrm dt}+\frac{\mathrm dp_2}{\mathrm dt}+\ldots +\frac{\mathrm dp_n}{\mathrm dt}=F_\textrm{net1}+F_\textrm{net2}+\ldots+F_\textrm{netn} = {F_\textrm{net}} \;.$$

But the $F_\textrm{net}$ reduces to $F_\textrm{net-external}$ only when one assumes that the forces of particles (of the system) on each other cancels out because they are equal in magnitude and opposite in direction. (Which is what we will call Newton's third law for the moment.) Actually, a stricter form of the third law asserts that these forces also lie along the same line of action and thus helps cancelling out terms of internal torque when one tries deriving the dynamic law for the total angular momentum of a system.

PS: Though it should be kept in mind that neither the weak nor the strong form of Newton's law has unlimited validity. The law clearly comes into big question marks when viewed in the light of relativity of simultaneity. But even in pre-relativistic physics, many simple cases of electrodynamic interactions clearly don't follow any form of Newton's third law.

Answer 3

Newton's third law emanates from the fact that the momentum of an isolated system is always conserved viz.

$$\mathrm d\mathbf p_1 +\mathrm d\mathbf p_2 ~=~0 \;.$$

From this, it can be inferred that

$$\int_{t_\mathrm i}^{t_\mathrm f}~ \mathbf F_{21}~\mathrm dt ~=~ - \int_{t_\mathrm i}^{t_\mathrm f}~ \mathbf F_{12}~\mathrm dt\tag 1$$

It could be that the two forces $\mathbf F_{12}$ and $\mathbf F_{21}$ might be unrelated without violating $(1)\;.$

However, failing to cite any evidence to the contrary, we can conclude that at every instant $$\mathbf F_{12}~=~- \mathbf F_{21} \;.$$

And that is Newton's Third Law of motion which is valid for any mechanical collisions etcetera.

But, it should be kept in mind that no interaction takes place instantaneously.

It is noteworthy to quote A.P.French in his book Newtonian Mechanics:

[...] There is no difficulty as far as far as "contact" collisions between ordinary objects are concerned. But in situations in which objects influence one another at a distance, as for example through the long range forces of electricity or gravitation, Newton's Third Law may cease to apply. For no interaction is transmitted instantaneously, and if the propagation time cannot be ignored in comparison with the time scale of motion, the concept of instantaneous action and reaction can no longer be used. ...

So, the above quote explicitly makes it clear when it is valid to use the Third Law.

---

That is not true, even when influeance is at a distance, momenta do balance. The Earth exerts same pull on the Sun as the Sun on the Earth, electron on proton etc

'momenta do balance'.... hmm, sometimes, it is vexing on how misinterpretation leads to wrong conclusion. Never ever have I said, it is not conserved for an isolated system. Probably the asker couldn't get what Mr. French wanted to tell. (He also used the word may;but that's trivial).

The main point is echoed in his statement:

[...] no interaction is transmitted instantaneously, and if the propagation time cannot be ignored in comparison with the time scale of motion, the concept of instantaneous action and reaction can no longer be used.

Momentum would be conserved even then also but not instantaneously thus violating the Third Law as he explicitly mentioned.