I tried to post this question on Computational Science SE, but I was really unsure about the most appropriate SE site. The question didn't attract a lot of attention, and since Physics SE was my second-best guess, I try again here. I have slightly modified the question, in order to make it in-topic for this site.
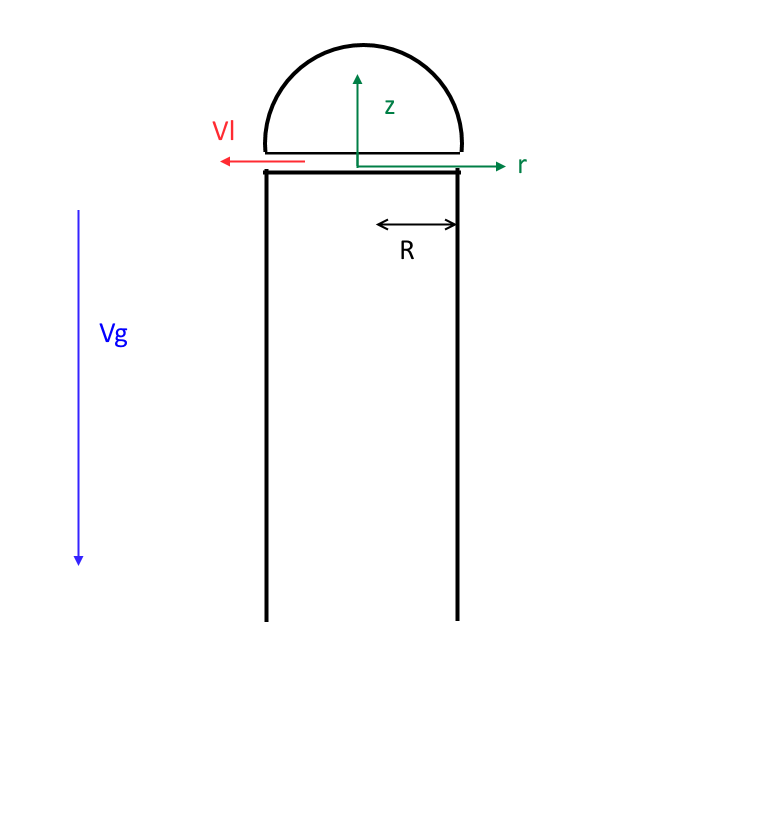
Consider a semicylinder of radius $R$ with a round top, i.e., an axisymmetric solid which extends to infinity in one direction along its axis, and has a round head in the other direction. Something like this Rankine solid. The semicylinder is vertical, and at the junction between the round top and the remaining semicylinder ($z=0$) there is a slit (or a collection of small holes along the circumferential direction, but I think the slit would be easier to model), through which liquid with a very low velocity $V_g$ exits and falls around the semicylinder, under the effect of gravity. Around the cylinder, gas flows in the negative $z$ direction, with velocity $V_l$, thus pushing the film towards $z=-\infty$. At the same time, the semicylinder is heated from inside. The heat flow rate is not very large, but the liquid film should be very thin ($V_l $ is small, and $V_g$ is large), so I guess there may be increased evaporation from the film.
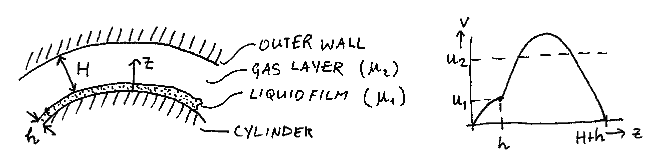
I need to dimension the system so that a thin film of liquid is generated around the cylinder. For example, I can imagine that I should make $V_l$ quite small, otherwise I would get a fountain, and not a liquid film around the cylinder. In particular, I need to estimate the liquid film thickness $h$. To start, heating may be neglected, but I would like to at least have an idea of how the film thickness would be affected by heating. I guess the estimate will be a function of $R$, $V_l$, $V_g$, as well as liquid and gas viscosities and densities.
Probably $h$ will also be a function of $z$. Intuitively, film acceleration due to gravity will tend to reduce the film thickness (by conservation of mass). On the other hand, I think friction between gas and liquid will tend to bring the liquid speed to that of the gas, and stop accelerating. So maybe there is an asymptotic thickness.
Can you help me?
EDIT: I haven't specified parameter values, because I'd rather have an expression of $h$ as a function of $\rho_g, \rho_l, R,\dots$, than just a number would like a generic answer, and also because the setup hasn't been frozen, thus I could make it bigger/smaller/increase flow rates/reduce them/etc. if that is needed to get a thin film. Anyway, just to put the problem in context, I give reference values for a couple parameters, but I want to stress that they are not definitive, and they can be modified if you believe that to be necessary in order to get a liquid film. $R\approx 5\,cm$, $V_g\approx 50 \,m \cdot s^{-1} $, gas is CO2 and liquid is water with dissolved CO2.