Annihilation operator in harmonic oscillator

Question

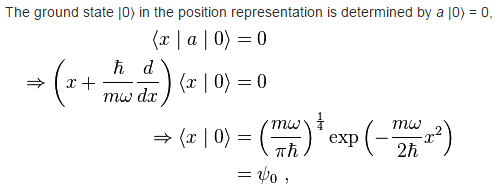
In Wikipedia's QHO page there is a moment when the following is stated:

I don't know why "the ground state in the position representation is determined by $a|0\rangle=0$". I would say that the position representation of the ground state is rather $\langle x|0\rangle$, isn't it?

However, there are other things that I'm not being able to understand about this procedure:

• Why $\langle x|a|0\rangle=0$? I thought that the annihilation operator couldn't be applied to the ground state. Does it return a $0$ if one does that?
• Is it possible to get operators out of a bra and a ket? I mean, for any operator $\hat{A}$, is $\langle\phi|\hat{A}|\psi\rangle=\hat{A}\langle\phi|\psi\rangle$ true? In the first case I would be doing the inner product between a bra ($\langle\phi|$) and a ket ($\hat{A}|\psi\rangle$), but in the second case I'm applying the operator to a constant. So... that doesn't seem right to me, but I'd appreciate it if you told me.

• Related to the last item: what happens when an operator is applied to a constant? Do I get another operator?

• How does it jump from the second line to the third one (I mean from the one with the derivative in it to the one with the $\exp$ function)? I have absolutely no idea about that.

Answer 1

1. The annihilation operator is a linear operator. A linear operator can be applied to ANY state. And yes, it returns zero when applied to the ground state.

2. You can really take this as a definition. The definition of the momentum operator $\hat{p}$ is the operator such that $\langle x|\hat{p}|\psi\rangle=-i\hbar \psi'(x)$. One could write this as $\langle x|\hat{p}|\psi\rangle=-i\hbar \frac{d}{dx}\langle x| \psi\rangle$. "x" isn't a constant here, so you're not applying an operator to a constant.

3. Physicists love to abuse notation. If you formalize it, you can't apply operators (like $\hat{p}$ and $\hat{x}$ and $\hat{a}$ and $\hat{a}^\dagger$) to constants, you can only apply them to elements in your Hilbert space.

4. The jump from the second line to the third line is made by actually writing out the differential equation $\psi_0'(x)=x \alpha \psi_0(x)$ and solving it, by whatever method you want. (I prefer "by observation" :)

The phrasing of "the ground state in the position representation is determined by ..." is a bit weird, I agree. What is really meant is that the equation $a|0\rangle=0$, when written in the position basis, gives rise to a first order ordinary differential equation which can be solved pretty easily.

Answer 2

Concerning point 2:

Operators do not always come through each other cleanly, but there are some very basic rules that always apply, which can be turned into less tedious rules that apply in special cases. Often the latter are taught first, causing mass confusion.

General rule: Operators can be expressed as

(Sum over a in the set of eigenvectors ) |a > eigenvalue(a) < a|

If there are an infinite number of eigenvectors, then the eigenvalue should have a differential quantity in it like 'dx'. Example:

x operator = integral over x: |x > x dx < x|

If you're working in some other basis you can instead express it as (Sum over vectors a, b) |a > matrix-element[a, b] < b|

Also, always put all these integrals all the way out front. So,

< x | p |Ψ> = (Integral over p) < x|p > p dp < p | Ψ>

You are free to reorder < x|p >, p, dp, and < p|Ψ > as you wish since the two brackets are just scalars, and p isn't an operator, just a point in momentum space, and dp is a differential quantity of momentum space. If you have more than one vector quantity, then you're restricted in moving those vectors around in the same fashion as you would be in regular linear algebra (if you reorder a cross-product you need to negate it, for example). Just, things of the form < label 1|label 2 > are scalars.

Getting back to this particular case, < x|p > = (a const depending on the dimensionality) e^{i*(p/ℏ)*(dot)x}. Try taking the gradient of < x | Ψ > and compare, and you'll see that it comes out so that what you said up front is right.

The same general technique can be used to find how to swap other operators around. There are generally deep reasons that they should have these particular relationships. Like, momentum-in-dimension-1 is orthogonal to position-in-dimension-2, so there aren't any interactions between these terms, so those operators can flow past each other freely. But there are relationships between p and x in the same dimension, so they have to change something on the way past.

Concerning point 3:

If a and c are states and B is a scalar operator, then

< a| B |c > is a scalar (see above)

B < a|c > is an operator. This operator is equal to B times the scalar, < a|c >. This expression does not involve applying the operator to anything. It just gets scaled.