I am a bit confused about these concepts- when is electric field or electric potential constant so i would appreciate if anyone could brief me about these things as well. Here i am referring to conservative electrostatic fields.
$\begingroup$
$\endgroup$
2
-
4$\begingroup$ if the potential is constant the electric field is zero... $\endgroup$– AccidentalFourierTransformCommented Apr 9, 2016 at 15:25
-
$\begingroup$ Potential is constant in the direction perpendicular to the electric field. $\endgroup$– YashasCommented Aug 13, 2017 at 15:44
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
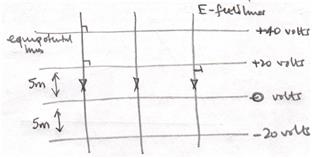
Electric field lines are always at right angles to equipotential lines or surfaces.
The electric field is minus the potential gradient.
So in the diagram showing a uniform electric field a positive charge would experience a downward force in the direction of decreasing electric potential.
In this case the magnitude of the electric field is $\frac {20}{5} = 4 $ N/C.
If the potential is constant then the electric field is zero.
If the rate of change of potential with distance is constant then the electric field strength is constant.
$\begingroup$
$\endgroup$
The electrical field is related to a force concept: it describes the force per unit charge. The potential is related to a potential energy concept: it's the added electrical potential energy per unit charge.
So, just as the force is the negative gradient (or in 1-dimension, the negative slope) of the potential energy function, the electric field is the negative gradient/slope of the potential: $$\vec{E}=-\nabla \phi,$$ where $\phi$ is the potential function due to nearby charges.
Related to your specific question, if the electric field is constant, then the slope of the potential is a constant which means that the potential is changing linearly. If the potential is constant, then the slope of the potential is zero, which means the electric field is zero. An extra charge added to an otherwise constant potential region will experience no electrical force.
$\begingroup$
$\endgroup$
When the electric potential is constant through out a given region of space the electric field is zero .Because the electric field is the force perunit positive charge. And electric potential is the potential difference. So when the electric potential is constant then there is no force acts and no change in wordone accurs so that's why the electric field is zero
$\begingroup$
$\endgroup$
It is an interesting question:
Firstly, the Electric field "$E$" is the slope of the potential, i.e., $E=-{\frac{dV}{dx}}$. Therefore "constant electric field" means the potential is either increasing or decreasing at a constant rate (along the space).
Secondly, $E$ is a physically measurable quantity but $V$ is not. You can never know the absolute value of potential $V$, although you know the value of $E$ which is constant in your case. It is because,
$V=-\int Edx$ $=$$-Ex+C$
Note that we have an integrating constant "$C$", i.e., you can always add any constant value with your solution for the potential. It further means $V$ must be measured with respect to some reference and the measured value always depends on that reference.
An example: Let's consider a parallel plate capacitor in which Potential of one plate is $V_1$ and another plate is $V_2$ and they are located at position $x_1$ and $x_2$ respectively. Also let the distance between two plates, $dx=x_2-x_1$=2cm, and electric field (which is ideally constant in such capacitor) $E=5V/cm$.
What is the value of potential that satisfies the case? Lets check,
if $V_1=0V$ and $V_2=10V$ then $E={\frac{dV}{dx}}={\frac{V_2-V_1}{dx}}={\frac{10-0}{2}}=5V/cm$
Now lets add some constant value with the potentials. i.e.,
if $V_1=5V$ and $V_2=15V$ then $E={\frac{V_2-V_1}{dx}}={\frac{15-5}{2}}=5V/cm$
if $V_1=10V$ and $V_2=20V$ then $E={\frac{V_2-V_1}{dx}}={\frac{20-10}{2}}=5V/cm$
See!!!! All of those set of ($V_1$,$V_2$) is giving the same "Constant" electric field. Therefore you can know the potential of one plate with respect to the potential of another, but can never know the absolute value of both.
This is true not only for capacitor but also for every other cases. At this point the potential of "Earth" is usually taken to be the reference in order to measure the potential at any point. It is because earth is a very large object and it's absolute potential does not get affected by any of our action.
