Dynamics question. Please help, exam coming soon [closed]

Question

I'm trying to solve this elementary problem. I'm studying mathematics, but there is a compulsory course in physics that has to be passed. I'm having an exam in 5 days and I have some doubts on problems like this:

In the above system, both 1 and 2 have the same mass. 2 has an initial velocity of $2 \frac m s$ (meters over second)

$(a)$ Graph the free body diagram of each block. Indicate it's reaction-action pair.

$(b)$ Calculate the acceleration of 1 and describe analytically and graphically it's movement as a function of time.

$(a)$ isn't too difficult:

What is getting me a little troubled is $(b)$. My professor says we should take a "curved" $x$-axis in following the rope. I suppose then I take the $y$-axis as perpendicular to the $x$-axis.

I decompose the problematic $\vec w$ vectors via the angles given. I use $\sin 37º \approx 0,6$ and $\cos 53º \approx 0,8$.

EDIT: The normal vectors are wrong. They should be.

$$\eqalign{ & \vec N = mg\sin 37\hat j \cr & {{\vec N}_0} = - mg\sin 37\hat j \cr & \vec N' = - mg\sin 37\hat j \cr & \vec N{'_0} = mg\sin 37\hat j \cr} $$ (there is a $-$ missing in $\vec w$, before $\cos 37$ which should be $i$, not $j$.)

Now I apply Newton's law, $\sum \vec F = m \cdot \vec a $, to my system. I'm interested only in $x$, so I have to use $\sum \vec F_x = m \cdot \vec a_x $. Since the conditions are ideal, $a$ is constant in all the system, $t$ is also constant. The equations are:

$$\eqalign{ & t - \left| w \right|\cos 37 = {m_1}a \cr & \left| w \right|\sin 37 - t = {m_2}a \cr} $$

Now $|w| = m_2 g=m_1g$, so

$$\eqalign{ & t - m \cdot g \cdot \cos 37 = m \cdot a \cr & m \cdot g \cdot \sin 37 - t = m \cdot a \cr} $$

So I find $a=-0.1 g\approx 1 \frac{m} {s^2}$

This means the acceleration of $1$ is in the positive direction of the $x$ axis. Is this correct? How can I move on with the problem?

Answer 1

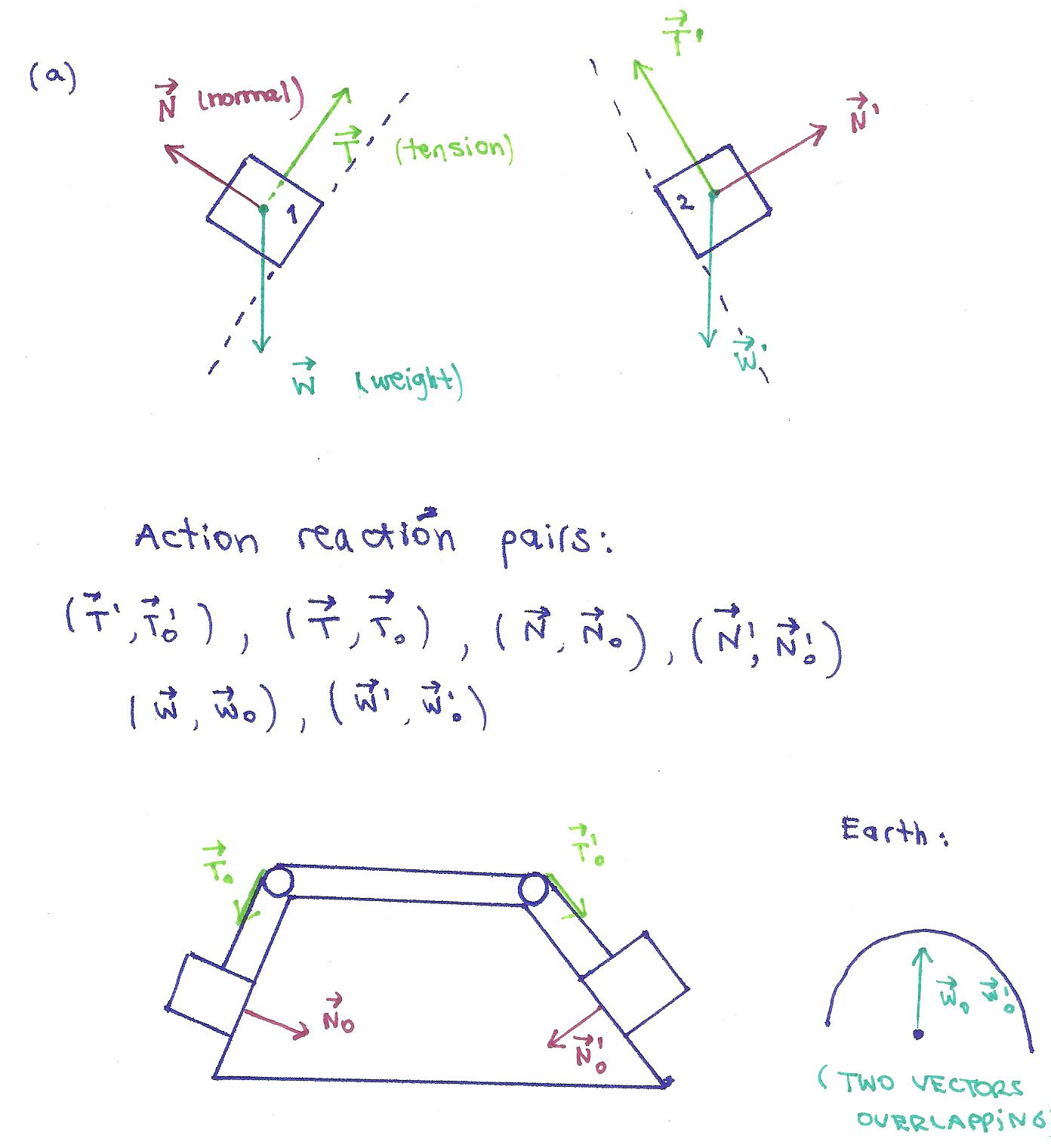
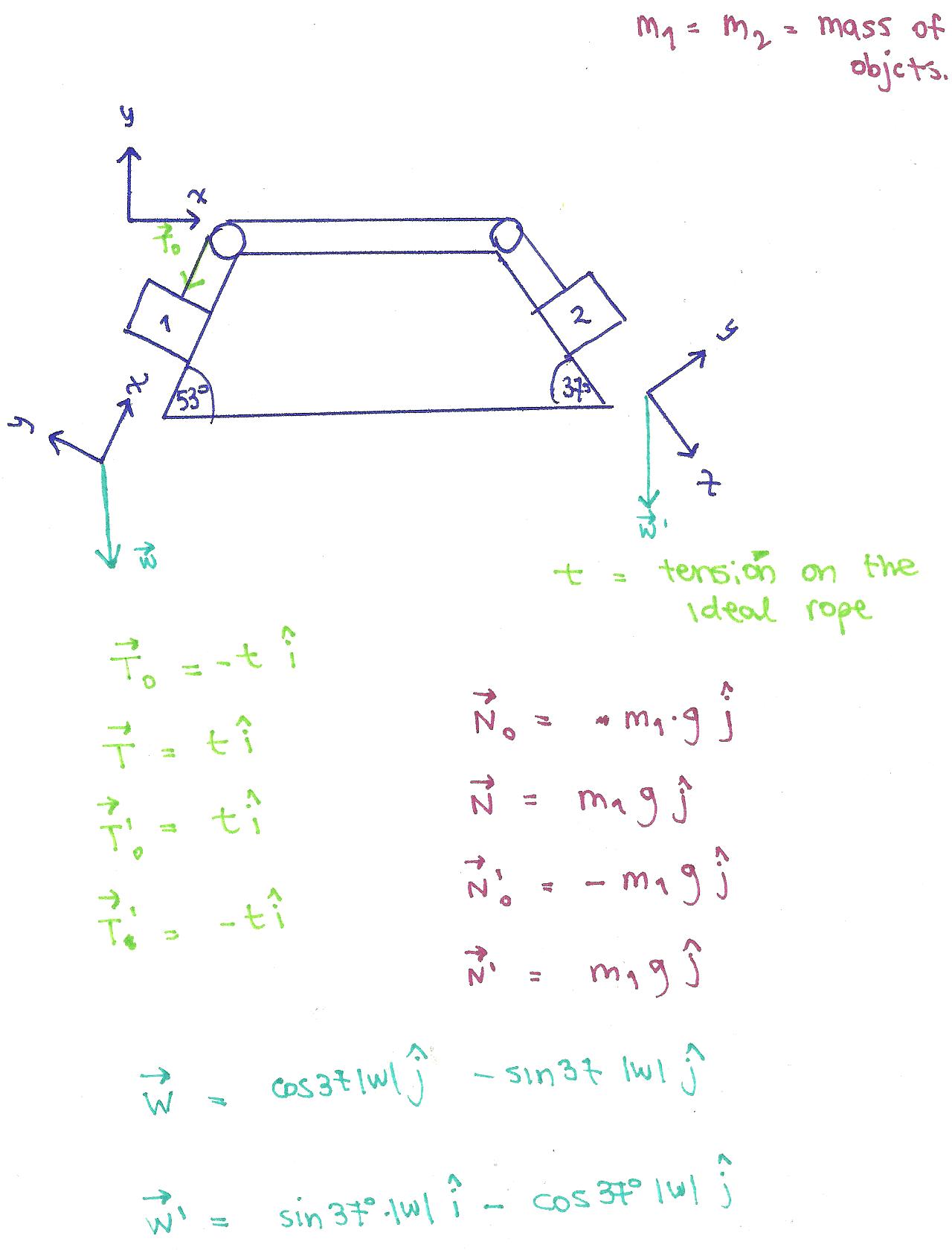
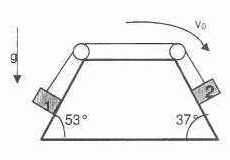
The curving x-axis is a trick in the context of mechanics--- you use the forces in the direction of the curving x-axis, in this case

$$F= m_1 g \sin(53) - m_2 g \sin(37)$$

(you can determine that it's sin and not cosine quickly in a test situation by thinking about what happens when the angle is small. If the angle-factor goes to zero, it's sine, if it goes to 1, it's cosine--- but beware of the occasional tangent!). This force is the remaining component of gravity along the inclines, and this gives you the acceleration:

$$ a={F\over m_1+m_2}$$

This solves your problem, by plugging in $m_1=1,m_2=2$. This simple thing is the result of the complicated combination of the normal forces, the pully forces, and the gravitational forces, and it is not 100% clear why this works in Newtonian mechanics (although it is true when you solve it)

The reason you have such a simple result is because of Hamiltonian/Lagrangian mechanics, which is indispensable for revealing the mathematical structure of problems like this, and justify heuristics like a "curving x-axis" which are really unjustifiable in other ways.

Hamiltonian/Lagrangian mechanics says that when you have a conservative mechanical system (if energy is conserved), the energy on the constrained surface of allowed motions determines the dynamics just the same as Newton's laws, except without having to deal with the constraint forces. The constrained motion in this case has one parameter, x, which is the position of either block relative to some starting point (their displacements are constrained to be the same). The total kinetic energy is

$$ {m_1\over 2} \dot{x}^2 + {m_2\over 2}\dot{x}^2$$

Where $\dot{x}$ is the rate of change of x, which is the speed of either block. The potential energy is, up to an additive constant, the amount of height gained/lost by the masses at displacement x:

$$ m_1 g x sin(57)x - m_2 g x sin(37) $$

which is the sum of $mg$ times the height for each mass. The resulting energy function is

$$ E = {m_1 + m_2\over 2} \dot{x}^2 + (m_1 g \sin(57) - m_2 g \sin(37)) x $$

Which is exactly the same as a one dimensional mass of size $m_1+m_2$ respoding to a constant force of the magnitude given by the formula for F. In the Lagrangian formulation, you subtract the PE from the KE, and write in terms of the velocity. In the Hamiltonian formulation, you add the PE and KE, but you use the momentum as the fundamental variables. In this case, the momentum corresponding to x coming from the Lagrangian is $(m_1+m_2)\dot{x}$

The Lagrangian method (method of "curved x-axis") becomes even more elegant when you want to include the rotational inertia of the pullies. If you always have stick-conditions on the pullies, the system is still conservative and Lagrangian, and there is additional kinetic energies from the rotation of the pullies, which is

$$ {I\over 2} \omega^2 $$

Where $\omega = {\dot{x}}/R$ and the moment of inertia of the pully is either given or calculated from the mass distribution. If you add this to the Lagrangian or Hamiltonian energy function, you get an increase in the effective mass, and that's it.

Further, if you have a massive rope, you get an additional potential contribution which is a little complicated, but just gives a force eitehr steadily increasing or decreasing with x (an upside-down spring). You can solve the problem exactly for this case too.

The Lagrangian method is not usually taught to elementary students, but it is so convenient, that professors introduce the "short-cut" of a curving x-axis instead. I would recommend that you write down the energy function and derive the effective mass and effective force from this, since this is the most fundamental description anyway.