2
$\begingroup$

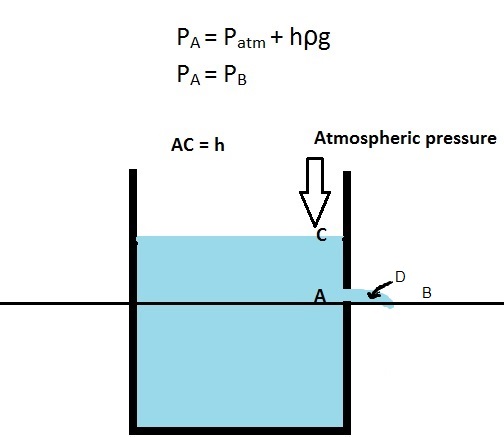
My question is similar to this one : Should the liquid come out of the tank if a hole is drilled in the vertical wall? , but I still don't understand why the pressure within a free jet of liquid/fluid leaving an open vessel is equal to atmospheric pressure.

Let's take the same example as in the previous post.

enter image description here

Indeed, hydrostatic law says that pressure within the same fluid at the same height (horizontal level) doesn't change.

The argument, in the previous post, was that point $B$ is in the open air, another fluid, so $p_B$ (the pressure at point $B$) is equal to atmospheric pressure and hydrostatic law doesn't apply to that point. I'm ok with this explanation.

However, if we check the pressure $p_D$ inside of the liquid, at point $D$, we should have the same pressure as $p_A$, at point $A$. The previous argument doesn't hold : we are in the same fluid and we are at the same height. So why is $p_D$ (roughly) equal to atmospheric pressure ?

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ Because if there is a pressure at D greater than the surrounding atmosphere, the liquid is free to move laterally to reduce that pressure. $\endgroup$
    – Jon Custer
    Commented Apr 7, 2016 at 20:14
  • $\begingroup$ The fluid is accelerated as it leaves the hole. The only thing that can accelerate a fluid is a difference in pressure. Inside the tank, there is pressure due to the height of fluid above the hole (+ atmospheric pressure). Outside the tank, the pressure is just atmospheric. That is a pressure difference. It makes the fluid accelerate. Whenever fluid accelerates, you will find a pressure difference. (That's Bernoulli, by the way.) $\endgroup$
    – Mike Dunlavey
    Commented Apr 8, 2016 at 0:31
  • 1
    $\begingroup$ @MikeDunlavey The fluid is not accelerated as it leaves the hole. It is accelerated as it approaches the hole (inside the tank). There is a pressure gradient approaching the hole inside the tank. See my submitted answer. $\endgroup$
    – Chet Miller
    Commented Apr 8, 2016 at 11:30

1 Answer 1

Reset to default
7
$\begingroup$

The pressure at D is not significantly greater than the surrounding atmosphere. The jet of fluid is surrounded by the atmosphere, and the pressure within the jet (like at point D) is virtually identical to atmospheric pressure.

What you are missing is what is happening inside the tank in the immediate vicinity of the exit hole. The hydrostatic pressure distribution inside the tank is disturbed by the fluid flow converging toward the exit hole. The effective region where this disturbance occurs is within just a few hole diameters of the exit. Thus, the point A in the figure is just about close enough to the exit hole for this disturbance to begin to be felt. In the region near the exit hole, the pressure rapidly decreases from the hydrostatic pressure variation away from the hole to atmospheric pressure at the exit hole. This localized pressure gradient provides the driving force for the flow out the hole. The surfaces of constant pressure are roughly hemispherical, with their center at the hole. The streamlines near the hole are all converging radially toward the hole. As the flow converges toward the hole, the fluid velocity increases, and the pressure decreases.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.