1
$\begingroup$

$ \left( \ddot r - r\dot\varphi^2 \right) \hat{\mathbf r} + \left( r\ddot\varphi + 2\dot r \dot\varphi \right) \hat{\boldsymbol{\varphi}} \ $

I'm not convinced about the term $- r\dot\varphi^2 \hat{\mathbf r} $, in particular about its orientation. On textbook it is called the centripetal acceleration. But, from the definition, the centripetal acceleration is orthogonal to the trajectory and this does not mean to be parallel to $\hat{\mathbf r} $. I'll make an example.

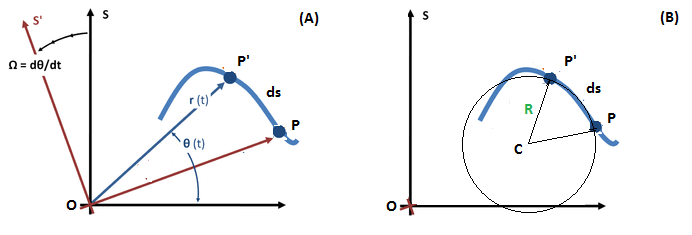
enter image description here

The same trajectory is described in polar coordinates (A) and using the osculating circle (B). Now the centripetal acceleration should be oriented as the radius of the osculating circle $R$, which is obviously not parallel to the radial unit vector $\hat{\mathbf r} $. So why is the centripetal term parallel to $\hat{\mathbf r} $ in the formula above? Am I missing something?

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ Indeed, the name "centripetal" is inappropriate for $- r\dot{\varphi}^2 \hat{\bf r}$ unless the curve is a circle. The centripetal acceleration is obtained by decomposing the total acceleration with respect to the intrinsic orthonormal frame made of the tangent, normal, and binormal unit vectors defined at each point of the trajectory. The component along the normal unit vector is the centripetal part of the acceleration, pointing towards the centre of the osculating circle. $\endgroup$
    – Valter Moretti
    Commented Apr 6, 2016 at 9:35
  • $\begingroup$ @ValterMoretti Thanks for your answer. Is a similar argument valid for the centripetal acceleration term $\vec{\omega}\times(\vec{\omega}\times \vec{r'})$ which appears for motion in rotating frames? Is that really always the centripetal acceleration or maybe just a part of it (as in the case considered in the question)? $\endgroup$
    – Sørën
    Commented Apr 11, 2016 at 16:53
  • $\begingroup$ In that case it is correct, but the rotation axis id $\vec{\omega}$ itself putting it at the origin $\vec{r}=0$. $\endgroup$
    – Valter Moretti
    Commented Apr 11, 2016 at 16:55

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.