2
$\begingroup$

Consider a vertical rod hanging from a ceiling. Neglect lateral contraction and frictional forces and air resistance and change in value of $g$ with height.

Case 1: In the absence of gravity, a rod is pulled by a force applied at the lowest point of the rod equal to its weight vertically downwards. Let the elongation be $x_1$.

Case 2: In the presence of gravity, the rod is subjected to no external forces (definitely, normal reaction of ceiling is considered) . Let its elongation be $x_2$.

Why $x_1 \ne x_2$?

Improve this question
$\endgroup$
4
  • $\begingroup$ I'll give you a hint which hopefully helps: What do you think what the streching force in the middle of the rod is (i.e. if you cut it there, what force would you need to still hold it together?) in each of these cases? Now what does this imply for the elongation? :) $\endgroup$
    – Ilja
    Commented Apr 5, 2016 at 13:07
  • 1
    $\begingroup$ Imagine that you cut each bar very close to the lowest end and 99% of the way through. Which bar do you think will break at the cut? (That is, this is a hint to help think about how the forces within each bar are different.) $\endgroup$
    – tom10
    Commented Apr 5, 2016 at 16:23
  • $\begingroup$ As I'm writing this, you have 4 close votes (with 5 needed to close it)--2 for "too broad" and 2 for "homework-like". To keep it open (which can give you further answers), as Ilja suggested, you should add what you think about it. $\endgroup$
    – Kyle Kanos
    Commented Apr 6, 2016 at 10:15
  • $\begingroup$ Related meta post. $\endgroup$
    – peterh
    Commented Aug 31, 2016 at 23:17

1 Answer 1

Reset to default
4
$\begingroup$

Suppose you have some weightless elastic strip and two weights of equal mass $M/2$. You arrange the weights in two different ways:

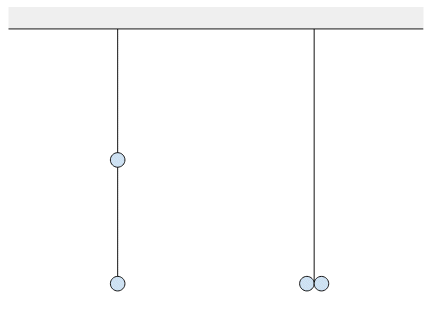
Elastic strip

In the strip on the left the tensions in the two halves of the strip are different because the upper half is stretched by both weights while the lower half is stretched by just a single weight.

In the strip on the right the whole strip has the same tension.

Since the stretching of the strips is proportional to the tension there is no reason to suppose the overall stretching of the strips will be the same, and indeed it isn't.

If we now take the strip on the left and keep halving the weights so there are four, then eight, then sixteen, and so on weights spread out evenly along the strip we approach the massive rod in your question. The massive rod is conceptually an infinite array of weights spread out along its length. By contrast the weightless rod being pulled at the end is like the strip with all the weight concentrated at the end.

It should be intuitively obvious why the extensions of the rods are different, but intuitively obvious does not constitite a proof. If I were marking this question I would expect you to derive an equation for the total stretching of the rod under its own weight. You would do this by considering the extension of a small element of length $dx$ due to the weight of the part of the rod below it. The total stretching would then be given by integrating.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.