S-matrix element

Question

I'm confused with the relation between the fully resummed propagator in a given QFT and the corresponding S-matrix element.

According to the LSZ reduction formula ($\phi^4$ theory for definiteness here), the S-matrix element for coming the simple "scattering" process:

$$ p \rightarrow q$$

can be computed as:

$$ \frac{i\sqrt{Z}}{p^{2} - m^{2}}\frac{i\sqrt{Z}}{q^{2} - m^{2}}\langle p|S|q\rangle = \int d^4y\text{ } e^{ip\cdot y}\int d^4x\text{ } e^{-iq\cdot x} \langle\Omega|T \phi(y) \phi(x)|\Omega\rangle$$

But the Fourier transformed of the 2-point correlator is just

$$\int d^4y\text{ } e^{ip\cdot y} \langle\Omega|T \phi(x) \phi(0)|\Omega\rangle = \frac{iZ}{p^2-m^2} + \text{terms regular at $p^{2} = m^{2}$}$$

Following this, for the right pole structur on both sides, the S-matrix element on the left side must be something like

$$c \cdot \delta^{(4)}(p-q) \text{ }(q^2-m^2)$$

which would vanish on-shell. But in my mind, the amplitude should just contribute to the 1 in the S-Matrix and thus just give a Kronecker delta (or just the delta function as in the above term).

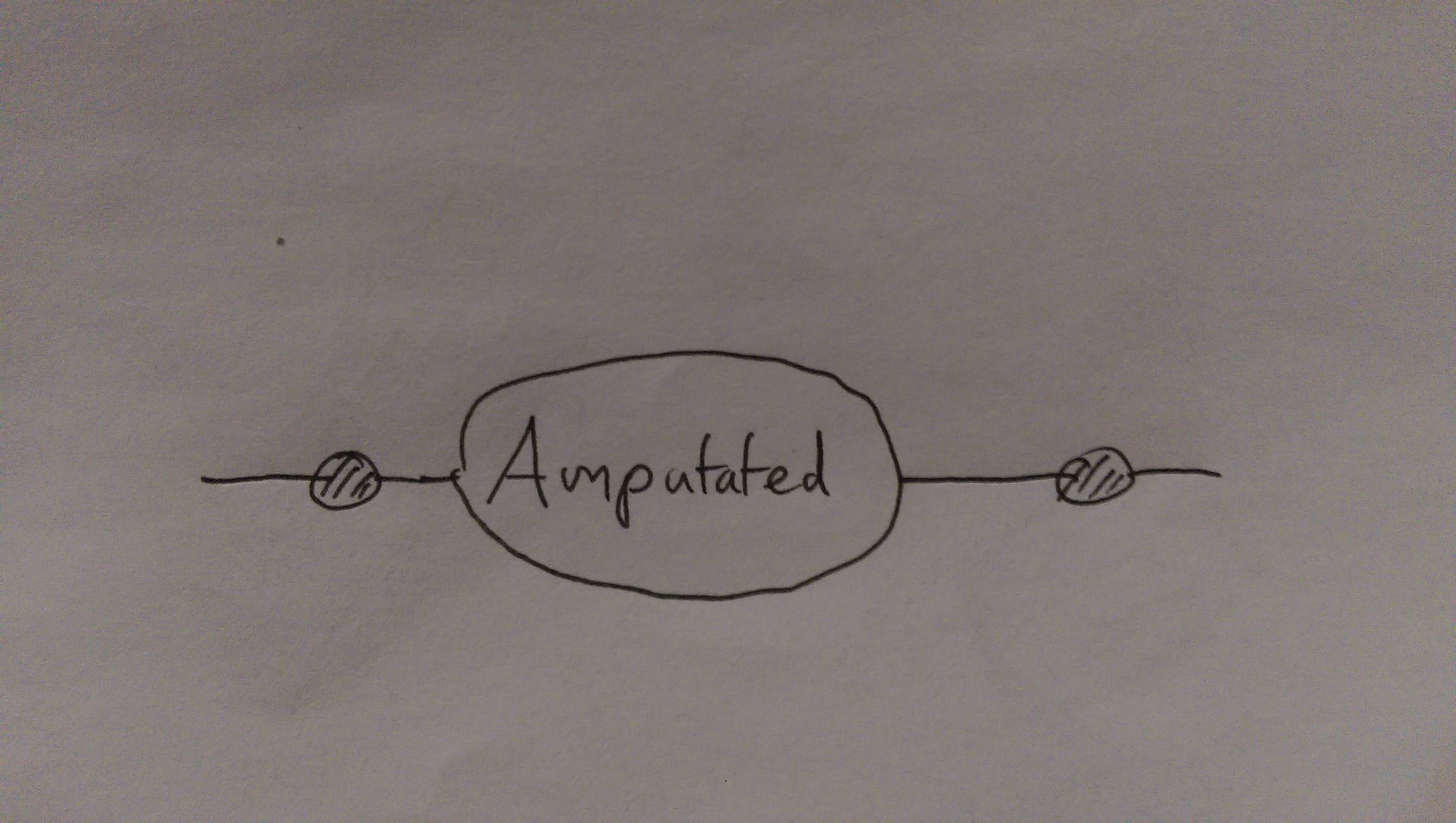
In the linked image, I visualized my problem in a diagram. There, the hatched bubbles denote the fully resummed propagator and "Amputated" denotes the S - matrix element up to an factor of $Z$, in the sense of Peskin,Schröder. I'm asking for the value of this S-matrix element or the corresponding amputated diagram.

Answer 1

There is no problem, you just have to go back to the definition of the terms in the LSZ formula to see that $\langle p \vert S \vert q \rangle\vert_\text{connected}$ is indeed just zero for the 1-particle process $p\to q$, and it is only this connected piece that appears in the LSZ formula you are trying to use to compute this amplitude. (In the notes you linked in a comment [pdf], it is chapter 2.4. where the split into connected and disconnected pieces happens.)

This is because the definition of "disconnected term" in the S-matrix is precisely "term in which nothing happens", i.e. a term that is represented by a Feynman diagram in which at least one particle just propagates without "participating in scattering". These are called "disconnected" because for all diagrams with more than 2 particles, they indeed correspond to disconnected graphs. For "2" particles, it's just a propagator, but this is a "disconnected piece" in the sense of the LSZ formula.

So there is no issue. Your reasoning is correct, the connected S-matrix is zero on-shell for this process - which is physically sensible, because the connected S-matrix entries represent interaction, something happening. A particle just propagating is not a scattering event.