The Theoretical Minimum: Confusion Over Susskind's Reasoning for mutually orthogonal states

Question

There's a better title for this question but my brain is so fried I can't come up with one.

Important Note: I am a layman, and my understanding of the mathematical concepts of quantum mechanics is very limited

In The Theoretical Minimum, pg 58, Suskind starts with this statement:

All possible spin states can be represented in a two-dimensional vector space

He then establishes a generic state $|A\rangle$ and this equation:

$$|A\rangle = \alpha_u |u\rangle + \alpha_d |d\rangle$$

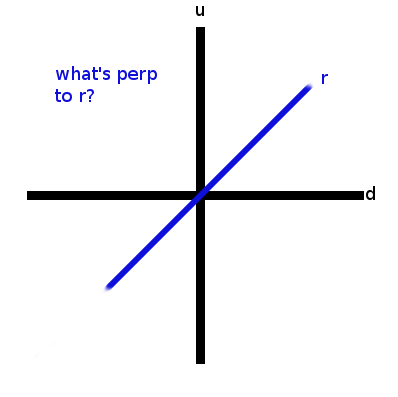
Then, on pg 61, there's this representation of a spin state prepared along $x$…i.e. $|l\rangle$ and $|r\rangle$ in terms of vectors $|u\rangle$ and $|d\rangle$

$$|r\rangle = \frac{1}{\sqrt{2}} |u\rangle + \frac{1}{\sqrt{2}} |d\rangle$$

Then the book looks at how to express $|l\rangle$ in the same way. It reminds us that

$\langle l|r\rangle = 0$ and $\langle r|l\rangle = 0$

and from that, states…as though it should be obvious…that

$$|l\rangle = \frac{1}{\sqrt{2}} |u\rangle - \frac{1}{\sqrt{2}} |d\rangle$$

Question How did he arrive at the equation for $|l\rangle$ based on the fact that $|l\rangle$ and $|r\rangle$ are mutually orthogonal?

I've tried to work this out in a couple of ways with no success. (Failed) work available by request…I'd have to re-type my written work and I'm submitting this question on a tablet. Also, this is not homework

4/5/2016: Since I'm still lost, please suggest material that covers these subjects in a way that assumes the reader failed QM 101 the first time.

UPDATE

I'm still not able to understand the proposed answers, even after reviewing the book twice and the various comments, which tells me one of two things

1. I am incapable of ever understanding this, or
2. I need a simpler explanation of these concepts.

I'm hoping its #2 so if it doesn't violate any rules, I'm updating my question to request references that could help.

Answer 1

The easiest way to see this is with Cartesian coordinates: Let $|u\rangle = (1,0)$ and $|d\rangle = (0,1)$, which are orthogonal. Then the superposition of these gives $|r\rangle = (1,1)$ for the first, and with with a minus sign in the other we have $|l\rangle = (1,-1)$ so that $$\langle l|r\rangle = (1,1)\cdot (1,-1)=1-1=0$$

You could also have $|l\rangle = (-1,1)$; and I have left our the normalization. Susskind is just more familiar with linear algebra.

Answer 2

This is a standard trick in linear algebra. If you have a vector $(a,b)$, then $(b,-a)$ is orthogonal to it (simply calculate the dot product). If we use $\{|u\rangle, |d\rangle\}$ as our basis, then we can represent $|r\rangle$ as $(1/\sqrt{2}, 1/\sqrt{2})$, so our orthogonal state $|l\rangle$ can be taken to be $(1/\sqrt{2},-1/\sqrt{2})$, that is, $|l\rangle = 1/\sqrt{2} |u\rangle - 1/\sqrt{2} |d\rangle$.

Edit: I think I see now why you are confused. There are two different vector spaces here. One is $\mathbb{R}^3$, that is, actual physical space; it is a real vector space. When we talk about spin being oriented along the $x$-axis or the $z$-axis, we mean the axes in this space. There is another vector space, called Hilbert space and usually denoted by $\mathcal{H}$, which is the space of states of our system (in this case, spin states); this one is a complex vector space. This is an abstract space, not a subset of physical, geometric space. For a spin 1/2 particle, it is two-dimensional and hence isomorphic to $\mathbb{C}^2$.

Experiment shows that for any axis in physical space, the states with spin up and down along that axis are an orthonormal basis for $\mathcal{H}$. Having fixed a $z$-axis, we call the states with spin in the $+z$ and $-z$ direction $|u\rangle$ and $|d\rangle$ respectively.

Now, when we pick a basis of $\mathcal{H}$ when can represent any ket $|\psi\rangle \in \mathcal{H}$ by a two dimensional vector: if $|\psi\rangle = a|u\rangle +b |d\rangle$, we represent it by the two-component vector $(a,b) \in \mathbb{C}^2$. When I speak of $x$-axis and $y$-axis above, I mean the axes in this abstract 2D space, not axes in physical space. Hopefully this helps.

Answer 3

You might benefit from a linear algebra refresher (e.g. from Khan academy).

Visually, the problem looks like this:

(Note that this is a 2d diagram. The $r$ line is lying flat, not pointing away.)

The apparent simplicity of the above diagram is obscured by the fact that the system these vectors are describing doubles up the angles. So you end up saying confusing things like "Up is perpendicular to Down". Also the fact that the coefficients can be complex doesn't help. Still, you should be keeping the above picture in mind when working the problem.

Anyways, on to the algebra.

---

Suppose that $|r\rangle = |u\rangle + |d\rangle$. We want to find an $|l\rangle$ that's perpendicular to $r$, but also expressed in terms of $|u\rangle$ and $|d\rangle$. (We know $|l\rangle$ must be expressible like that because we're in a two dimensional vector space and $|u\rangle$ is perpendicular to $|d\rangle$.)

So $|l\rangle = x |u\rangle + y |d\rangle$ for some unknown $x$ and $y$ satisfying $\langle r|l\rangle = 0$.

Now work it:

$\langle r|l\rangle$

$= (\langle r|) \cdot (|l\rangle)$

$= (\langle u| + \langle d|) \cdot (x |u\rangle + y |d\rangle)$

$= (\langle u|\cdot (x |u\rangle + y |d\rangle) + \langle d| \cdot (x |u\rangle + y |d\rangle))$

$= ( (x \langle u|\cdot|u\rangle + y \langle u|\cdot |d\rangle) + (x \langle d| \cdot |u\rangle + y \langle d| \cdot |d\rangle))$

$= x \langle u|u\rangle + y \langle u|d\rangle + x \langle d|u\rangle + y \langle d|d\rangle$

$= x \langle u|u\rangle + y \langle d|d\rangle$

$= x + y$

So $\langle r|l\rangle = 0$ and $\langle r|l\rangle = x + y$. Thus $x + y = 0$, meaning $y = -x$. Which tells us that $|l\rangle = x |u\rangle - x |d\rangle$ since we knew $|l\rangle = x |u\rangle + y |d\rangle$. We want a unit vector, so we set $x$ to satisfy $2xx^* = 1$ i.e. we want $|x| = \frac 1 {\sqrt 2}$. We arbitrarily pick the phase of $x$ to be 1 instead of -1 or $i$ or whatever.

And that's how you get $|l\rangle = \frac{1}{\sqrt 2} |u\rangle - \frac{1}{\sqrt 2} |d\rangle$.

Answer 4

Since the other answers don't seem to be helping, let's do it explicitly with bra-ket notation. We know that:

$$|r\rangle = \frac{1}{\sqrt{2}} |u\rangle + \frac{1}{\sqrt{2}} |d\rangle,$$

and we also know that $\langle l |r\rangle = \langle r |l\rangle =0$. Finally, we know that the state $|l\rangle$ can be expressed as a linear combination of the basis states $|u\rangle$ and $|d\rangle$:

$$|l\rangle = A |u\rangle + B |d\rangle,$$

where $A$ and $B$ are constants (suppose they're real numbers for simplicity -- and because we already know that they are from the answer). So using what we know from above:

$$\langle l|r\rangle = (A \langle u| + B \langle d|) \left( \frac{1}{\sqrt{2}} |u\rangle + \frac{1}{\sqrt{2}} |d\rangle \right)$$

$$=\frac{A}{\sqrt{2}} \langle u |u\rangle + \frac{A}{\sqrt{2}} \langle u | d\rangle + \frac{B}{\sqrt{2}} \langle d |u\rangle + \frac{B}{\sqrt{2}} \langle d | d\rangle$$

$$=\frac{A}{\sqrt{2}} (1) + \frac{A}{\sqrt{2}} (0) + \frac{B}{\sqrt{2}} (0) + \frac{B}{\sqrt{2}} (1)$$

$$=\frac{A+B}{\sqrt{2}} =0$$

The only way this can be true is if $B=-A$. So we now know that:

$$|l\rangle = A |u\rangle -A |d\rangle$$

Now we want to determine the value of $A$ so that $\langle l|l\rangle=1$:

$$\langle l|l \rangle = ( A \langle u| - A \langle d|)(A |u\rangle -A |d\rangle)$$

$$=A^2 \langle u |u\rangle - A^2 \langle u |d\rangle - A^2 \langle d |u\rangle + A^2 \langle d |d\rangle$$

$$=A^2 (1) - A^2 (0) - A^2 (0) + A^2 (1) = 2A^2 = 1$$

From this we know that $A = 1/\sqrt{2}$. Therefore:

$$|l\rangle = \frac{1}{\sqrt{2}} |u\rangle -\frac{1}{\sqrt{2}} |d\rangle$$