Interesting question.
Here's an alternative approach using a symmetrical relationship involving launch and terminal velocities that I found, which helps simplify the solution.
$\hspace{4cm}$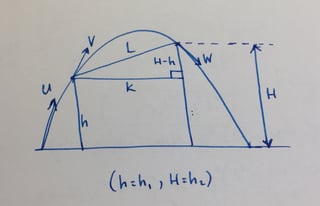
Let $u,v,w$ be velocities at launch, at the first wall and at the second wall respectively.
Let $k$ be the horizontal distance between the two walls. Hence $k^2=L^2-(h_2-h_1)^2$.
Squaring equation $(1)$ here (${v^*}{w^*}=gk$) for minimum velocities (omitting $^*$ for minimum velocities for clarity of notation) and using the standard energy conservation/kinematics formula $V^2=U^2+2AS$ gives
$$\begin{align}
v^2w^2&=g^2k^2\\
(u^2-2gh_1)(u^2-2gh_2)&=g^2 \big(L^2-(h_2-h_1)^2\big)\\
u^4-2g(h_1+h_2)u^2+4gh_1h_2+g^2(h_2-h_1)^2&=g^2L^2\\
u^4-2g(h_1+h_2)u^2+g^2(h_1+h_2)^2&=g^2L^2\\
\big(u^2-g(h_1+h_2)\big)^2&=g^2L^2\\
u^2-g(h_1+h_2)&=gL\\
\text{Minimum launch velocity, }\;\;\;\;\;\;\color{red}u&\color{red}{=\sqrt{g(h_1+h_2+L)}}
\end{align}$$
No trigonometric ratios or calculus.
Alternatively, using the results here, and omitting $^*$ for minimum velocities for clarity of notation,
$${v}^2=g(L+H-h)\qquad $$
Using conservation of energy,
$$\begin{align}
{u}^2
&={v}^2+2gh\\
&=g(L+H-h)+2gh\\
&=g(L+H+h)\\
&=g(L+h_1+h_2)\\
\color{red}u&\color{red}{=\sqrt{g(h_1+h_2+L)}}\end{align}$$
ALTERNATIVE METHOD
(Added 9 March 2021)
Let $p = h_2-h_1$.
Energy:
$$v^2-w^2=2gp\tag {1}$$
Minimal energy (see Note $1$):
$$v^2+w^2=2gL \tag {2}$$
$[(1)+(2)] \div 2:$
$$v^2=g(p+L)\tag {3}$$
Also,
$$u^2-v^2=2gh_1 \tag{4}$$
$(3)+(4):$
$$\begin{align}
u^2&=g(p+L+2h)\\
&= g(h_1+h_2+L)\\
\color{red}{u}&\color{red}{=\sqrt{g(h_1+h_2+L)}}\end{align}$$
Note 1
Let the horizontal and vertical components of $v$ and $w$ be indicated with subscripts $1$ and $2$ respectively. Note that $v_1=w_1$.
Vertical component:
$$w_2 = v_2 - gt \Longrightarrow t=\dfrac {v_2+w_2}g$$
Horizontal component:
$$k=v_1t=w_1 t =\frac {v_1+w_1}2 t=\frac {(v_1+w_2)(v_2+w_2)}{2g}$$
Pythagoras:
$$\begin{align}
L^2
&=p^2+k^2\\
&=\left(\frac {v^2-w^2}{2g}\right)^2+\left(\frac {(v_1+w_1)(v_2+w_2)}{2g}\right)^2\\
4g^2L^2
&=(v^2-w^2)^2+[(v_1+w_1)(v_2+w_2)]^2\\
&=(v^2-w^2)^2+4v^2w^2-4(v_1w_1-v_2w_2)^2 &&(*)\\
&=(v^2+w^2)^2-4(v_1 w_1 - v_2 w_2)^2\\
&\le (v^2+w^2)^2
\end{align}$$
Hence, for minimum energy,
$$v^2+w^2 = 2gL.$$
$(*)$
$$\begin{align}
[(v_1+w_1)(v_2+w_2)]^2
&=(v_1 v_2+w_1 w_2+w_1 v_2+v_1 w_2)^2\\
&=(2(v_1 w_2+v_2 w_1))^2&&(v_1=w_1)\\
&=4(v_1^2 w_2^2+v_2^2 w_1^2 +2v_1v_2w_1w_2)\\
&=4[(v_1^2+v_2^2)(w_1^2+w_2^2)-v_1^2 w_1^2-v_2^2 w_2^2+2v_1v_2w_1w_2\\
&=4v^2w^2-4(v_1 w_1 - v_2 w_2)^2\end{align}$$

