Relating Poyntings theorem to Lenz and Faraday's law?

Question

In system's similar to a motor, where the armature begins to accelerate simultaneously there is induced $-\epsilon$ to reduce the applied current(hence the applied power $P(t)$ is also reduced), or others similar to that principle such as a rail gun, Lenz's law would state the conservation of energy in Faraday's law of induction, however, how does Poyntings theorm relate to such systems? To add to the conservation of energy?

Answer 1

Definitions

First, let's start by defining some parameters:

• $\mu_{o}$ is the permeability of free space
• $\varepsilon_{o}$ is the permittivity of free space
• $\mathbf{E}$ is the 3-vector electric field
• $\mathbf{B}$ is the 3-vector magnetic field
• $\mathbf{S}$ is the 3-vector Poynting flux (also called the Poynting vector)
• $\mathbf{j}$ is the 3-vector electric current density
• $\partial_{\alpha}$ is the partial derivative with respect to parameter $\alpha$
• $q_{s}$ charge of particle species $s$
• $n_{s}$ number density of particle species $s$ (i.e., number per unit volume)
• $\mathbf{v}_{s}$ bulk flow velocity of particle species $s$

Background

Poynting's theorem is defined mathematically (in differential form) as: $$ \partial_{t} \left( w_{B} + w_{E} \right) + \nabla \cdot \mathbf{S} = - \mathbf{j} \cdot \mathbf{E} \tag{1} $$ where $\partial_{t}$ is the partial time derivative, $w_{B} = B^{2}/\left( 2 \mu_{o} \right)$, $w_{E} = \varepsilon_{o} E^{2}/2$, $\mathbf{S} = \left( \mathbf{E} \times \mathbf{B} \right)/\mu_{o}$, and $\mathbf{j} = \sum_{s} \ q_{s} \ n_{s} \mathbf{v}_{s}$. $^{\mathbf{A}}$ We can often write Poynting's theorem in differential form because the volume over which one integrates (i.e., the surface through which $\mathbf{S}$ is leaving/entering) is generally arbitrary [e.g., see pages 258-264 in Jackson [1999]].

We can define Poynting's theorem in terms of physically significant phrases, like the following:

1. the time rate of change of the energy density of the electromagnetic fields; plus
2. the rate of electromagnetic energy flux flowing out of an arbitrary surface; equals
3. the energy lost due to momentum transfer between particles and fields.

We could just as easily describe 1. as the rate of energy transfer per unit volume, 2. as the power flowing out of a volume through a defined surface, and 3. as the rate of work done per unit volume on the charges in the volume element.

One thing to note is that when in differential form as in Equation 1, Poynting's theorem is one example of a continuity equation. All continuity equations are expressed as:

1. the time rate of change of a density; plus
2. the rate of flux flowing out of an arbitrary surface; equals
3. sources and losses.

In terms of units, a flux is just a density multiplied by a velocity. In simple (loose/careless) terms, the velocity gives the direction and rate while the density supplies the volume and number.

how does Poyntings theorm relate to such systems?

Poynting's theorem is, in short, a statement of the conservation of electromagnetic energy.

The $\left( \mathbf{j} \cdot \mathbf{E} \right)$ term in Equation 1 shows how energy is transformed from electromagnetic(particle mechanical) to particle mechanical(electromagnetic) energy.$^{\mathbf{B}}$ You can see this by recalling that one form for expressing power (i.e., energy per unit time) is given by: $$ P = \mathbf{F} \cdot \mathbf{v} $$ where $\mathbf{F}$ is some force acting on some object and $\mathbf{v}$ is the velocity of said object. When you look at the $\left( \mathbf{j} \cdot \mathbf{E} \right)$ term, you can see that it can be represented by: $$ \left( \mathbf{j} \cdot \mathbf{E} \right) = \mathbf{E} \cdot \left( \sum_{s} \ q_{s} \ n_{s} \mathbf{v}_{s} \right) \\ \sim \sum_{s} \ \frac{ \mathbf{F} }{ q_{s} } \cdot \left( q_{s} \ n_{s} \mathbf{v}_{s} \right) $$ where I have just rewritten $\mathbf{E}$ as $\mathbf{F}/q$ (from the Lorentz force). Then you can see that there is a term similar to $\mathbf{F} \cdot \mathbf{v}$ within $\left( \mathbf{j} \cdot \mathbf{E} \right)$. Thus, the $\left( \mathbf{j} \cdot \mathbf{E} \right)$ term is clearly a rate of change of energy per unit volume.

To add to the conservation of energy?

Poynting's theorem is part of the total conservation of energy of a given system. There are numerous ways to treat this and many can be too involved to go into here, but the simple answer is that it defines the conversion of particle energy to/from electromagnetic energy. For instance, one can use Poynting's theorem with the generalized Ohm's law [e.g., see page 572 in Jackson [1999]] when describing the transport properties (e.g., conductivity) of a given system.

So in a sense, yes, Poynting's theorem adds to the conservation of energy in that it is one part of that law.

Side Notes

A. I already converted the expression for current density to a macroscopic form. To see more details on the difference between micro- and macroscopic Maxwell's equations, see pages 248-258 in Jackson [1999].
B. Note that I have included heat (i.e., random kinetic energy) and bulk flow kinetic energy in my use of the term mechanical energy here.

References

• J.D. Jackson, Classical Electrodynamics, Third Edition, John Wiley & Sons, Inc., New York, NY, 1999.

Answer 2

I have had an idea but I do not know whether or not my analysis is flawed?

Rather than starting by looking at a motor I want to start with a consideration of a generator.

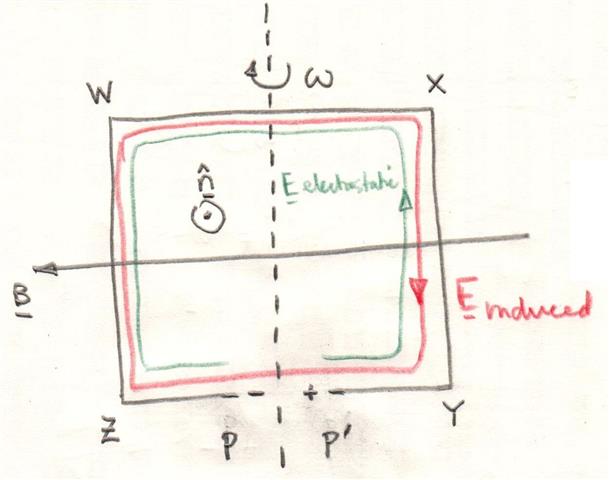
A conducting coil $WXYZ$ with a small gap in it is in the plane of the screen rotating as shown in the diagram with side $XY$ coming out of the screen and side $WZ$ going into the screen.
The unit vector $\hat n$ coming out of the screen and defines the positive direction.

Using Faraday’s law $\int_S \vec E \cdot d\vec s = - \frac {d}{dt} \iint_A \vec B \cdot d\vec a$ the induced E-field is predicted to be in a clockwise direction.
This is because $\vec B \cdot d \vec a$ is positive and the E-field line integral must be done in an anticlockwise direction when using the right hand convention.

The induced E-field moves charges in the wire which then produces an equal magnitude but opposite in direction electrostatic E-field in the coil.
Once the electrostatic E-field has been set up no current flows in the circuit and as there is no B-field resulting in the Poynting vector $\vec S$ being zero.

The integral $\int_S \vec E \cdot d\vec s$ is called the induced emf.

The electrostatic E-field has been introduced because it is a conservative field and so it can be used when applying Kirchhoff’s voltage law $\int_S \vec E \cdot d\vec s =0$.

Introducing a resistor across the gap means that a current $I$ will flow in the coil and a B-field is produced.
Note that in the diagram above this would mean that the induced current in the circuit will be in a clockwise direction.
That induced current will be producing a magnetic field inside the coil into the screen thus trying to reduce the increasing magnetic flux which is producing it – Lenz’s law.

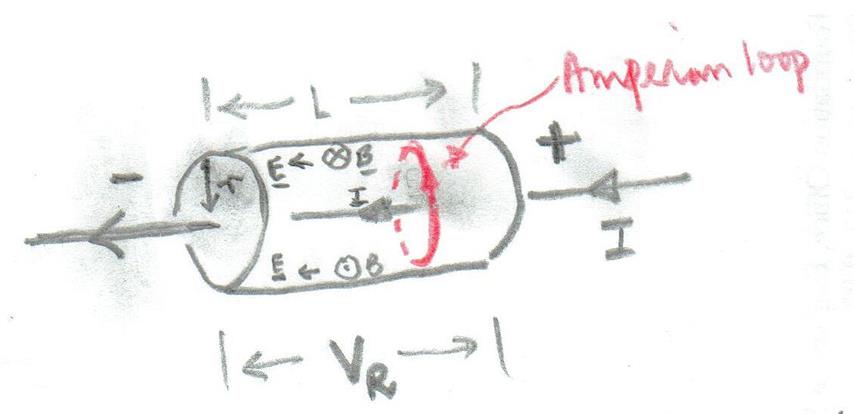
Consider the resistor as a cylinder.

Using Ampere’s law $\int_S \vec B \cdot d\vec s = \mu_o\iint_A \vec J \cdot d\vec a$ gives $B = \dfrac{\mu_oI}{2 \pi r}$.

The direction of the Poynting vector is the direction of $\vec E \times \vec B$ and for the resistor it points inwards towards the resistor.

The magnitude of the E-field is $\frac{V_R}{L}$ giving the magnitude of the Poynting vector as $\frac{V_RI}{2 \pi r L}$.

Since $2 \pi r L$ is the surface area of the resistor through which the energy is entering, the energy per second is $V_RI$ - as expected.

---

It is the next bit that I am not sure about and would value some comments about it.

For the electrostatic E-field and the associated B-field in the coil the Poynting vector points outwards.
Is this this the energy flowing out of the coil which then enters the resistor.

For the induced E-field and the associated magnetic field the Poynting vector points inwards and it always has the same magnitude as the Poynting vector associated with the electrostatic E-field.

Does the inward pointing Poynting vector associated with the induced E-field represent the flow of energy into the coil coming from the work done by the external forces making the coil rotate?

---

If what I have written above is correct then introducing a voltage source, with its positive terminal on the right hand side in the diagram above, in series with the resistor and the coil can result in one of three things happening.

The first is that current in the circuit carries on flowing in the same direction.
The analysis of the voltage source will be exactly the same as that for the resistor with the Poynting vector pointing inwards showing a power of $V_s I$ into the voltage source.

Applying KVL gives $V_B - V_S - V_R= 0 \Rightarrow V_B I = V_S I + V_R I$

Which can be interpreted as the power input to the generator $V_B I$ equals the power dissipated in the resistor and the voltage source.

The second possibility is that $V_S$ is such a value that no current flows in the circuit.

The last situation is when the voltage source $V_S$ is large enough to produce an E-field in the circuit external to it which is large to drive the current in the opposite direction.
One has gone from a generator to a motor and $V_B$ is sometimes called the back emf.

With this change of current direction the resistor has a Poynting vector inwards as does the coil but for the voltage source the Poynting vector is outwards.

Applying KVL gives $V_S - V_B – V_R = 0 \Rightarrow V_S I= V_BI +V_R i$

The power output from the voltage source equals the mechanical work done per second by the motor plus the power dissipated in the resistor.