Water molecules move faster at higher temperatures.
Does shaking a boiling kettle whilst it is in the process of boiling water increase the rate of rising temperature ?
Is it worthwhile to do so to save time ?
It's dangerous, so I wouldn't try it myself.
Yes, agitation will generally promote heat transfer and reduce heating times (although quantifying the effect is not easy). But the effect is not related to the bulk speed of the kettle.
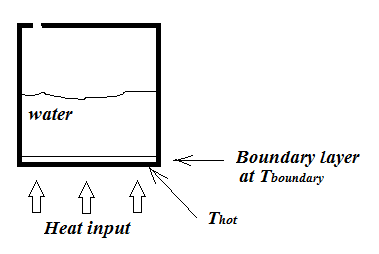
When the water is heated a diffuse (poorly defined) boundary layer is formed on the bottom of the vessel. This layer is at a temperature that is slightly higher than the bulk of the water above it.
This limits the transfer of heat from the heated surface somewhat, acc. Newton's Law of Heating/Cooling:
$$\frac{dQ}{dt}=kA(T_{hot}-T_{boundary}),$$
where $\frac{dQ}{dt}$ is the amount of heat ($\mathrm{Watt}$) transferred from the hot surface to the boundary layer per unit of time, $k$ a heat transfer coefficient, $A$ the surface area of contact, $T_{hot}$ the temperature of the bottom of the vessel and $T_{boundary}$ the temperature of the boundary layer.
But agitating effectively has the effect of reducing the boundary layer's thickness and through homogenisation probably also its temperature. The first effect actually means we're increasing the value of $k$, the effect of which on $\frac{dQ}{dt}$ is straight forward. And increased $\frac{dQ}{dt}$ lowers overall heating times.
For slightly reduced heating times, all other things being equal, stirring/shaking/agitation is beneficial (but the effect is difficult to quantitatively model).
In fluid mechanics the thickness of a boundary layer is correlated to the Reynolds number:
$$Re=\frac{vL}{\eta}$$
where $v$ is the fluid speed, $L$ is a characteristic length (like the diameter of the kettle) and $\eta$ the viscosity of the fluid. Increasing Reynolds numbers (increased fluid speed $v$, e.g.) reduces boundary layer thickness and promotes heat transfer.
As the boundary layer is essentially stationary, heat transfer across the boundary layer is essentially by conduction (Fourrier's Law}:
$$\frac{dQ}{dt} \approx \kappa A\frac{\Delta T}{\Delta x}$$
where $\Delta T$ is the temperature difference across the boundary layer, $\kappa$ the heat conductivity of water and $\Delta x$ the thickness of the boundary layer. The smaller $\Delta x$, the higher $\frac{dQ}{dt}$.
Coherent motion does not add to the temperature; so you would have to shake it violently, with random motions.
Consider sound in air - this is a coherent motion - when you can no longer make out the sounds in a closed room, the energy of the sound waves has been transformed into heat.
