What will be final velocity of three charges $q$, $q$, $2q$? [closed]

Question

What will be final velocity of three charges q, q and 2q kept along an equilateral triangle of side r at infinite distance. All three masses are equal.

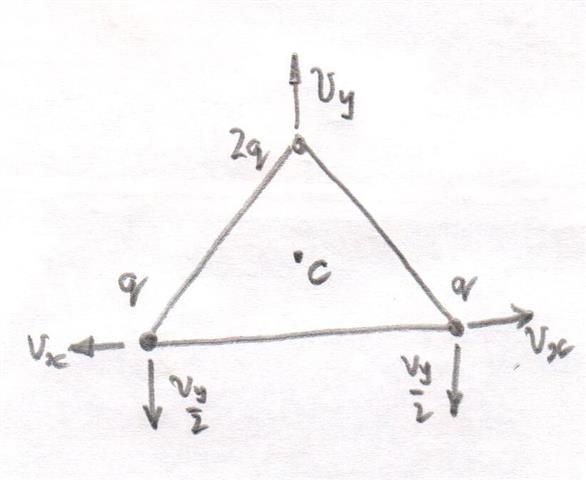I tried to conserve Total Energy

$$\frac{2kq^2}{r} + \frac{2kq^2}{r} + \frac{kq^2}{r} = \frac{mv^2}{2}+ \frac{mv^2}{2} + \frac{m(v_{2})^2}{2})$$

$$\frac{5kq^2}{r} = m(v^2 + \frac{v_{2}^2}{2}$$

$$\sqrt{\frac{10kq^2}{rm}-2v^2}= v_{2}$$

Conserving momentum gave

$$v_{2} = v\sqrt{2(1+\cos\theta)}\tag{1}$$ where $\theta$ is angle b\w velocity of q & q.

When I tried to make some graph predicting their motion and speed in different direction was like hell.

I could not get any further.

As helped by Fire I used COM along y direction.

$$my + my - my' = 0$$ $$ y' = 2y$$ $$dy'/dt = 2dy/dt $$ $$ v_{2}= 2 v_{y}$$ $$v_{2}= 2 v\cos\left(\frac{\theta}{2}\right)\tag{2}$$

I can't imagine if charges would move along straight line or in curves. Will $\theta$ change or not? Can all three have same velocity at infinity?

Someone told me it uses Taylor series.

Answer 1

It might help you to think about the symmetry of the situation.

First in the application of conservation of momentum and then what the trajectories of the charges must be to keep the centre of mass $C$ at the same position. This will give you a connection between $v_y$ and $v_x$.

Answer 2

Consider a more general case in which the three charges are $\lambda q, q$, and $q$, with $\lambda\geq 0$. Let $A$, $B$, and $C$ be the respective positions of the charges. By symmetry, we have $|AB|=|AC|$. Due to the conservation of momentum, the center of mass $O$ of the system is stationary.

Let $\theta$ be the angle $OBC$, and $\alpha$ be the angle $ABO$. Simple trigonometric manipulations give the relation

$$\tan\alpha=\frac{2\tan\theta}{3\tan^2\theta+1}.$$

Notice that $\theta=\alpha=\pi/6$ at $t=0$.

Now, consider the forces $\mathbf F_{AB}$ and $\mathbf F_{CB}$ on charge $B$ by charges $A$ and $C$, respectively. The angle $\theta$ changes when $\mathbf F_{AB} + \mathbf F_{CB}$ is not solely along $OB$. So, as $t\rightarrow\infty$, we expect $\theta$ to approach a constant value $\theta_\infty$, such that $\mathbf F_{AB} + \mathbf F_{CB}$ is solely along $OB$. That is, $\theta_\infty$ satisfies

$$F_{AB} \sin\alpha_\infty = F_{CB}\sin\theta_\infty.$$

Using the form of the Coulomb's law for $F_{AB}$ and $F_{CB}$, we find that

$$4\lambda\sin\alpha_\infty=\sin\theta_\infty(1+9\tan^2\theta_\infty),$$

which then allows us to solve for $\theta_\infty$.

Finally, with the relative positions of the charges known, the conservation of energy easily yields the final velocities of the three charges.

Lastly, for $\lambda\ll1$ and $\lambda\gg1$, we see that $\theta_\infty=0$ and $\theta_\infty=\pi/2$, respectively. For the specific case of $\lambda=2$, we can numerically obtain $\theta_\infty\approx0.657$.

Answer 3

You're only a half-step away. You listed conservation of energy and linear momentum, both of which are due to there being no external forces on the three-charge system. But with no external forces, you know that the center of mass of the system won't accelerate. Since the COM starts at rest, this means that the COM will remain stationary. Think about what this means in terms of the geometry of your problem.

(I'll help you out with this if you don't see it, but I think you will.)

Answer 4

This is actually a particular case of the three-body problem (https://en.wikipedia.org/wiki/Three-body_problem), but with repulsion, rather than attraction. There are very few exactly solvable cases of the three-body problem, among them - the Lagrange's case, where the three bodies are at the vertexes of an equilateral triangle at each moment. Therefore, I suspect you should try to prove for the initial conditions of your problem that the distances between the charges remain equal (although I cannot be sure that this is so). Some homework...