Why, here, the torque with respect to bob is zero?

Question

here, as you see, is a diagram of a pendulum with moving pivot along x-y surface.

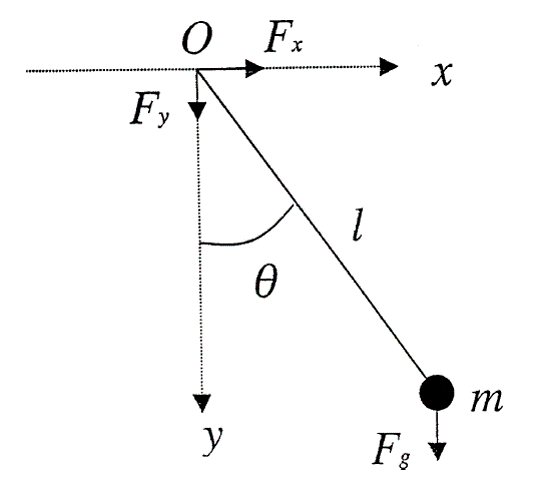
This picture was obtained from a journal in which this equation was written:

$$F_x l \cos\theta = F_y l \sin\theta $$

The explanations stated that this was because the torque of pivot with respect to the bob is zero, this is my approach:

$$\vec l= -l\sin\theta \hat i -l\cos\theta \hat j $$ $$\vec F= F_x \hat i + F_y \hat j$$ $$\vec \tau=\vec l \times \vec F = -F_y l\sin\theta \hat k + F_x l\cos\theta \hat k$$

so assuming $\vec\tau =0$ :

$$F_y l \sin\theta \hat k=F_x l\cos\theta \hat k$$

$$F_y l \sin\theta = F_x l \cos \theta$$

My question is Why can we say $\vec\tau = 0$ ?

Answer 1

$F_x$ and $F_y$ are components of a force. If the line of action of that force goes through the bob then the torque that the forces exerts about the bob is zero.
In the text those two component forces are external forces applied to the bob via a connecting wire which presumably can only transmit tensile and compressive forces ie forces along the wire.