conservation of momentum? [closed]

Question

At hyperphysics I got this image, with the same description in text as is in this image

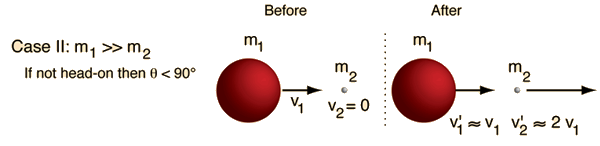
It says that when a massive particle (say $A$) moving with a velocity collides with an object having a relatively low mass (say $B$), then $B$ gains an velocity of $2v$ and the velocity of $A$ remains essentially unchanged.

Well, I agree with this fact because $A$ will create an acceleration in $B$ from a very far area, but $B$ will influence $A$ the least. So, here it appears like the law of conservation of momentum is violated? Can you please correct the fault that I have made in thinking about this phenomenon because conservation of momentum is not violated anywhere, or please just say that this source has provided the wrong information?

Answer 1

This is essentially the same question as https://astronomy.stackexchange.com/questions/13302/

In a perfectly elastic collision, both momentum and kinetic energy are conserved.

The initial momentum is $\text{m1} \text{v1}$ and the initial kinetic energy is $\frac{\text{m1} \text{v1}^2}{2}$, since m2 is at rest.

Let u1 and u2 be the velocities of the two masses after the collision. By conservation of momentum and kinetic energy, this means:

$\text{m1} \text{u1}+\text{m2} \text{u2}=\text{m1}\text{v1}$

$ \frac{\text{m1} \text{u1}^2}{2}+\frac{\text{m2} \text{u2}^2}{2}=\frac{\text{m1} \text{v1}^2}{2} $

There are two solutions to these simultaneous equations, one of which is the initial condition (u1 = v1, u2 = 0). The other is:

$ \left\{\text{u1}\to \frac{\text{v1} (\text{m1}-\text{m2})}{\text{m1}+\text{m2}},\text{u2}\to \frac{2 \text{m1} \text{v1}}{\text{m1}+\text{m2}}\right\} $

Since m2 is small, compared to m1, let's set r=m2/m1 (which we expect to be a small number) in the solution:

$ \left\{\text{u1}\to \frac{\text{v1}-r \text{v1}}{r+1},\text{u2}\to \frac{2 \text{v1}}{r+1}\right\} $

Now, as r approaches 0, we see that u1 approaches (v1-0*v1)/(0+1), or v1, and u2 approaches 2*v1/(0+1) or 2*v1