Different results for thermal resistances using different methods

Question

A system is set up which is composed of a cylinder with hemispheres on either ends. The length of the cylinder is l and inner and outer radii of cylinder and hemisphere are $R_i$ and $R_f$. The thermal conductivity of both parts are same and equal to k. The temperature inside the setup is maintained at a constant temperature of $\theta$ while the surrounding temperature is $\theta_o$.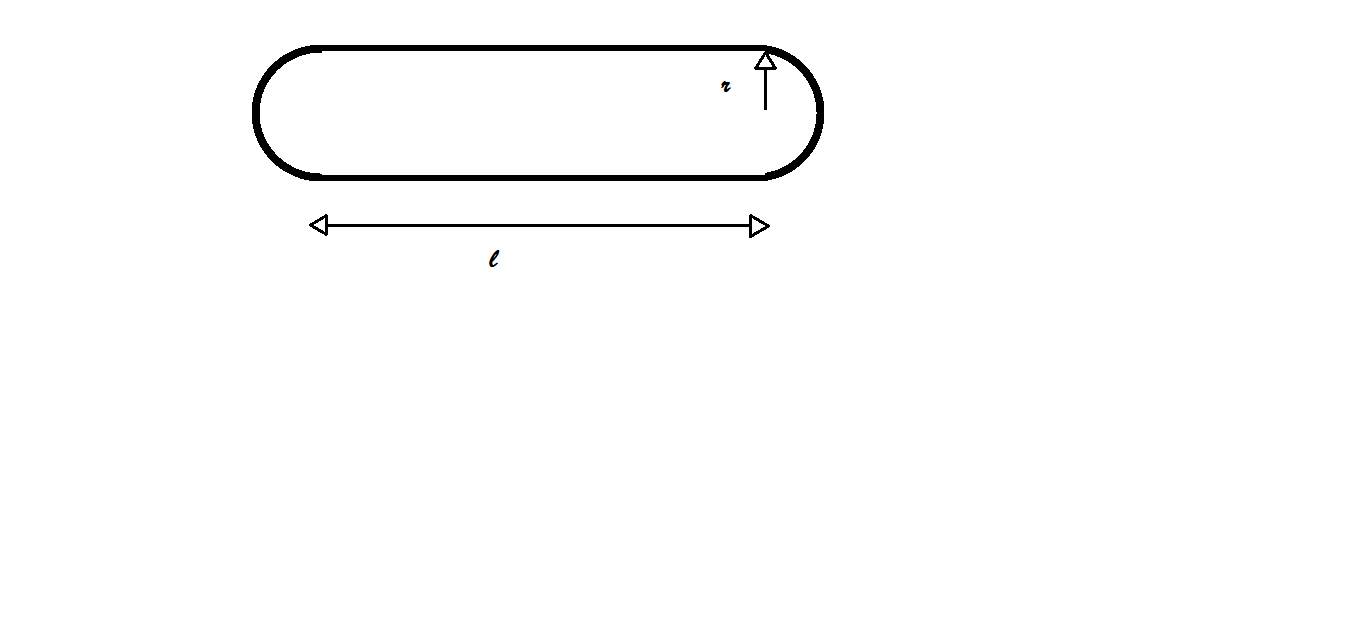

$\mathbf{Approach 1}$: Consider an infinitesimal shell at distance r from the axis of the cylinder and r distance from the center of the hemispheres. Let the thickness of the shell be $dr$.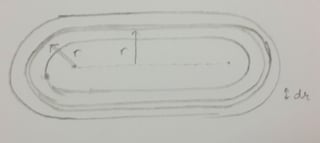

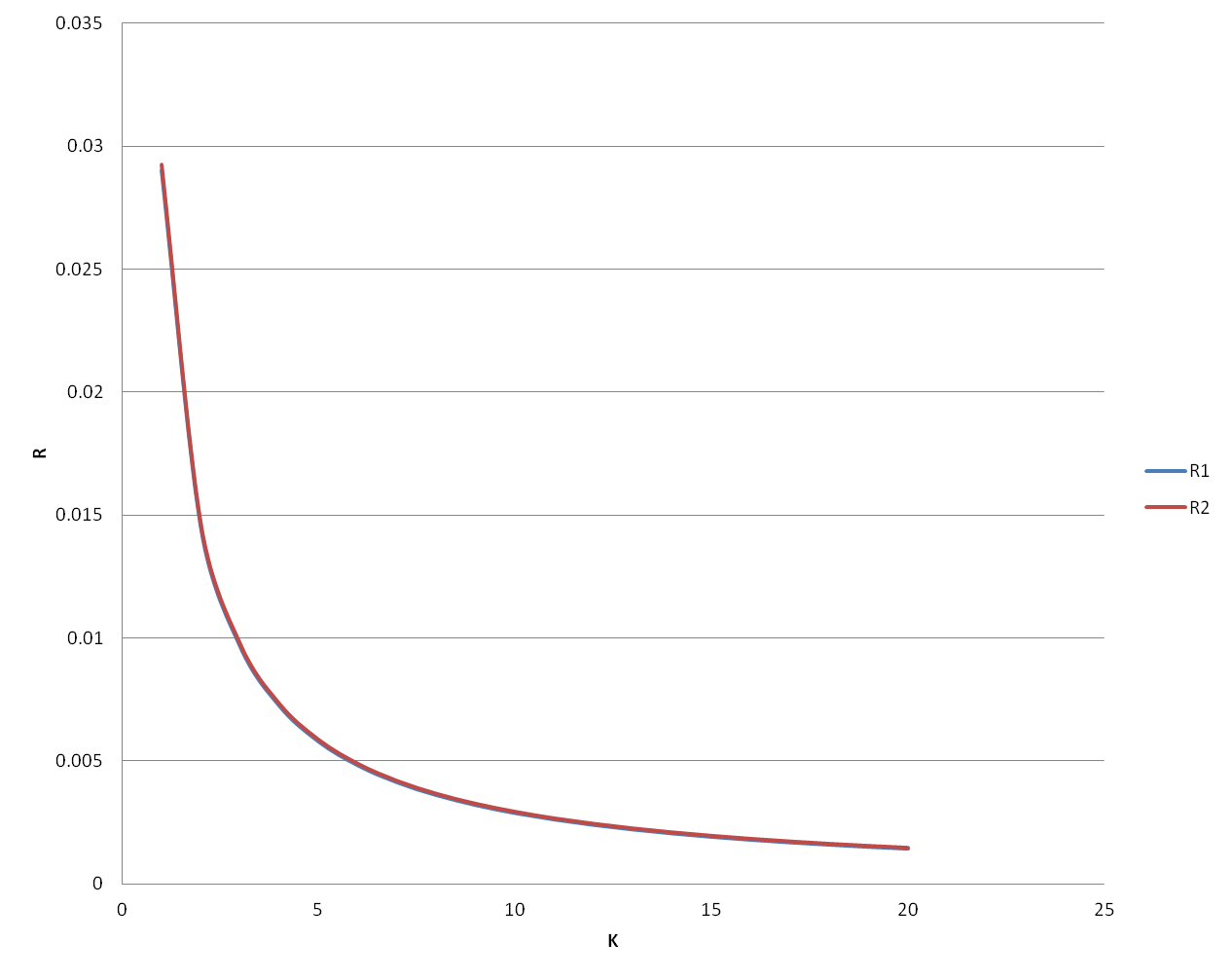
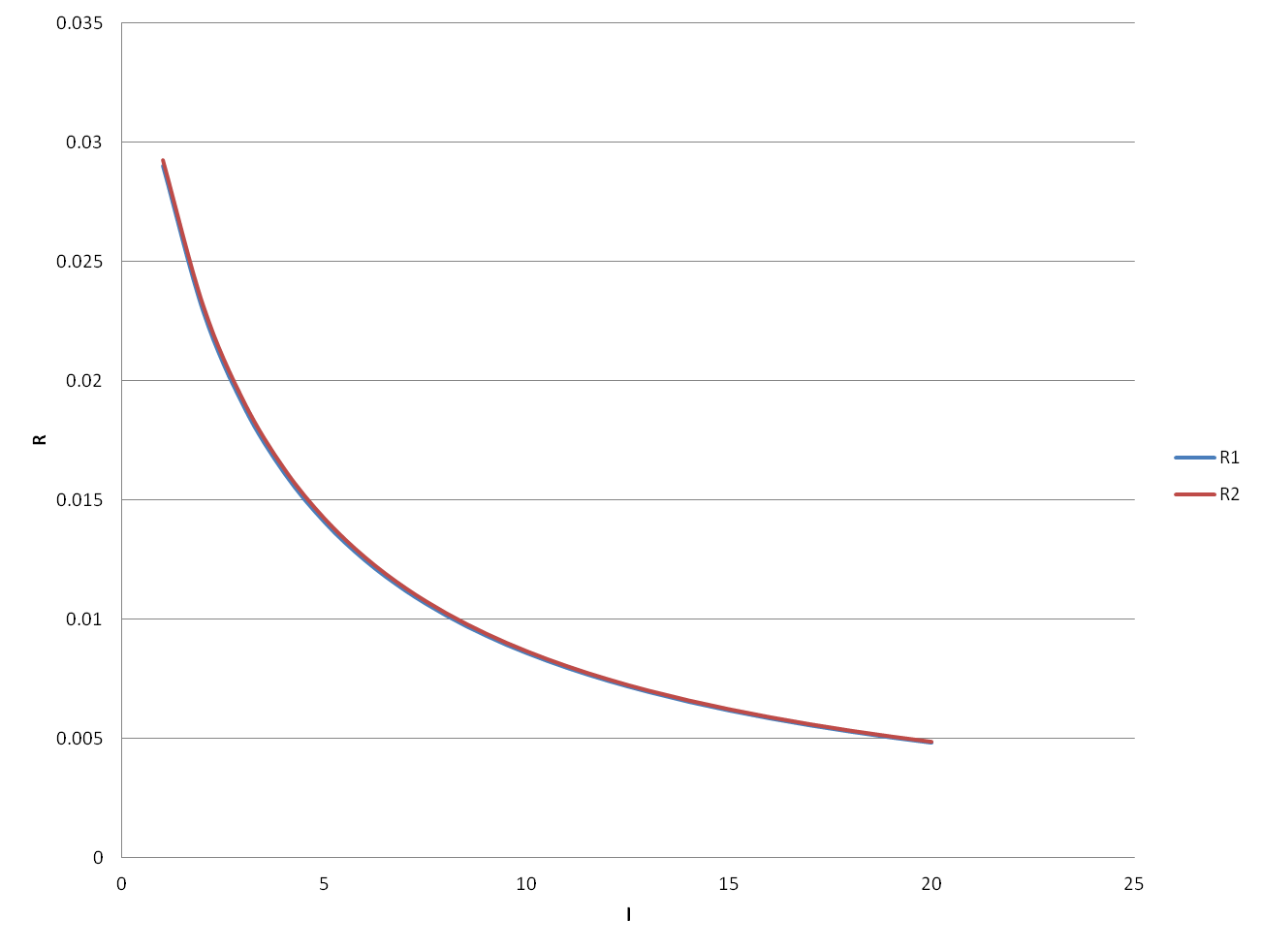
The resistance of this shell is $$dR=\frac{1}{k}\frac{dr}{2\pi rl+4\pi r^2}$$. Since all the shells are in series connection, the net resistance is $$\int dR=\int^{R_f}_{R_i}\frac{1}{k}\frac{dr}{2\pi rl+4\pi r^2}$$ which gives $ R=\frac{1}{2\pi kl}(ln\frac{R_f}{R_f+l/2}-ln\frac{R_i}{R_i+l/2})$

Now consider an alternative approach.

$\mathbf{Approach 2}$:

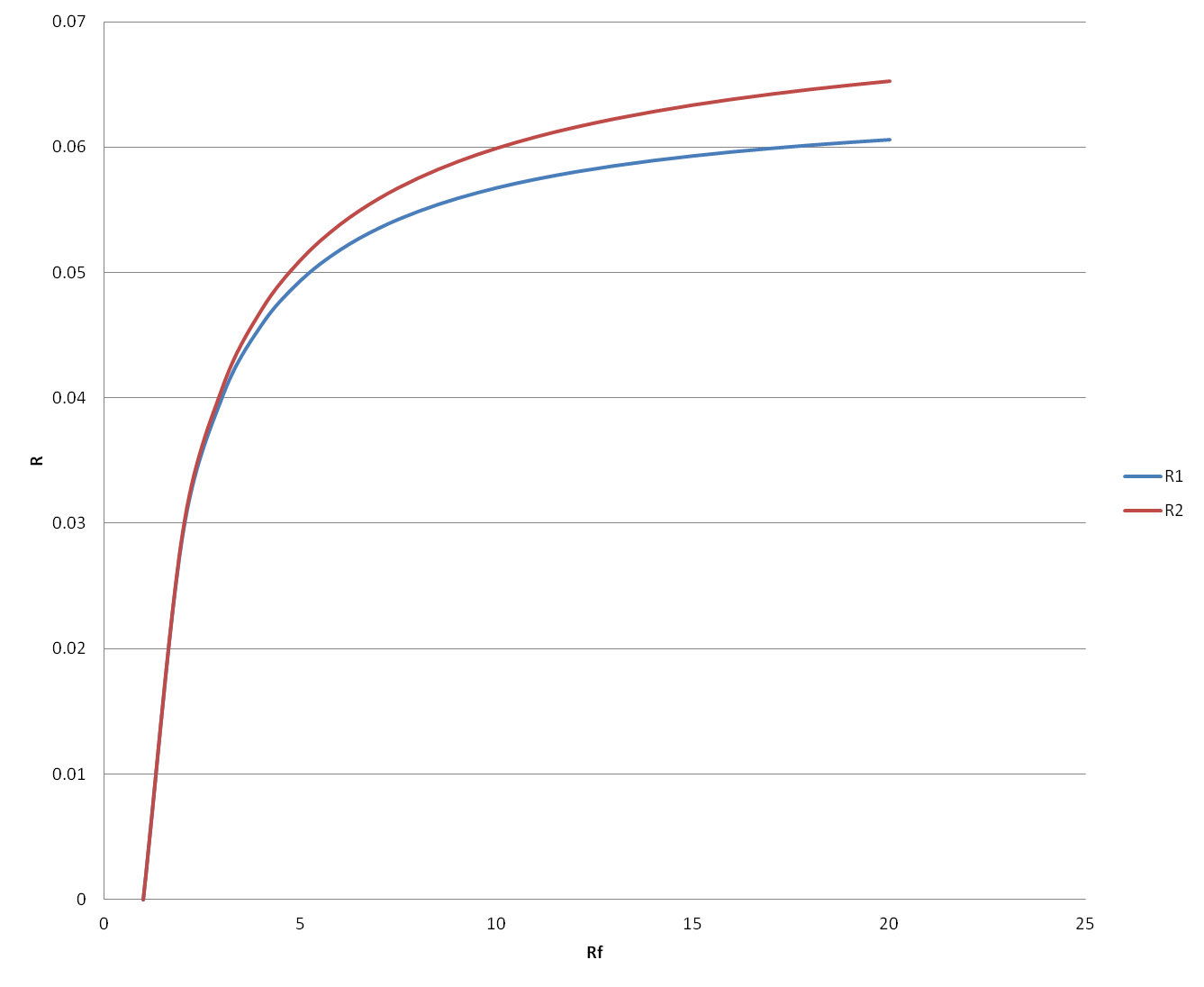
(I am not going to show the calculations for the resistance of cylinder and hemisphere; I will directly use the results.) Since the the inner parts of the cylinders and the hemispheres are at the same temperature. Similarly, the outer parts are also at the same temperature. So we can say that the cylinders and hemispheres are arranged in a parallel connection. So the equivalent resistance is given by $$\frac{1}{R_eq}=\frac{2\pi kl}{ln\frac{R_f}{R_i}}+\frac{4\pi k R_f R_i}{R_f-R_i}$$ which on solving for $R_eq$ gives a completely different result from the first one.

On observing the first approach more carefully, it is clear that the equivalent resistance for parallel combination has been used before integrating the elemental resistance. While in the second approach, the elemental resistances have already been integrated and then the formula for parallel combination has been used.

Why do these two methods give different results and which one is correct?

Answer 1

Why do these two methods give different results and which one is correct?

Both are incorrect; but the second more, I'd say. As H.Tofaili pointed out, both often are very similar, since every of them makes some approximation which is only always allowed in some limit.

• In your second approach you assume, that there is no heat conducted across the (imagined) cut between the cylinder and the hemispheres. But it will. It may be very little in most cases, but you can see it's there in an extreme case:

  take a thick spherical shell and insert a very thin cylinder (great $R_f-R_i$, small $\ell$). It is in practice still a shell and will transport heat radially. Then with $\frac{R_i}{R_f} \rightarrow 0$ arbitrarily little of the heat starting in the cylinder will end up in it, it will all cross the border.

• In your first approach you assume, that the surfaces of equal temperature are all of the same form and equally spaced. This example is not as convincing as the above (I mean, here the assumption doesn't get arbitrarily wrong), but consinder:

  Take now a thin ($R_f\approx R_i$) figure. The central parts of the cylinder and the hemispheres are far from the other form respectively, so the heat conduction in them is decoupled from each other and behaves just as in a cylinder or hemisphere. The mean temperature will be at the geometrical mean of the radii in the cylinder and at the harmonical mean of the radii in the hemisperes.

So every approximation has a limit in which it is bad. But I think, the first approximation is "better", it looks so "second-order", and as I said, I don't see how to make it arbitrarily wrong. But this get sheer intuition now...

Answer 2

These two forms look different but they are the same in fact.

You can try different values for $R_i$,$R_f$,$l$ and $k$ to see that the two answers will only differ in a very small error, coming from the calculator.

It happens many times when solving differential equations that you get a solution using a certain method, then, solving with another method you get a seemingly different solution. Nevertheless, the two solutions are the same one but in different forms.

So you need not to worry because both answers are correct.

EDIT: I tried to prove them equal but couldn't, so I plotted the two forms as functions of $R_i$,$R_f$,$l$ and $k$ using Excel.Here are the results:

You can see that the functions are equivalent to each other,maybe except for the last figure where the error is relatively great. I think you can get a more accurate result using a better program.