4
$\begingroup$

I was taught at school that the formula for period of a pendulum is $T=2\pi \sqrt{\frac{l}{g}}$ Later I found out that this is only an approximation valid for small angles and the accuracy of this formula is decreasing when you increase the angle you displace the pendulum by. So I decided to check this myself and then compare the graph from my experiment to a graph from wikipedia.

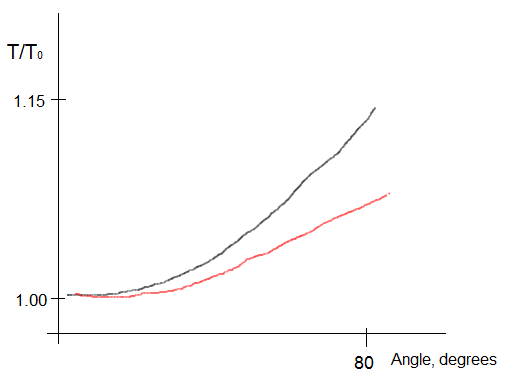
The graph below is the relationship between "real" period divided by linear period calculated with $T=2\pi \sqrt{\frac{l}{g}}$ and the angle by which you displace the pendulum. The thing is that the $T$ in the black graph is "real" period calculated in the abstence of air resistance and friction and $T$ in the red graph is what I got from my experiment which obviously involved air resistance and friction.

enter image description here

The problem is that I don't know how to explain to myself that for larger angles, the difference between the two graphs is increasing. For small angles they are similar but for larger angles they become more and more seperated. Why?

I have one hypothesis but don't know if my explanation is correct, or if it is, I don't know if it's the main factor affecting the discrepancy. It goes like that: I measured periods for $10°$, $20°$, $30°$ etc, but to be more precise, I let the oscillation for every angle be 10 periods and then I divided the time by 10 to get the value of period for a given angle. The way I would explain the discrepancy with the above information is that for large angles like $70°$ ten oscillations lasted longer and due to air resistance and friction the pendulum had more time to decrease its amplitude because it loses energy on doing work against air, etc. So even if the first of ten periods in a single measurement had an initial amplitude of $70°$, the tenth could have had an amplitude of e.g. $50°$ so the last period was smaller because the angle was smaller. Therefore, when I divided this total time by 10 I actually got a smaller value of period than I should. Check out the graphs in this answer: What is the period of a physical pendulum without using small-angle approximation? What do you think? If this reasoning is wrong, then how would you explain the discrepancy?

Improve this question
$\endgroup$
6
  • $\begingroup$ I don't know, but your analysis sounds plausible. Consider also that the drag due to air is velocity dependent. $\endgroup$
    – garyp
    Commented Dec 18, 2015 at 21:25
  • $\begingroup$ So this would also explain why the gap between the two graphs is increasing - because the force exerted by air on the pendulum is increasing with speed so air friction will play a proportionally more important role for large speeds (=large angles), right? $\endgroup$
    – user101707
    Commented Dec 18, 2015 at 21:28
  • $\begingroup$ Still waiting for a full answer if someone can help me with the explanation. :) $\endgroup$
    – user101707
    Commented Dec 18, 2015 at 21:30
  • $\begingroup$ By which formula was the black graph calculated? Is it from Wikipedia? Which page? $\endgroup$
    – Qmechanic ♦
    Commented Dec 18, 2015 at 22:20
  • $\begingroup$ @Qmechanic - upload.wikimedia.org/math/7/8/5/… from en.wikipedia.org/wiki/Pendulum_%28mathematics%29 $\endgroup$
    – user101707
    Commented Dec 18, 2015 at 22:52

1 Answer 1

Reset to default
2
$\begingroup$

If your amplitude decays significantly during the experiment, then you need to consider doing a different experiment. Possible approach: film the pendulum using slow motion mode (now available on many cell phone camera). Count the number of frames between zero crossings, and measure the angle nomadism deflection. Then you can plot one as a function of the other, normalized by the number of frames for small deflection. The data may be a little bit noisy but it will be more precise.

Alternatively, use a larger (heavy, dense) bob for your pendulum - drag goes with square of the radius but energy with the cube of the radius (mass, volume). That would require minimal change to your existing setup and possibly no new equipment (assuming you have a heavier weight lying around).

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.