When I shine a beam of light at a mirror why does the ray go in the exact opposite direction (tested with protractor). Why does it not go back in exactly the same direction? The beam is of an unspecified wavelength, it was just shone from a lightbulb in a box with a slit of a few millimeters to let a beam of light shine through.
$\begingroup$
$\endgroup$
4
-
2$\begingroup$ That is what is called reflection. $\endgroup$– SchrodingersCatCommented Dec 10, 2015 at 17:38
-
$\begingroup$ But why does the light reflect in the direction it does? Why does it not reflect right back at the source of it. All light sources in the room were turned off during the test. $\endgroup$– FeatherballCommented Dec 10, 2015 at 17:42
-
1$\begingroup$ Relevant: en.wikipedia.org/wiki/Fresnel_equations Also: en.wikipedia.org/wiki/Specular_reflection $\endgroup$– tmwilson26Commented Dec 10, 2015 at 17:44
-
$\begingroup$ What do you mean by "Why does it not reflect right back at the source of it?" Can you provide an illustration of what you mean? $\endgroup$– SchrodingersCatCommented Dec 10, 2015 at 17:45
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
One quite general argument is one of symmetries and conserved quantities.
Light does carry momentum in the direction of propagation and momentum conservation is related to a symmetry: the homogeneity of space. (This result is a special case of the Noether-Theorem).
The mirror (idealized as being infinitely large and perfectly even) can be understood as a boundary condition, that breaks continuous translation symmetry only in one direction (let's call it $y$), while the $x$ and $z$ directions continue to be translation invariant.
This tells you, that the presence of the mirror cannot change $x$ and $z$ components of the propagation direction of the light, therefore the light beam does not return to its origin, unless it is perpendicular to the mirror.
The fact that the $y$ component of the direction is exactly reversed is a bit more tricky. But if we disallow our mirror to change the energy of the photons during the reflection, then energy conservation and some basic facts from the quantum theory of light will help (energy conservation, by the way, corresponds to a symmetry as well, this symmetry is time translation). If we supposedly know that $E = \hbar k = \hbar \sqrt{k_x^2 + k_y^2 + k_z^2}$, then $E = E'$ and the fact that $k_x$ and $k_z$ do not change, constrains the reflection to the observed behaviour $k_y' = -k_y$. (Note that this energy conservation argument can also be made rigorous for a classical wave packet. In that case the formulation will, however, be much more complicated).
answered Dec 10, 2015 at 22:02
$\begingroup$
$\endgroup$
13
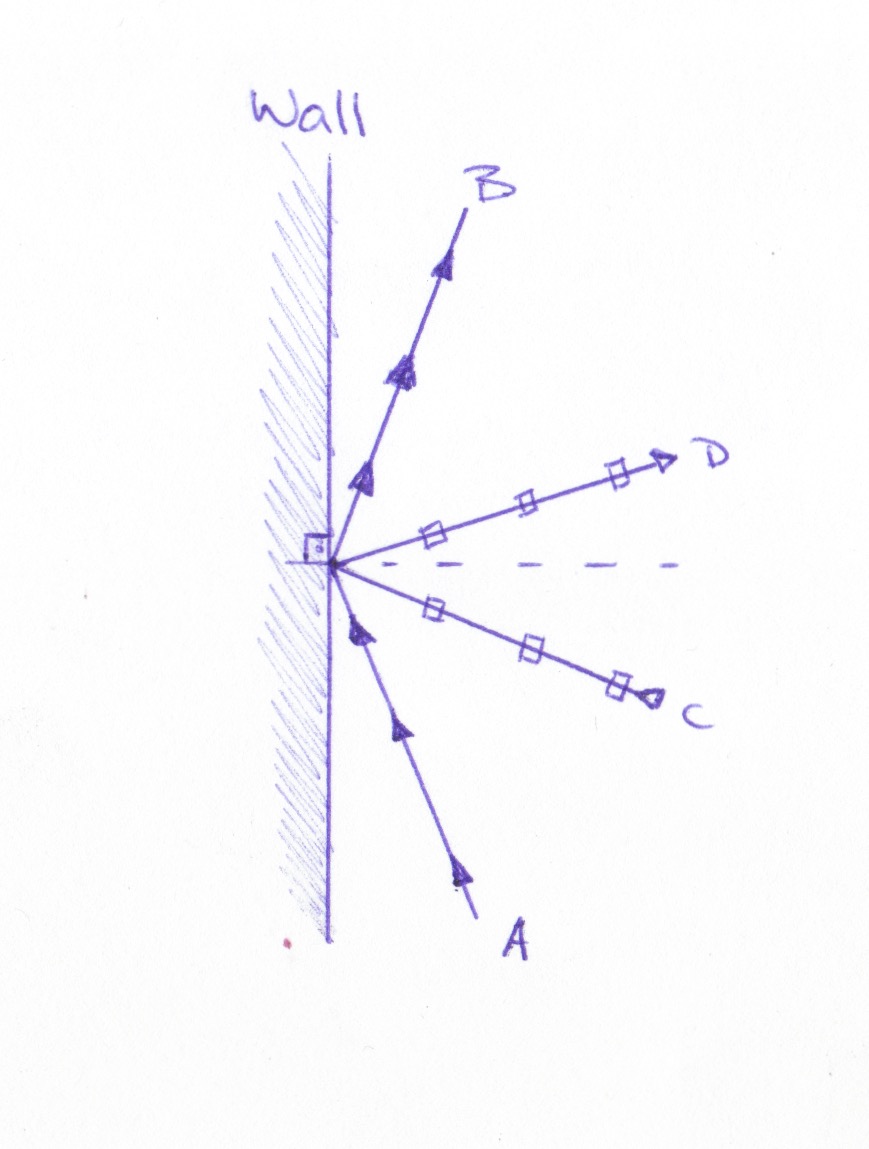
Imagine you throw a tennis ball at a wall. If you throw it perpendicular to the wall, it'll bounce right back, but if you bounce it at an angle, it will bounce exactly in the "opposite" direction (without taking friction and elasticity into account). If you are located at A and throw the ball, it will bounce off to B. If you're at C and throw it, it'll go to D. The same applies to light: if you shine a light at an angle from A, the light will reflect and go to B and so on.
Just to clarify, I say "opposite" because it is not called opposite. But I understand what you mean :)
-
-
$\begingroup$ He did ask for exactly that. If he did know this, why did he ask in the first place? @manshu $\endgroup$ Commented Dec 10, 2015 at 19:03
-
$\begingroup$ he meant why not in any other direction. Normal sized objects (like ball) have different properties than light. Then why should light be like them in this case? We know light have different properties. So we cant say light should behave like a material every time. $\endgroup$– manshuCommented Dec 10, 2015 at 19:07
-
1$\begingroup$ Yes. This conservation of momentum is the reason. Photons also have a momentum. Further information is given by quantum theory. $\endgroup$ Commented Dec 10, 2015 at 19:07
-
1$\begingroup$ @manshu Thanks, those answers helped improve my understanding of why light reflects at a certain angle, I'm going to look into this question further with my physics teacher, it might be helpful to have someone explain it to me with words rather than text. $\endgroup$ Commented Dec 14, 2015 at 17:16