1
$\begingroup$

More specifically , when we try and prove that up thrust experienced by an liquid column inside the liquid is equal to the weight of the liquid column , I have seen it being derived like this: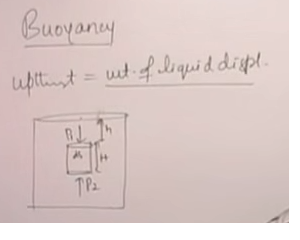enter image description here

thrust = $(P_2-P_1 )dS$ (where $P_1$ and $P_2$ are the pressures indicated in the image and $\rho$ is the density of the fluid) followed by :

enter image description here

in this entire derivation why don't we consider adding the pressure exerted by air or the weight of the liquid column to the equation of thrust ?

Improve this question
$\endgroup$
2
  • $\begingroup$ Could you please write out the equations in MathMode (use \$...\$ and \$\$...\$\$.? They are not easy to see from your photos. Also, what is $dS$ in your thrust equation? And where does the rho enter, which you mention in the text but doesn't include in the expression? $\endgroup$
    – Steeven
    Commented Nov 26, 2015 at 12:53
  • $\begingroup$ sorry about that , I'm actually still familiarizing myself with the mathmode . dS is the sectional area of the column. Also rho is used in the derivation (in its symbolic form). $\endgroup$
    – Ishita Gupta
    Commented Nov 26, 2015 at 13:14

1 Answer 1

Reset to default
4
$\begingroup$

$P_1$ and $P_2$ are defined in terms of a reference pressure and a contribution due to the weight of the liquid column: $$P_1= P_0 + \rho g h_1 \quad P_2=P_0+\rho g h_2$$

Note that the $\rho g h = \rho g V/A = F/A$ is the pressure exerted by the weight of the liquid column. Taking the difference $P_1-P_2=\rho g (h_1-h_2)$ cancels the reference pressure (which could be atmospheric or otherwise).

That is why the pressure exerted by air is not included.

Improve this answer
$\endgroup$
2
  • $\begingroup$ ok I think I understand now . However lets say that the body we were talking about link had once face directly facing the air .Will we still not consider air pressure ? why should buoyancy be defined in this case since there is no P1 (according to the derivation ) ? $\endgroup$
    – Ishita Gupta
    Commented Nov 26, 2015 at 13:20
  • $\begingroup$ @IshitaGupta - There is a $P_1$; in that case $h_1=0$ and the pressure $P_1=P_0$ is atmospheric. Unless your in a perfect vaccuum there is always a pressure to be considered. The buoyancy force comes from the fact that the object experience forces at its surfaces which want to push it in the direction of decreasing pressure. Whether the object moves or not depends on the balance between its weight in the fluid vs the bouyancy force it experiences. $\endgroup$
    – nluigi
    Commented Nov 26, 2015 at 13:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.