10
$\begingroup$

Let's consider an excited nucleus emitting one gamma-ray (not cascade etc). Is the direction of gamma-ray emission completely random? In other words, is the probability to detect this gamma equal for any angle?

Will the answer change in case of presence of magnetic field?

Improve this question
$\endgroup$
1
  • $\begingroup$ Trivially speaking, if we view the same event in two reference frames moving at a constant relative speed, it can't be a uniform angular distribution in both frames. I assume you're wondering about the angle in the reference frame of the nucleus before the gamma-ray was emitted? (Conservation of impulse means that the nucleus will change speed when emitting a photon) $\endgroup$
    – MSalters
    Commented Nov 9, 2015 at 12:45

3 Answers 3

Reset to default
14
$\begingroup$

1) To define an angle for a nuclear reaction one has to have an orientation for the nucleus. This could be a magnetic moment or a dipole moment.

2) a photon carries away spin 1, i.e. angular momentum, and will leave a nucleus minus that angular momentum. As the word states, angles are involved, and the probability distribution for the gamma ray will in general not be uniform. This can be calculated given the initial state:

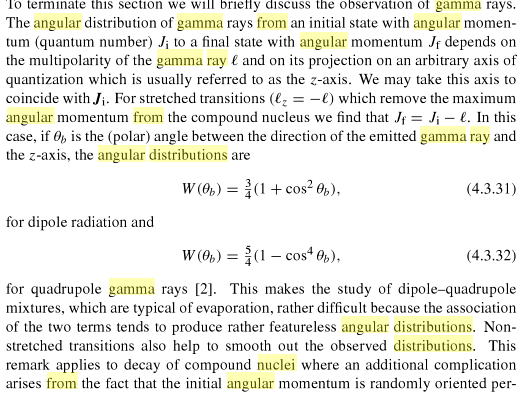
gamma orientations

Improve this answer
$\endgroup$
2
  • $\begingroup$ But are there any cases where the spatial distribution is symmetric in all directions with respect to any of abovementioned axes? Or it's symmetric for some sources only because the dipole moments have chaotic direction? $\endgroup$
    – Martino
    Commented Nov 9, 2015 at 2:17
  • $\begingroup$ If the originating nuclei have a random orientation, then the gammas will be isotropic from the radioactive sample. The distributions will be non uniform if there exists an orientation of the nuclei $\endgroup$
    – anna v
    Commented Nov 9, 2015 at 4:32
11
$\begingroup$

There are examples of nuclei whose emission is not isotropic in the presence of a magnetic field. Feynman gave an example of this during a lecture on symmetry in physical laws (vol 1-52).

In particular in 52-7 he mentions an experiment in which the emission of an electron by a cobalt nucleus (Co-60) is asymmetrical with respect to the magnetic axis - more is emitted from one pole than from the other. This was experimental proof of no conservation of parity and led to the Nobel Prize in 1957 for Lee and Yang.

You can find details in http://www.wikipedia.org/wiki/Wu_experiment - it's not exactly gamma emission but the closest example I know of...

Improve this answer
$\endgroup$
2
  • $\begingroup$ well, but that's about beta-decay which is caused by weak interaction - and we know that weak intraction violates parity. It's not a case for a gamma transition which is an electromagnetic process. $\endgroup$
    – Martino
    Commented Nov 8, 2015 at 19:37
  • $\begingroup$ @Martino you are right, and I stated so explicitly - this answer is about beta not gamma emission. having said that, the Wu experiment is the proof of this parity violation (before 1956 there were just a handful of future Nobel Prize winners who thought this would be so...) $\endgroup$
    – Floris
    Commented Nov 8, 2015 at 22:24
4
$\begingroup$

Anna and Floris have given excellent descriptions of how the photon emission depends on the orientation of the nucleus, however there is another aspect of the question that I think is worth mentioning.

If you conside an isolated nucleus, e.g. no magnetic field, then it will be in a suerposition of all possible orientations so overall the system is spherically symmetric. When the gamma ray photon is emitted it will be in a superposition of all possible directions preserving this spherical symmetry. When we interact with the photon by detecting it the superposition will collapse into a specific photon momentum state, and therefore a specific momentum state for the nucleus it came from. Since the system was originally spherically symmetric the probability of measuring the photon is the same in all directions.

If you apply a magnetic field then you break the spherical symmetry, typically down to an axial symmetry. In this case the probability of measuring the photon will no longer be spherically symmetric. As Anna and Floris have said, it will be a complicated function of the nuclear structure. However we would still expect the probability of detection to be axially symmetric.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks for your answer. So in either scenario, magnetic field on or off, the symmetry is preserved? (in the off case, it is trivial as you explained, but in the on case, the overall system before emission is no longer in a superposition of all possible states, due to the presence of the external field, or as you say, before and after emission, we have axial symmetry.) where am I going wrong in this? $\endgroup$
    – user929304
    Commented Nov 13, 2015 at 14:23
  • $\begingroup$ @user929304: with no field we have spherical symmetry with a field on we have axial symmetry. In both cases the photon is emitted ina superposition of momentum states, but the form of the superposition is different in the two cases. $\endgroup$
    – John Rennie
    Commented Nov 13, 2015 at 16:10

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.