Let's say I have a ball attached to a string and I'm spinning it above my head. If it's going fast enough, it doesn't fall. I know there's centripetal acceleration that's causing the ball to stay in a circle but this doesn't have to do with the force of gravity from what I understand. Shouldn't the object still be falling due to the force of gravity?
-
4$\begingroup$ If you know there's centripetal acceleration, then what's your question? $\endgroup$– ACuriousMind ♦Commented Nov 4, 2015 at 22:45
-
2$\begingroup$ I'm thinking of it as 2 dimensional, where centripetal acceleration is causing the ball to move toward the center of this imaginary plane in which the ball is spinning, and it's not countering the force of gravity. But then gravity adds a 3rd dimension, and it's trying to pull the ball down out from the plane. $\endgroup$– rb612Commented Nov 4, 2015 at 22:47
-
3$\begingroup$ What makes you think it doesn't fall? It simply rises at the same accelleration as it falls. Ask yourself: why doesn't the ball fall when you have it suspended from the string? $\endgroup$– LuaanCommented Nov 5, 2015 at 15:09
-
$\begingroup$ @Luaan that's right, but what we learned in class was the tension force was the centripetal force and it seemed to me like the force was completely horizonral (ie no vertical tension vector component) but these other answers explained it well. $\endgroup$– rb612Commented Nov 5, 2015 at 15:12
-
2$\begingroup$ Great job at noticing your confusion - classes often have the problem of giving authoritative answers without giving you any understanding. It should now be pretty much obvious why the general statement is only correct as long as there are no other forces involved. This isn't the case when rotating "against" gravity - now you have a balancing act between the two forces. Fortunately, two forces are still quite easy to model :) $\endgroup$– LuaanCommented Nov 5, 2015 at 15:15
4 Answers
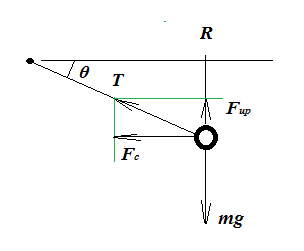
The string is at a slight angle to horizontal $\theta$. It is not exactly horizontal. The slight angle is such that the tension in the string exactly counteracts gravity, $T\sin(\theta)=m g$. So, there is actually a force acting upwards that counteracts gravity, and it is supplied by the string.
You're right that if $\theta=0$ exactly, there would be a problem and the object would necessarily fall a bit.
-
$\begingroup$ Wow! That's so cool! Thanks! So then if it's at an angle, centripetal force causing the angular acceleration (I don't know if that's the right term, the one counteracting the tangential force) is $Tcos(\theta)$? $\endgroup$– rb612Commented Nov 4, 2015 at 23:01
-
2$\begingroup$ @rb612 well, there IS no force countering the tension. There is a force $T\cos(\theta)$ in the horizontal plane towards your hand (if you hold your hand still and the thing circles around), and there are forces $T\sin(\theta)$ and $-m g$ along the vertical axis. Those are the only forces acting on the object you're spinning around. $\endgroup$– user12029Commented Nov 4, 2015 at 23:13
-
1$\begingroup$ @rb612 you could relate $T$ to $v$ by saying that the acceleration of the object, $v^2/R$, has to be caused by the acceleration due to tension, $T\cos(\theta)/m$. Then you get $mv^2/R=T\cos(\theta)$ and you could solve for the equilibrium value of $\theta$! $\endgroup$– user12029Commented Nov 4, 2015 at 23:15
-
$\begingroup$ @NeuroFuzzy It's not just "saying", that is the case, at least with classical mechanics. Leaving aside gravity, if you cut the string or release it at any point, the ball will stop accelerating (technically it will start decelerating due to air resistance if you aren't in a vacuum) and will continue travelling in (roughly) the direction its velocity was pointing when the tension was removed. In order for the ball to travel in a circle, it needs that acceleration perpendicular to the direction of motion. $\endgroup$– JABCommented Nov 5, 2015 at 21:42
We have the ball orbiting at a distance $R$ from the centre of rotation and the string inclined at angle $\theta$ with respect to the horizontal.
Two main forces act on the ball: gravity $mg$ ($m$ is the mass of the ball, $g$ the Earth's gravitational acceleration) and $F_c$, the centripetal force needed to keep the ball spinning at constant rate. $F_c$ is given by:
$$F_c=\frac{mv^2}{R},$$
where $v$ is the orbital velocity, i.e. the speed of the ball on its circular trajectory.
Trigonometry also tells us that if $T$ is the tension in the string, then:
$$T\cos\theta=F_c.$$
Similarly, as the ball is not moving in the vertical direction, thus $F_{up}$:
$$T\sin\theta=F_{up}=mg.$$
From this relation we can infer:
$$T=\frac{mg}{\sin\theta}.$$
And so:
$$\frac{mg}{\tan\theta}=F_c=\frac{mv^2}{R}.$$
Or:
$$\tan\theta=\frac{gR}{v^2}.$$
From this follows that for small $\tan\theta$ and thus small $\theta$ we need large $v$. But at lower $v$, $\theta$ increases. Also note that $\theta$ is invariant to mass $m$.
-
$\begingroup$ just as acceleration due to gravity is also invariant to mass m. $\endgroup$– OctopusCommented Nov 5, 2015 at 7:00
-
$\begingroup$ @Octopus it is important to enforce those, as this is a [homework-and-exercises] question. $\endgroup$ Commented Nov 5, 2015 at 14:05
-
1$\begingroup$ This relationship can be a useful way to mechanically measure angular velocity: en.wikipedia.org/wiki/Centrifugal_governor $\endgroup$ Commented Nov 5, 2015 at 14:05
-
3$\begingroup$ Fun corollary: It is impossible to have a perfectly straight wire between two trees. $\endgroup$– DanuCommented Nov 7, 2015 at 14:36
-
$\begingroup$ @Gert When speed increases, the angle decreases. At the same time, R in the numerator also increases. Can we relate this increase in R to the decrease in angle? I am a bit confused because, both numerator and denominator increases. $\endgroup$ Commented Dec 18, 2019 at 13:20
I appreciate that this has already been answered correctly, but I thought it may be worth adding a simplistic summary:
When the ball is spinning, there is a force acting on it which pushes it away from the centre of rotation. The only way it can get further away from that point is by moving upwards (because the string stops it from moving outwards without moving upwards). So if the force pushing the ball out is greater than the force pulling it down (gravity), it will rise.
-
$\begingroup$ There rather is a lack of force (the part of the force perpendicular to the velocity is too small to deliver the proper centripetal acceleration) which causes the ball to go away from the center (more or less a straigth line, like in Newtons First Law). The force pushing it away you are talking about is a fictional force that only exists in a reference system that moves with the ball. $\endgroup$– DriesCommented Mar 13, 2016 at 8:47
I differ with all of the explanations above. If you are spinning a ball horizontally and leave the string, it would fall at once if it is in vacuum/airless place. In other scenario which is the realistic one, it does not fall because the ball stirs the air around it away, thus creating lower air pressure zone in the plane where it is being rotated. So the air below it creates an upward pressure to hold the rotating object.
This is the theory behind how a helicopter works. By the way, have you heard of the Ninja weapon Shuriken?