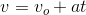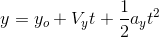1
$\begingroup$

This is a homework question. I tried to solve it, but failed.

I had to translate this question, so please forgive me if it is poorly worded.

In this question you can ignore the atmospheric effects.
A certain bird dives down 28 meters towards the sea to catch a fish. When it reaches the surface of the water, its speed is 100 km/h. However, it could never have reached 100 km/h in 28 meters, it needs a starting speed. What is the initial speed of the bird?

The acceleration is 9.8 m/s The distance is 28 m The final speed is 100 km/h, which is approximately 27.8 m/s

I figured I'd use these two formulas to figure out how much the bird accelerates in 28 m and subtract that from the final speed.

s = 1/2 * a * t^2
v = a * t

This did not work. The answer should be 15m/s. What have I done wrong?

Improve this question
$\endgroup$
0

3 Answers 3

Reset to default
2
$\begingroup$

Here are the steps you want to take. We need to find $v_0$. The equations are

$$v_t = v_0 + g\cdot t\\ y_t = y_0 + v_0 t + \frac12 g t^2$$

Two equations, two unknowns. Eliminate $t$, then solve for $v_0$

(Note that I use a Y axis that increases as you go down - just saves thinking about the sign of $g$).

Alternatively you can use conservation of energy. You have the change in potential energy, and the final kinetic energy. This allows you to calculate the initial kinetic energy. In other words

$$\frac12 m v_0^2 + m g h = \frac12 m v_f^2$$

Improve this answer
$\endgroup$
1
  • $\begingroup$ Don't know why it got downvote. However, I'm compensating it. $\endgroup$
    – user36790
    Commented Nov 4, 2015 at 16:52
1
$\begingroup$

You need initial velocity. Use these two kinematics equations.

enter image description here

enter image description here

So first find time using the second formula, then use the first formula to solve for initial velocity.

Improve this answer
$\endgroup$
2
  • $\begingroup$ What is $V_y$? Isn't it the same as $v_o$? $\endgroup$
    – Bill N
    Commented Nov 4, 2015 at 22:21
  • $\begingroup$ Sorry about that. When I split a vector into its components I put an x or y subscript in it. And yes it is the same as Vo. $\endgroup$
    – K. Ande
    Commented Nov 4, 2015 at 22:43
-1
$\begingroup$

You have three knowns, and one unknown. Pick the newtonian kinematic equation that just has the 4 variables. You don't need to use two equations.

Improve this answer
$\endgroup$
2
  • $\begingroup$ "one unknown"? I think you don't have $t$ and $v_0$. Of course if you know enough variations of kinematic equations you might have a handy single equation. I assume you do. I certainly only remember the most basic ones and derive everything else (every time...). $\endgroup$
    – Floris
    Commented Nov 3, 2015 at 21:13
  • 2
    $\begingroup$ $V_f^2 = V_o^2 + 2a \Delta S$ is all you need. $\endgroup$
    – Uys of Spades
    Commented Nov 3, 2015 at 21:15

Not the answer you're looking for? Browse other questions tagged or ask your own question.