What happens in circuits where the propagation time of the electric field is significant?

Question

Kirchhoff's current law assumes "that whenever current flows into one end of a conductor it immediately flows out the other end".

I want to understand what happens when we cannot make this assumption.

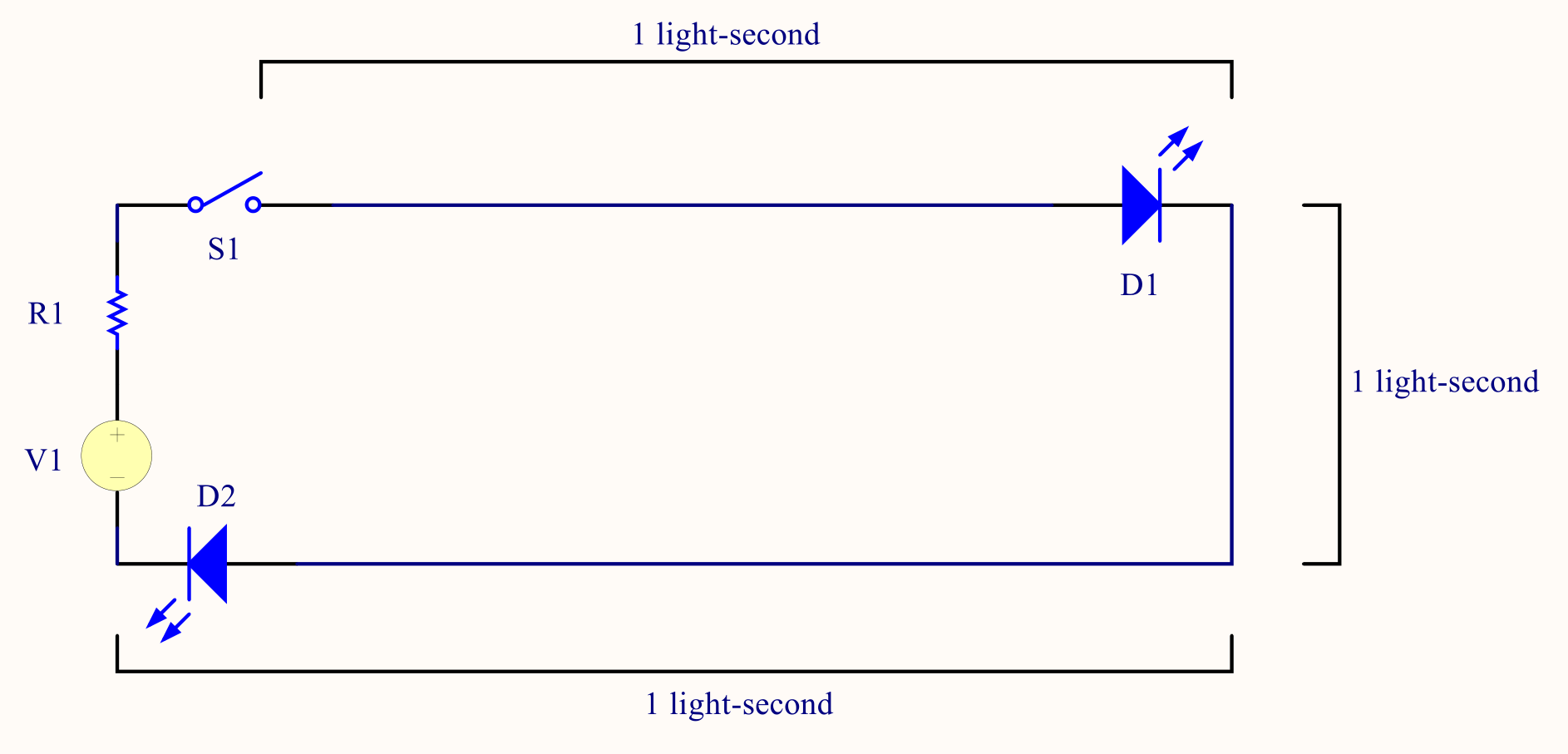
In the circuit below, imagine that $S1$ has been open (disconnected) for a long time. At a time $t = 0$ the switch is instantaneously closed. If we assume that the LEDs have instantaneous switching time, at what time $t$ will $D1$ and $D2$ emit light?

Do the LEDs begin to emit light simultaneously? Do they emit light after 3 light-seconds, 1.5 light-seconds, or some other time?

My guess is that $D1$ will emit light at $t = 1s$ and $D2$ will emit at $t = 3s$. It should be impossible for $D1$ to emit light when $t < 1s$ and for $D2$ to emit light when $t < 3s$. If this were not the case then this would result in superluminal communication, which violates causality.

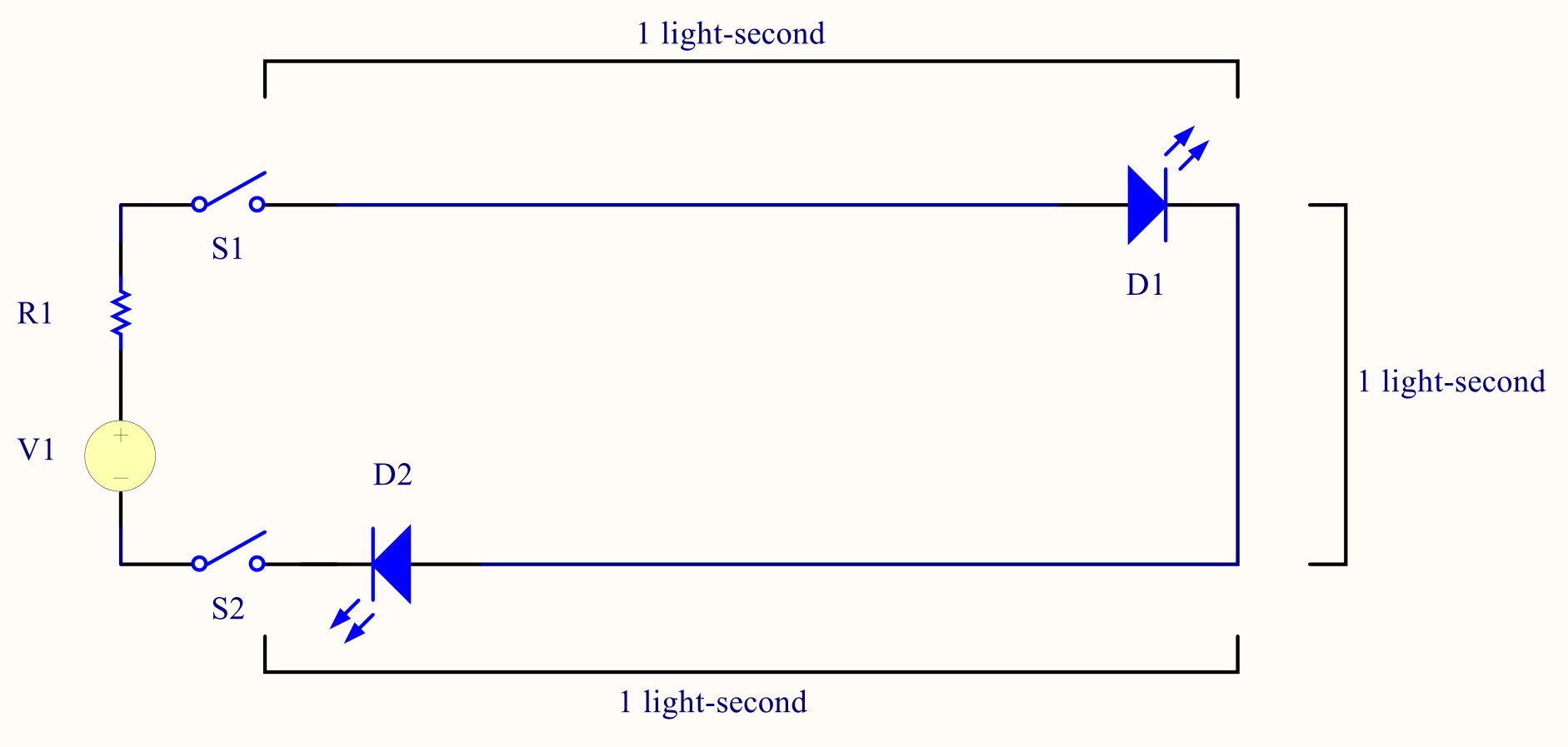
What happens when a second switch $S2$ is introduced to the circuit below? Imagine that both switches have been open for a long time. At time $t=0$ both $S1$ and $S2$ are simultaneously closed.

At what time $t$ do the LEDs emit light? What changes compared to first circuit?

Assumptions:

• The LEDs and switches are placed in close proximity to each other, although the length of the wires separating them may be very long. For example, $S1$ and $D1$ are separated by a 1 light-second length of wire, however, we assume that they are physically placed close together relative to a human observer looking at the circuit. Only the electrical paths have significant propagation times.
• The electric field propagates through the circuit at c
• All circuit elements are ideal
• Switches and LEDs have instantaneous switching time
• When a switch is open, it is physically disconnected from the circuit.
• There are no parasitic resistances, capacitances, or inductances

Answer 1

Any wire circuit will have inductance and capacitance between the "outbound" and "return" wires - this immediately follows from very basic laws of physics, and in fact is intimately related to the finite propagation velocity of the electrical signal. The expression

$$u=\frac{1}{\sqrt{LC}}$$

would give an infinite velocity if either $L$ or $C$ was zero... So I am not going to answer the non-physical question "in the absence of parasitic inductance and capacitance".

This relationship is usually explained in terms of the Telegrapher's Equations, which show that a transmission line will have a characteristic impedance, and that a signal travels along such transmission line with a velocity related to the speed of light in the medium between the conductors. If the conductors are in vacuum, the speed of propagation is the speed of light.

We cannot answer your question and ignore this. But if we do take it into account, we can analyze the voltage pulse that travels along the wire - and the current that it generates.

We need to know the characteristic impedance of the wire. Again, the Telegrapher's Equations come to our help, and we find that

$$Z = \sqrt{\frac{L}{C}}$$

Thus, if we apply a voltage $V$ at the start of the transmission line, there will be a current $I = \frac{V}{Z+R}$ that starts to flow into the circuit immediately. An LED that is close to the voltage source will light to the extent that this current is sufficient to light it. Note that $Z+R$ is always greater than $R$, so the current is initially less than the steady state current.

Once the voltage pulse reaches the far end of the circuit, it will be reflected by the "short circuit" that is present there; and this results in the circuit finally "knowing" it is part of a circuit. The reflected pulse (inverted voltage) will take another second to travel back.

The presence of the LEDs with their finite impedance makes the calculation of the actual intensity of the emissions quite complex, as they result in partial reflections: consequently there will be multiple reflections back and forth before the steady state is reached. But the bottom line is:

1. D2 will light 1 second before D1
2. The answer is unchanged when you introduce the second switch
3. In either case, the final intensity is not reached until several seconds after closing the switch(es)

Answer 2

What happens in circuits where the propagation time of the electric field is significant?

The answer to your question is given in the article: Telegrapher's equations.

"The telegrapher's equations (or just telegraph equations) are a pair of coupled, linear differential equations that describe the voltage and current on an electrical transmission line with distance and time. The equations come from Oliver Heaviside who in the 1880s developed the transmission line model, which is described in this article. The model demonstrates that the electromagnetic waves can be reflected on the wire, and that wave patterns can appear along the line. The theory applies to transmission lines of all frequencies including high-frequency transmission lines (such as telegraph wires and radio frequency conductors), audio frequency (such as telephone lines), low frequency (such as power lines) and direct current.", Source.