How to find speed from Newton's law?

Question

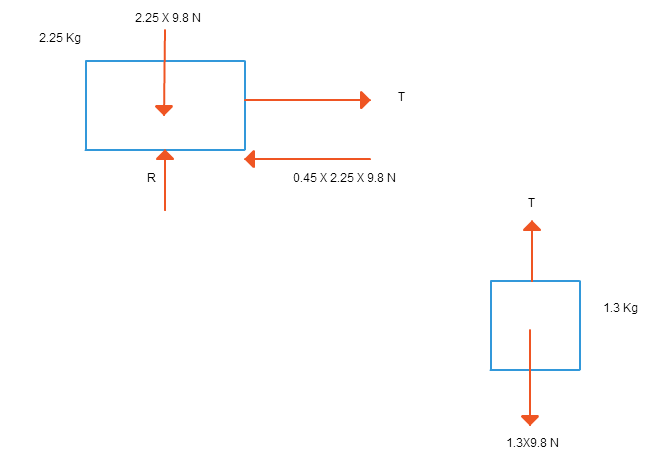
I have a question about Newton's law. The question says

block A(mass 2.25kg) rests on a tabletop. It is connected by a horizontal cord passing over a light, friction less pulley to hanging block B(mass 1.30kg). The coefficient of kinetic friction between block A and the tabletop is 0.450. After the blocks are released from rest, find (a) the speed of each block after moving 3.00 cm and (b) the tension in the cord. Include the free-body diagram or diagrams you used to determine the answer.

I tried to solve using following formula

$$f_{k} = \mu_{k}N$$

$$T-w_{B} = m_{b}a$$

$$T = m_{A}a$$

First time, I tried to get $a$ Since

$$f_{k}=\mu_{k}N$$

$$m_{A}a = \mu_{k}N$$ $$m_{A}a = \mu_{k}m_{A}g$$ From given $m_{A} = 0.45, \delta x=0.03(m), m_{A}=2.25$ I cancelled out $m_{A}$ both side then I got $$a = \mu_{k}g$$ which is $$a = 0.45*9.8 \implies a = 4.41\ \mathrm{m/s^2}$$

Part a asked for its speed so I used $\delta x = \frac{V^2-V_{0}^2}{2a}$

I got $V = 0.897\text{ m/s}$ but the speed I got was not right speed. So I think I made some mistake at some point. What did I do wrong?

Answer 1

Your $ T=m_Aa $ is wrong.

It's $ T - f= m_Aa $.

Because Tension due to $ m_B $ is pulling it forward and friction is trying to resist that force.

Using $v^2 = u^2 + 2as$ for calculating velocity after moving $ s = 0.03 m $ forward with initial velocity $ u = 0 m/s $ is correct!.

Answer 2

The complete analysis and solution for this problem is as given below.

STEP 1: Draw the free-body diagram of each mass, showing all forces acting upon each mass. Assume the normal reaction force from table on the mass of 2.25 kg is R Newtons, and the tension in the string connecting the masses is T Newtons.

STEP 2: Write down the equations for each mass using Newton's second law of motion. Write these equations for vertical and horizontal directions for each mass. We assume that mass on table moves to the right due to the hanging mass pulling it down and also assume that the acceleration of each mass is a $m/s^2$.

For mass on table, following are equations of motion in horizontal and vertical directions. $$T - (0.45 X 2.25 X 9.8) = 3.25a$$ $$R = 2.25 X 9.5$$

For hanging mass, following is the equation in vertical direction. There are no horizontal forces on this mass and so we only have an equation in vertical direction. $$(1.3 X 9.8) - T = 1.3a$$

STEP 3: Solve the equations to get the variable(s) needed. In this case we will solve for a and T. We get a = 0.619 $m/s^2$ and

$T$ = $11.935 N$

.

STEP 4: Use the following equation of motion: $v^2=u^2+2as$

$v^2=(0)^2+2(0.619)(.03)$

Solving above equation gives us:

$v = 0.19275$ $ m/s$

as the velocity of each mass after the mass on table top has moved by 3 $cm$.