What is the Al'tshuler-Aronov-Spivak effect?

Question

1. What is the Al'tshuler-Aronov-Spivak effect (AAS)?

2. How does it relate to the Aharonov-Bohm effect (AB)?

Answer 1

The Al'tshuler-Aronov-Spivak effect is an oscillation in the conductivity of a topologically doubly-connected conductor as one increases the magnetic flux it encloses. It is very similar - and intimately related - to the Aharonov-Bohm effect, but it differs from it in that

1. the oscillations are twice as fast, because the relevant loops are twice as long, and

2. it is more sturdy with respect to noise, and it is still present even when the electrons scatter multiple times along the loop.

In contrast, Aharonov-Bohm oscillations in conductivity generally require clean samples with no impurities that will scatter the charge carriers.

The original proposal,

The Aaronov-Bohm effect in disordered conductors. B. L. Al'tshuler, A. G. Aronov and B. Z. Spivak, Pis'ma Zh. Eksp. Teor. Fiz. 33, 101 (1981) [JETP Lett. 33 no. 2, 94 (1981)].

is relatively terse, but it's there.

A typical experimental setup is a long cylindrical conductor with leads on the ends, and one tries to measure the cylinder's resistance along its length. Other experiments use rings and measure transmission or reflection coefficients from them.

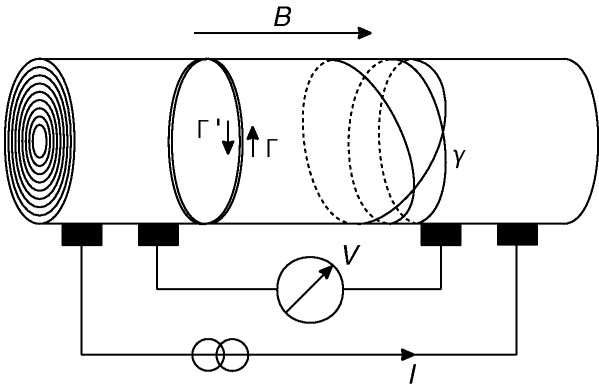
^{Image source: Aharonov–Bohm oscillations in carbon nanotubes. Bachtold et al., Nature 397, 673 (1999).}

In propagating along such a cylinder, there are multiple trajectories which loop around the cylinder and then join up at the other lead. Because of the Aharonov-Bohm effect, such trajectories will accumulate a phase that is proportional to the magnetic flux inside the cylinder. This means that by changing the flux inside the cylinder we can change the conditions of the interference between the different trajectories, and turn them from constructive to destructive and vice versa.

However, in calculating this interference it is important to know whether the different paths simply go around the cylinder on different sides and then meet up on the other end, or whether they go all the way around the cylinder in different directions and meet up on the same side they started.

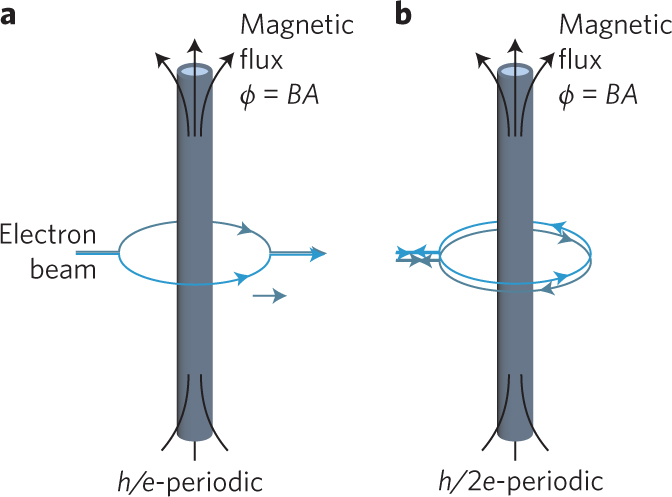
^{Image source: Topological insulators: Oscillations in the ribbons. T. Ihn, Nature Materials 9, 187 (2010)}

It is fairly clear that in the second case the flux enclosed by the path difference is twice as in the first case, so the flux dependence will be twice as fast. This is the Al'tshuler-Aronov-Spivak effect, while the first case is the standard Aharonov-Bohm interference.

Depending on the experimental conditions, either or both can be present. The AAS effect is present when the system exhibits a significant amount of disorder, i.e. when the mean-free-path of the electron among the material's impurities is of the order of the sample size or smaller. (On the other hand, the phase-diffusion mean free path must still be longer than the sample size.)

The standard Aharonov-Bohm oscillations, on the other hand, are destroyed by disorder, as explained by Ihn*:

The reason why in these experiments the h/e-periodicity was not observed was explained by various studies on metals¹⁰ and semiconductors⁶, for example on ring-shaped planar geometries and arrays of rings. Briefly, pairs of paths contributing to the fundamental h/e period have a specific relative phase at zero magnetic field. If many such pairs with uncorrelated zero-field phases contribute to transport, the h/e oscillations average out. In contrast, the h/2e-periodic oscillations contain a significant contribution of time-reversed paths, which all have the same relative phase of zero at the interference point, and are therefore robust against averaging.

Ihn's reference,

Aharonov-Bohm effect in normal metal quantum coherence and transport. S. Washburn and R.A. Webba. Adv. Phys. 35 no. 4, 375 (1986). Eprint, Researchgate.

gives a more in-depth analysis of these differences.

^{* German speakers will, I hope, appreciate the pun.}