2
$\begingroup$

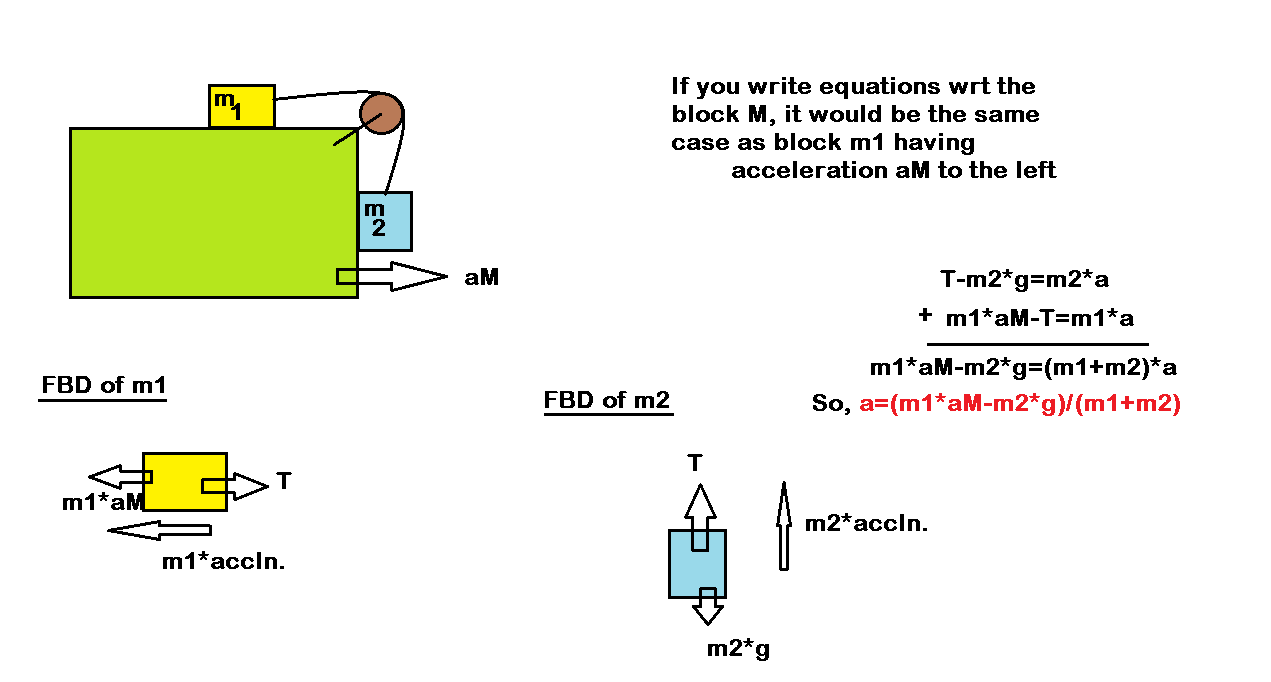
This is a follow-up to my previous question, in which I am now trying to calculate the acceleration of the cart (as before, the block surfaces are frictionless). The mass $m_2$ is attached to $M$ via a frictionless track that keeps it fixed onto the side of $M$ but allows it to move vertically with respect to $M$.

enter image description here

To do this, I first need to find the tension on the string.

I came up with the system of equations:

$$T=m_{1}\left( a-a_{M} \right)$$ $$T-m_{2}\text{g}=-m_{2}a$$

Where the acceleration of $m_1$ is $a - a_M$ (where $a$ is the magnitude of the acceleration of $m_2$) since $ m_1 $ moves right while $M$ moves left. However, is this the right way to approach it — would the accelerations even cancel each other since the surface is frictionless and the movement of $M$ cannot "pull" $m_1$ along?

Also, since the tension created is responsible for the acceleration of the $M + m_2$ system, isn't this equation also valid?: $$T = a_M\left(M + m_2 \right)$$

Clearly the solutions to both are not the same, so one (or possible both) of the above are incorrectly accounting for the forces acting on the mass.

Furthermore, once this tension is found how do you account for the normal force between $M$ and $m_2$ that also affects the acceleration?

Alternatively, is it possible to solve this using conservation of momentum or by using center of mass?

Improve this question
$\endgroup$
6
  • $\begingroup$ When you do a plain half Atwood's machine the pulley is held at rest. In this system it (and the cart) accelerate. Follow the thinking there and you'll answer you're own question. (BTW self-answers are encouraged.) $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Oct 4, 2015 at 21:11
  • $\begingroup$ @dmckee So is it then correct to say that the tension on both halves of the string are not the same, due to the effect of the cart's acceleration? $\endgroup$
    – 1110101001
    Commented Oct 4, 2015 at 21:55
  • $\begingroup$ As before, the answer depends on whether mass $m_2$ is free to swing away from the side of the cart. $\endgroup$
    – Daniel Griscom
    Commented Oct 4, 2015 at 23:26
  • $\begingroup$ @DanielGriscom I believe the mass $m_2$ is attached via a railing that keeps it pinned to $M$ and only allows it to move up/down $\endgroup$
    – 1110101001
    Commented Oct 4, 2015 at 23:49
  • 1
    $\begingroup$ If the mass was free to swing the answer becomes very complicated as the relationship between tension on the sting, acceleration on $m_2$ and the acceleration of the string across the pulley depends on the position and velocity of $m_2$ relative to the pulley. so the problem becomes a system of second order partial differential equations with 4 variables, which would be equivalent to a seventh order differential equation, which would have to be solved numerically. $\endgroup$
    – Eph
    Commented Oct 13, 2015 at 12:32

2 Answers 2

Reset to default
1
$\begingroup$

Your equations for tension are all correct. You have three equations and three unknowns $T$, $a$, and $a_M$ so you can solve for that system.

If you'd like to know the normal force between $M$ and $m_2$ you can calculate that as:

$$N=m_2\,a_M$$

Improve this answer
$\endgroup$
0
0
+50
$\begingroup$

Actually, due to lack of friction, you could say $m_1$ would remain right there and $M$ would move underneath it. But, you could say it the other way round. $M$ is still and $m_1$ moves to the left with acceleration $\frac{m_1a_M-T}{m_1}$. Now, $m_2$ would have an acceleration of $\frac{T-m_2g}{m_2}$. Now, both these accelerations are same. Solve for acceleration by adding the 2 equations (this will eliminate $T$)enter image description here

Improve this answer
$\endgroup$
5
  • $\begingroup$ Ok that makes sense. As a followup, would the free body diagram and force expressions change if $m_2$ were no longer kept pinned to the side of $M$? Furthermore, is it possible to solve this by considering that the center of mass of the system must remain constant? $\endgroup$
    – 1110101001
    Commented Oct 11, 2015 at 4:28
  • $\begingroup$ No, it doesn't matter if you fix m2 because the block M is moving towards m2. But if there is friction, answer would definitely change. Also, COM would not remain the same because the net external force on the system (3 blocks + pulley) is non zero (there is gravitational force acting which causes acceleration). It would only make things complicated if you write equations for COM. Try to use force equations as much as possible. If you want help writing COM equations, post a new question and I will answer it. $\endgroup$
    – ShankRam
    Commented Oct 11, 2015 at 11:52
  • $\begingroup$ So, you're working in the accelerating reference frame of $M$, but then you don't specify how to solve for $a_M$. Block $M$ is accelerating away from $m_2$ so the answer would change if the mass was no longer pinned. The COM does remain stationary as the net force is zero because the normal force cancels with gravity. $\endgroup$
    – Eph
    Commented Oct 13, 2015 at 12:16
  • $\begingroup$ Rick, how does the gravitational force on m2 cancel? There is no normal force in this direction. The net force will be zero if you take earth also in the system $\endgroup$
    – ShankRam
    Commented Oct 14, 2015 at 5:54
  • $\begingroup$ Oh, good point, the COM will move in the vertical direction, but keeping the COM stationary in the horizontal direction would be another way to solve it. I would consider it simpler than using an accelerating reverence frame, but maybe that's just me. $\endgroup$
    – Eph
    Commented Oct 19, 2015 at 14:27

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.