My textbook has clearly explained and derived a velocity that is required for a car to navigate a turn on a frictionless banked surface. I have understood it too. My doubt is about what happens when the cars velocity exceeds or falls short of this required velocity. Someone told me that it will veer towards the outside of the curve when the velocity exceeds and will veer towards the inside when the velocity is less. I'm just not able to wrap my head around this. I tried proving it mathematically but I failed everytime.
$\begingroup$
$\endgroup$
5
-
$\begingroup$ Try drawing a free body diagram with all the forces acting on the car. $\endgroup$– GertCommented Sep 29, 2015 at 14:31
-
$\begingroup$ @Gert I did. But since the opposing forces are not equal like they would be if the car was as the exact required velocity, I'm not able to move further. $\endgroup$– MayankJainCommented Sep 29, 2015 at 15:09
-
$\begingroup$ The difference between the force is $ma$, with $a$ the acceleration with which the car will sliding 'up' the slope or 'down' the slope. The sign of $a$ tells you 'up' or 'down'. $\endgroup$– GertCommented Sep 29, 2015 at 15:14
-
$\begingroup$ @Gert I get this and I tried using this but was unable to get to the answer. Could you explain this in a little more detail as an answer? It would be highly appreciated. $\endgroup$– MayankJainCommented Sep 29, 2015 at 15:39
-
$\begingroup$ See my answer below. $\endgroup$– GertCommented Sep 29, 2015 at 16:26
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
1
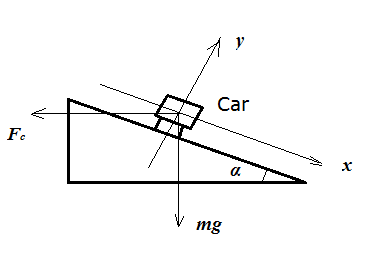
Place the car's centre of gravity at the origin of a Cartesian coordinate system $x,y$, as shown above.
Now determine the balance of forces along the $x$ axis. Assume the car will slide (up or down), as there's no friction. The equation of motion is:
$ma_x=mg\sin\alpha-F_c\cos\alpha$ (Eq.1).
$F_c=\frac{mv^2}{R}$ with $v$ the velocity of the car and $R$ the radius of the turn. Insert into Eq.1, drop $m$ and solve for $a_x$:
$a_x=g\sin\alpha-\frac{v^2}{R}\cos\alpha$.
If $g\sin\alpha>\frac{v^2}{R}\cos\alpha$, then $a_x>0$ and the car will sliding 'down' (acceleration in the $x$ direction).
If $g\sin\alpha<\frac{v^2}{R}\cos\alpha$, then $a_x<0$ and the car will sliding 'up' (acceleration in the opposite of the $x$ direction).
Note that this is a very unrealistic case: a road that offers no friction at all.
-
$\begingroup$ Thanks for the great answer! Much clearer now. I have just one question. The force Fc here is centrifugal force right? $\endgroup$ Commented Sep 30, 2015 at 3:59