To my knowledge it is not forbidden by the standard model laws. The point is that at low energy scale this process is strongly subleading with respect to other decay's channels, for instance to an electromagnetic decay in two photons.
At high energy the strength of the gravitational force grows and that process could be one of the main channels of decay.
To be more precise we need a theory of quantum gravity in which we can do calculations. Actually we already have such a theory, is string theory.
In string theory this is a very simple scattering amplitude (at least at tree level) and in particular this is a mixed open-closed string amplitudes.
The open strings lives on a Dp-brane and join to emit a closed string. This is the stringy realization of the Hawking radiation (the time reversal of the picture on the left).
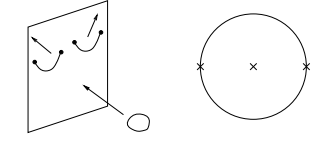
On the right of the image you can see the process from the viewpoint of the worldsheet. Closed strings vertex operators must be inserted in the interior of the disk (the disk is the worldsheet's topology that in this case gives the leading contribution in a perturbative $g_s$ string coupling expansion), while open vertex operators are inserted on the boundary.
More explicitly the amplitude is something like:
$$A=\int dx <V_F(x) V_{NS-NS}(z=i,\bar{z}=-i) V_F(y=-x)>$$
In which $V_F$ and $V_{NS-NS}$ are respectively the open vertex operators for fermions and closed vertex for Neveu-Schwarz bosons (so for instance gravitons). The explicit form of the vertex operators I think is too technical and it doesn't add much to the discussion.
(A clarification: particles like electrons of the standard model emerge in string theory from complicated intersecting brane models. This is very simplified situation, and these fermions are the more exotic gravitini, dilatini or gaugini, that are superpartners respectively of gravitons, dilaton and gauge bosons.)
The picture is taken from:
Scattering of strings from D-branes,
(Akikazu Hashimoto, Igor R. Klebanov),
Mar 1996, hep-th/9611214

