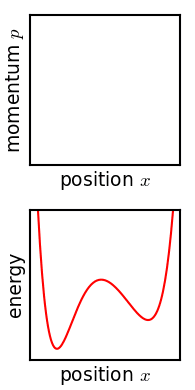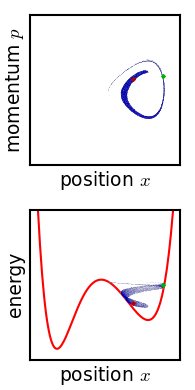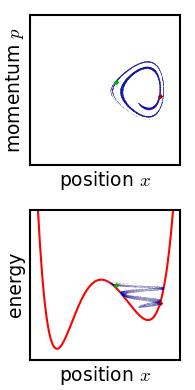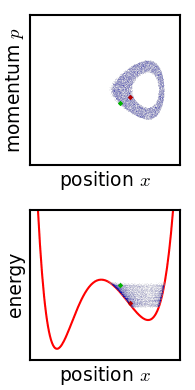
I think the intended question was about a thermally isolated system, so no heat could be exchanged; work exchange is allowed.
What's wrong with the reasoning here?
In short, the statistical entropy (=statistical physics concept of thermodynamic entropy) that is defined for any equilibrium state and can increase in thermally isolated system is not the same thing as the information entropy (=a functional of the probability density, or phase space volume where the probability density is the same everywhere) that remains constant due to the Liouville theorem. These two entropies are related, but they are not the same concept nor do they always have the same value. When in non-equilibrium process the system comes to a new equilibrium, the statistical entropy has increased, while the information entropy has remained constant.
1)
The second law says that entropy can only increase
More accurately, it says that when state is changed from equilibrium macrostate $A$ to equilibrium macrostate $B$, thermodynamic entropy cannot decrease. Equilibrium macrostate can be specified by stating values of sufficient number of macroscopic variables $X_1,X_2,...$ (we can denote them together as a tuple $\mathbf X$). (For example, equilibrium state of ideal gas in a closed vessel is specified by giving values of volume $V$ and internal energy $U$.) Then thermodynamic entropy function $S(\mathbf X)$ of these state variables can be introduced.
With this, the consequence of 2nd law for adiabatic systems - non-decrease of thermodynamic entropy - can now be stated in this way:
$$
S(\mathbf X_A) \leq S(\mathbf X_B).
$$
2)
entropy is proportional to phase space volume.
Here we get into statistical entropy, a statistical physics concept of thermodynamic entropy. Statistical entropy of the equilibrium macrostate $\mathbf X$ is proportional to logarithm of the volume of the phase space region defined by all those points that are compatible with the macrostate $\mathbf X$. Let us denote volume of this region by $\Omega(\mathbf X)$. Statistical entropy of macrostate $\mathbf X$ is defined as
$$
S^{(stat)}(\mathbf X) = k_B \ln \Omega(\mathbf X).
$$
Thus statistical entropy is a function of the macrostate $\mathbf X$. If macrostate changes, $\Omega$ may change, and if so, then so does $S^{(stat)}$.
Statistical entropy changes are (in the so-called thermodynamic limit) supposed to be the same as changes of thermodynamic entropy of the system modeled - otherwise something in the statistical model is wrong.
3)
Liouville's theorem says that phase space volume is constant
[...in time].
Yes, but "volume" in Liouville's theorem is something different. Consider, at time $t_1$, a set of representative points in phase space that are all compatible with the macrostate $\mathbf X_A$. Let the volume of this set be denoted $\Delta \omega(t_1)$, and of course, we have $\Delta \omega(t_1) = \Omega(\mathbf X_A)$.
As time goes on, these points move along their phase trajectories, but volume of the set of all points remains constant, at least as long as the set is "nice enough" (measurable). Thus the function $\Delta \omega(t)$ is a constant function.
If this set of moving representative points comes, at some time $t_2$, to positions that are all consistent with the macrostate $\mathbf X_B$, we have a kind of description of evolution of the macrostate $\mathbf X_A$ to the macrostate $\mathbf X_B$ (an ensemble of imagined systems that move from one macrostate to another). The set of representative points moved from being in the region $A$ to being in the region $B$. But this does not mean that the set of moving points became the same as the set of all points in region $B$; at time $t_2$, there may be "holes" in $B$, not occupied by any representative points. Motion of the representative points is described by "all points of the region $A$ come into the region $B$", not by "all points of the region $A$ move onto all points of the region $B$". Mathematically speaking, the mapping of the phase region $A$ from time $t_1$ to $t_2$ is into $B$ (it is an injection), not onto $B$ (it is not a surjection). So $\Delta \omega (t_2)$ is not volume of the same set as the volume $\Omega(\mathbf X_B)$ is. $\Delta \omega(t_2)$ is usually smaller.
The volume that is sometimes used to formulate the Liouville theorem is, using a here-made up notation, volume of the set $M(L_t A)$ - set of phase points that are reached by evolution of the original set $A$ in time $t$. At time $t_2$, this is a different set than the set $M(B)$ of all microstates compatible with the macrostate $\mathbf X_B$, even if $M(L_{t_2}A)$ is a subset of $M(B)$.
The big "If" above is an assumption about behaviour of the set of representative points chosen. But in mechanics, if we check all points in region A, then in general, not all will end up in B. Some of them may go to completely different regions of phase space, e.g. regions describing macrostates of lower phase volume and lower statistical entropy. So the Liouville theorem does not imply either increase or decrease of statistical entropy, but it is compatible with both. And it is a statement about phase volume of a different set from that referred to in statistical entropy.
In other words, the actual problem many people have with understanding how 2nd law is compatible with the Liouville theorem is that they mistakenly think the formula for statistical entropy is
$$
k_B\ln \Delta\omega(t).
$$
But this is not so. The latter expression is for a different kind of entropy, a special case of information entropy, or the Gibbs entropy, which is not a function of the macrostate, but a functional of the probability distribution:
$$
S^{Gibbs}[\rho] = k_B\int -\rho \ln \rho ~ dqdp .
$$
When $\rho$ is $\frac{1}{\Delta \omega}$ inside the region made up of the moving points, and 0 outside, we have
$$
S^{Gibbs}[\rho] = k_B \int -\frac{1}{\Delta \omega} \ln \frac{1}{\Delta \omega}~ dqdp = k_B \ln \Delta \omega.
$$
which is constant in time.
In a scenario where the Liouville theorem applies, information/Gibbs entropy remains constant in time, while statistical entropy, being a function of macrostate $\mathbf X$, may change in time.