How is this circuit equivalent to a Bell measurement?

Question

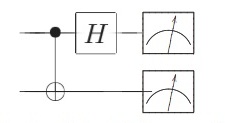
The circuit elements are :

1. CNOT gate, first qubit is the control bit
2. Hadamard gate (H)
3. The dials represent measurements made in the computational basis

Therefore, the circuit (without the measurements) is equivalent to $\text{CNOT}\;(H \otimes I)$.

My question is, how is this circuit equivalent to a Bell measurement?

In this paper (pages 14-15) the author mentions that the initial part of this circuit (without the measurements) is also equivalent to a change of basis from the computational basis to the Bell basis.

But at the same time, Nielsen and Chuang mention in their book (Quantum Computation and Information, Page 188) that the initial part is a change of basis from the Bell basis to the computational basis!! Which one is true and why is it true?

Edit :- By comparing the matrix of the net operation $\text{CNOT}\;(H \otimes I)$ and the actual change of basis matrix (from Bell to computational), as given in the paper, I could figure out that the circuit does represent a change of basis from the computational basis into the Bell basis. But why should both be true?

Answer 1

The reverse of the circuit in your picture prepares the Bell states:
\begin{align} C_X (H\otimes I) |00\rangle &=(|00\rangle +|11\rangle)/\sqrt{2}\\ C_X (H\otimes I) |01\rangle &=(|01\rangle +|10\rangle)/\sqrt{2}\\ C_X (H\otimes I) |10\rangle &=(|00\rangle -|11\rangle)/\sqrt{2}\\ C_X (H\otimes I) |11\rangle &=(|01\rangle -|10\rangle)/\sqrt{2} \end{align} (The order of $C_X$ and $H\otimes I$ in the picture is correct.)

Just multiply all equations with $(H\otimes I)C_X$ from the left to get \begin{align} |00\rangle &=(H\otimes I)C_X (|00\rangle +|11\rangle)/\sqrt{2}\\ |01\rangle &=(H\otimes I)C_X (|01\rangle +|10\rangle)/\sqrt{2}\\ |10\rangle &=(H\otimes I)C_X (|00\rangle -|11\rangle)/\sqrt{2}\\ |11\rangle &=(H\otimes I)C_X (|01\rangle -|10\rangle)/\sqrt{2} \end{align} and these equations describe how your measurement works: read it from right to left: the incoming Bell states are processed by the controlled-Not gate $C_X$ and then $H$ acts on the first qubit. The final state is measured in the computational basis.

Answer 2

Your circuit $\text{CNOT} (H \otimes I)$ transforms the Bell basis to the calculation basis, and the meters measure in the calculation basis, also known as the z basis. Hardware is set up to measure in the z basis, so to measure which Bell state the two-qubit state is in (probabilistically, if the state is a superposition of Bell states), we transform the Bell states to the z eigenstates, measure, and deduce which Bell state the two qubits were in. This is called a Bell measurement.

To leave the state in the Bell state that was deduced, the way you normally think of measurement doing, you could apply $(H \otimes I)\text{CNOT}$ after the measurement, but cannot defer the measurement. This is a Bell filter, and a circuit that works for that is given in https://arxiv.org/abs/1612.08578. That circuit is designed to work even when the two qubits are in different locations, making a CNOT impossible to implement locally, but requires consumption of two ebits. (An ebit being the entanglement of a Bell pair.)